What is the ratio between the distance travelled by the oscillator in one time period and amplitude?
To find the ratio between the distance traveled by the oscillator in one time period and its amplitude, we can follow these steps:
Step 1: Understand the Motion of the Oscillator
An oscillator moves back and forth between two extreme positions. The maximum displacement from the mean position is called the amplitude (A).
Step 2: Determine the Distance Traveled in One Time Period
In one complete time period (T), the oscillator moves from one extreme position to the other and back. The motion can be broken down as follows:
- The oscillator moves from the mean position to the maximum positive amplitude (A).
- Then, it moves back to the mean position (0).
- Next, it moves to the maximum negative amplitude (-A).
- Finally, it returns back to the mean position (0).
Step 3: Calculate the Total Distance Traveled
The total distance traveled during one complete cycle (one time period) can be calculated as:
- From 0 to A: distance = A
- From A to 0: distance = A
- From 0 to -A: distance = A
- From -A to 0: distance = A
Adding these distances together:
Total Distance=A+A+A+A=4A
Step 4: Calculate the Ratio
Now, we need to find the ratio of the total distance traveled to the amplitude:
Ratio=Total DistanceAmplitude=4AA=4
Conclusion
Thus, the ratio between the distance traveled by the oscillator in one time period and its amplitude is 4.
---
An oscillator moves back and forth between two extreme positions. The maximum displacement from the mean position is called the amplitude (A).
In one complete time period (T), the oscillator moves from one extreme position to the other and back. The motion can be broken down as follows:
- The oscillator moves from the mean position to the maximum positive amplitude (A).
- Then, it moves back to the mean position (0).
- Next, it moves to the maximum negative amplitude (-A).
- Finally, it returns back to the mean position (0).
The total distance traveled during one complete cycle (one time period) can be calculated as:
- From 0 to A: distance = A
- From A to 0: distance = A
- From 0 to -A: distance = A
- From -A to 0: distance = A
Total Distance=A+A+A+A=4A
Now, we need to find the ratio of the total distance traveled to the amplitude:
Ratio=Total DistanceAmplitude=4AA=4
Thus, the ratio between the distance traveled by the oscillator in one time period and its amplitude is 4.
Topper's Solved these Questions
- MOTION IN A STRAIGHT LINE BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - MOTION IN A STRAIGHT LINE EXERCISE - Very Short Answer Type Qns
14 Videos
- SYSTEM OF PARTICLES AND ROTATIONAL MOTION BOOK - NCERT EXEMPLAR ENGLISHCHAPTER - SYSTEM OF PARTICLES AND ROTATIONAL MOTION EXERCISE - Long answer type questions
12 Videos
Similar Questions
Knowledge Check
A particle executing simple harmonic motion with an amplitude A. The distance travelled by the particle in one time period is
AzeroBAC2AD4A
What is the relation between time period (T) and frequency (f) of an oscillation of a simple pendulum?
View SolutionThe distance moved by a particle in simple harmonic motion in one time period is
View SolutionIf the molecules of SO2 effuse a distance of 150cm in a certain period of time, the distance travelled by the molecules of CH4 effusing in the same time is
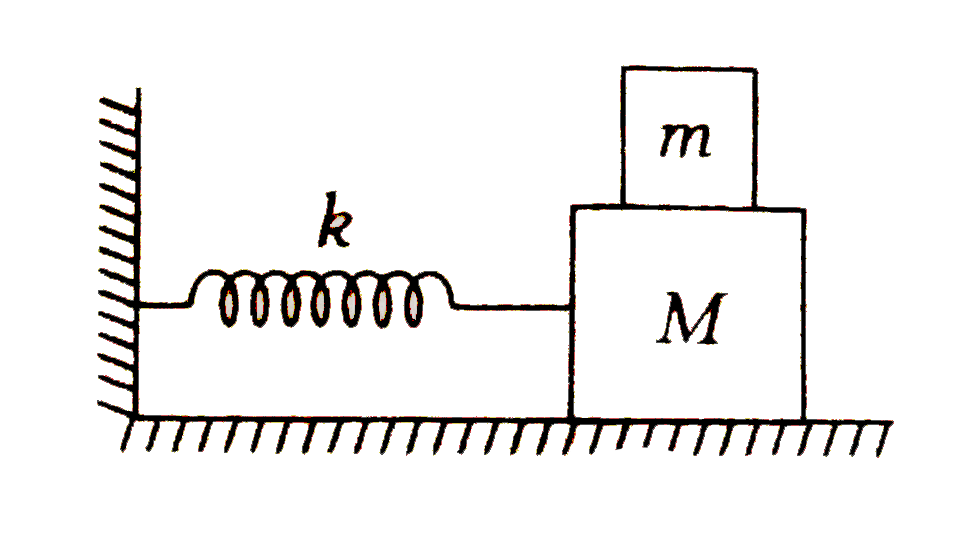
View SolutionA mass M is attached to a horizontal spring of force constant k fixed one side to a rigid support as shown in figure. The mass oscillates on a frictionless surface with time period T and amplitude A. When the mass M is in equilibrium position, another mass m is gently placed on it. When will be the new amplitude of ocillation?
View SolutionWhat are wavelength, frequency, time period and amplitude of a sound wave ?
View SolutionA simple pendulum has some time period T. What will be the percentage change in its time period if its amplitude is decreased by 5%
View SolutionThe equation of a travelling plane sound wave has the form y=60cos(1800t−5.3x), where y is in micrometres, t in seconds and x in metres. Find
(a). The ratio of the displacement amplitude with which the particle of the medium oscillate to the wavelength,
(b).the velocity oscillation ammplitude of particles of the medium and its ratio to the wave propagation velocity , (c ).the oscillation amplitude of relative deformation of the medium and its relation to the velocity oscillation amplitude of particles of the medium, (d). the particle acceleration amplitude.
View Solution