A wire AB is carrying a steady current of 12 A and is lying on the table. Another wire CD carrying 5 A is held directly above AB at a height of 1mm. Find the mass per unit length of the wire CD so that it remains suspended at its position when left free. Give the direction of the current flowing in CD with respect to that in AB. [Take the value of g=10ms−2]
To solve the problem step by step, we need to analyze the forces acting on wire CD and how they relate to the current in wire AB.
Step 1: Identify the forces acting on wire CD
Wire CD is held above wire AB and carries a current. The forces acting on wire CD are:
- The gravitational force (weight) acting downward, Fg=mg
- The magnetic force due to the interaction with wire AB, acting upward.
Step 2: Set up the equilibrium condition
For wire CD to remain suspended, the magnetic force must balance the weight of the wire. Therefore, we have:
Fm=Fg
Where:
- Fm is the magnetic force acting on wire CD.
- Fg=mg is the weight of wire CD.
Step 3: Calculate the magnetic force between the two wires
The magnetic force per unit length between two parallel wires carrying currents I1 and I2 separated by a distance d is given by:
Fm=μ0I1I22πd⋅L
Where:
- μ0=4π×10−7T m/A (permeability of free space)
- I1=12A (current in wire AB)
- I2=5A (current in wire CD)
- d=1mm=1×10−3m
Step 4: Relate the mass per unit length to the forces
The mass per unit length of wire CD is denoted as λ. The weight of wire CD can be expressed as:
Fg=λg
Substituting this into the equilibrium condition gives:
μ0I1I22πd⋅L=λg
Step 5: Solve for mass per unit length λ
Rearranging the equation, we have:
λ=μ0I1I22πdg
Step 6: Substitute the known values
Substituting the known values into the equation:
λ=(4π×10−7)×12×52π×(1×10−3)×10
Simplifying this:
- The π cancels out:
λ=4×10−7×12×52×(1×10−3)×10
- Calculating the numerator:
4×12×5=240
- The denominator:
2×10−3×10=2×10−2
Thus:
λ=240×10−72×10−2=240×10−72×10−2=120×10−5=1.2×10−3kg/m
Step 7: Determine the direction of the current in wire CD
Since wire AB carries a current of 12 A, for wire CD to be repelled and remain suspended, the current in CD must flow in the opposite direction. Therefore, if the current in AB flows from A to B, the current in CD must flow from D to C.
Final Answer
The mass per unit length of wire CD is 1.2×10−3kg/m and the direction of the current in CD is opposite to that in AB.
---
Wire CD is held above wire AB and carries a current. The forces acting on wire CD are:
- The gravitational force (weight) acting downward, Fg=mg
- The magnetic force due to the interaction with wire AB, acting upward.
For wire CD to remain suspended, the magnetic force must balance the weight of the wire. Therefore, we have:
Fm=Fg
Where:
- Fm is the magnetic force acting on wire CD.
- Fg=mg is the weight of wire CD.
The magnetic force per unit length between two parallel wires carrying currents I1 and I2 separated by a distance d is given by:
Fm=μ0I1I22πd⋅L
Where:
- μ0=4π×10−7T m/A (permeability of free space)
- I1=12A (current in wire AB)
- I2=5A (current in wire CD)
- d=1mm=1×10−3m
The mass per unit length of wire CD is denoted as λ. The weight of wire CD can be expressed as:
Fg=λg
Substituting this into the equilibrium condition gives:
μ0I1I22πd⋅L=λg
Rearranging the equation, we have:
λ=μ0I1I22πdg
Substituting the known values into the equation:
λ=(4π×10−7)×12×52π×(1×10−3)×10
Simplifying this:
- The π cancels out:
λ=4×10−7×12×52×(1×10−3)×10
- Calculating the numerator:
4×12×5=240
- The denominator:
2×10−3×10=2×10−2
Thus:
λ=240×10−72×10−2=240×10−72×10−2=120×10−5=1.2×10−3kg/m
Since wire AB carries a current of 12 A, for wire CD to be repelled and remain suspended, the current in CD must flow in the opposite direction. Therefore, if the current in AB flows from A to B, the current in CD must flow from D to C.
The mass per unit length of wire CD is 1.2×10−3kg/m and the direction of the current in CD is opposite to that in AB.
Topper's Solved these Questions
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - [Set- II]4videos
XII BOARDS
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - [Set-III]3videos
XII BOARDS
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET-III, DELHI BOARD4videos
XII BOARDS
- BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D6videos
SAMPLE PAPER 2019
Similar Questions
A wire AB is carrying a steady current of 10A and is lying on the table. Another wire CD carrying 6A is held directly above AB at a height of 2 mm. Find the mass per unit length of the wire CD so that it remains suspended at its position when left free. Give the direction of the current flowing in CD with respect to that in AB. [Take the value of g=10ms−2]
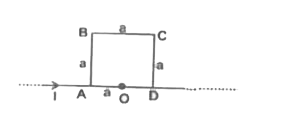
View SolutionThe resistance per unit length of the wires in segments AB, BC, CD, and AD are the same. The magnetic field due to the current at point O is
 View Solution
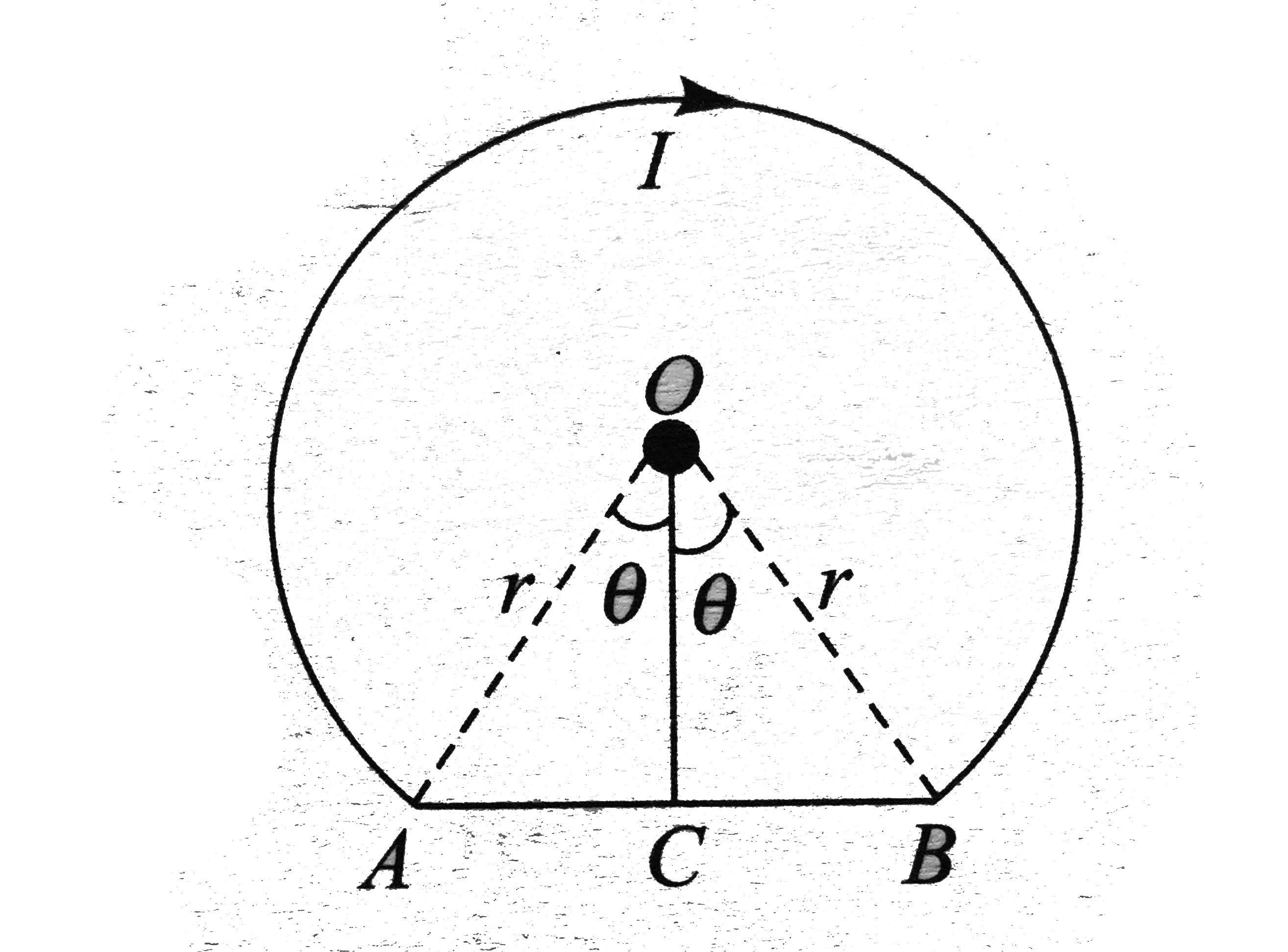
View SolutionA wire is bent in the form of a circular arc with a straight portion AB. Magnetic induction at O when current flowing in the wire, is
 View Solution
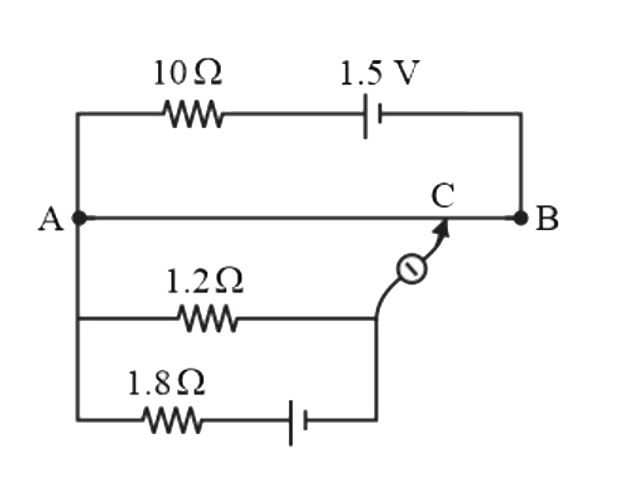
View SolutionIn the below circuit , AB is a wire of length 100 cm with 5Ω resistance . If there is no deflection in the galvanometer , the current flowing in the wire AB is
 View Solution
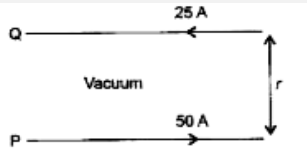
View SolutionA long horizontal wire P carries a current of 50A. It is rigidly fixed. Another wire Q is placed directly above and parallel to P, as shown in figure below. The weight per unit length of the wire Q is 0.025 Nm−1 and it carries a current of 25A. Find the distance .r. of the wire Q from the wire P so that the wire Q remains at rest.
 View Solution
View SolutionTwo long parallel copper wires carry current of 5A each in opposite directions. If the wires are separated by a distance distance of 0.5m, then the force per unit length between the two wires is
View SolutionA straight wire of diametre 0.5 mm carrying a current of 1 A is replaced by another wire of 1mm diametre carrying same current. The strenth of magnetic field far away is
View SolutionTwo parallel wires carry currents of 20A and 40A in opposite directions. Another wire carrying a current antiparallel to 20A is placed midway between the two wires. The magnetic force on it will be
View SolutionWhen two parallel wires carrying current l and 2l in same direction are placed at a distance d apart, the ratio of force per unit length acting upon the wire due to other is
View SolutionCalculate force per unit length acting on the wire B due to the current flowing in the wire A. (see figure below)
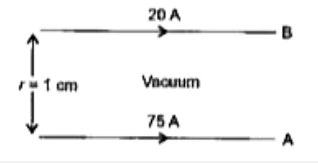 View Solution
View Solution

