A potentiometer wire of length 1 m has a resistance of 10Ω. It is connected to a 6V battery in series with a resistance of 5Ω. Determine the emf of the primary cell which gives a balance point at 40cm.
To solve the problem step by step, we will follow these calculations:
Step 1: Understand the Circuit Configuration
We have a potentiometer wire of length 1 meter (100 cm) with a resistance of 10 Ω. It is connected to a 6V battery in series with a 5Ω resistor. We need to find the EMF of a primary cell that gives a balance point at 40 cm on the potentiometer.
Step 2: Calculate the Total Resistance in the Circuit
The total resistance Rtotal in the circuit is the sum of the resistance of the potentiometer wire R0 and the series resistor R:
Rtotal=R0+R=10Ω+5Ω=15Ω
Step 3: Calculate the Current in the Circuit
Using Ohm's law, the current I flowing through the circuit can be calculated using the total voltage V and the total resistance Rtotal:
I=VRtotal=6V15Ω=0.4A
Step 4: Calculate the Potential Difference Across the Potentiometer Wire
The potential difference VAB across the potentiometer wire can be calculated using the current I and the resistance R0:
VAB=I×R0=0.4A×10Ω=4V
Step 5: Calculate the Potential Gradient (k)
The potential gradient k along the potentiometer wire is given by:
k=VABL=4V100cm=0.04V/cm
Step 6: Calculate the EMF of the Primary Cell
The EMF ϵ of the primary cell that gives a balance point at 40 cm can be calculated using the potential gradient k:
ϵ=k×l=0.04V/cm×40cm=1.6V
Final Answer
The EMF of the primary cell is 1.6V.
---
We have a potentiometer wire of length 1 meter (100 cm) with a resistance of 10 Ω. It is connected to a 6V battery in series with a 5Ω resistor. We need to find the EMF of a primary cell that gives a balance point at 40 cm on the potentiometer.
The total resistance Rtotal in the circuit is the sum of the resistance of the potentiometer wire R0 and the series resistor R:
Rtotal=R0+R=10Ω+5Ω=15Ω
Using Ohm's law, the current I flowing through the circuit can be calculated using the total voltage V and the total resistance Rtotal:
I=VRtotal=6V15Ω=0.4A
The potential difference VAB across the potentiometer wire can be calculated using the current I and the resistance R0:
VAB=I×R0=0.4A×10Ω=4V
The potential gradient k along the potentiometer wire is given by:
k=VABL=4V100cm=0.04V/cm
The EMF ϵ of the primary cell that gives a balance point at 40 cm can be calculated using the potential gradient k:
ϵ=k×l=0.04V/cm×40cm=1.6V
The EMF of the primary cell is 1.6V.
Topper's Solved these Questions
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - [SET - II DELHI BOARD]
5 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - [SET - III, DELHI BOARD]
6 Videos
- XII BOARDSBOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - XII BOARDSEXERCISE - SET-II
33 Videos
- SAMPLE PAPER 2019BOOK - XII BOARD PREVIOUS YEAR PAPER ENGLISHCHAPTER - SAMPLE PAPER 2019EXERCISE - SECTION D
6 Videos
Similar Questions
A potentiometer wire of length 1.0 m has a resistance of 15 ohm. It is connected to a 5 V source in series with a resistance of 5Ω. Determine the emf of the primary cell which has a balance point at 60 cm.
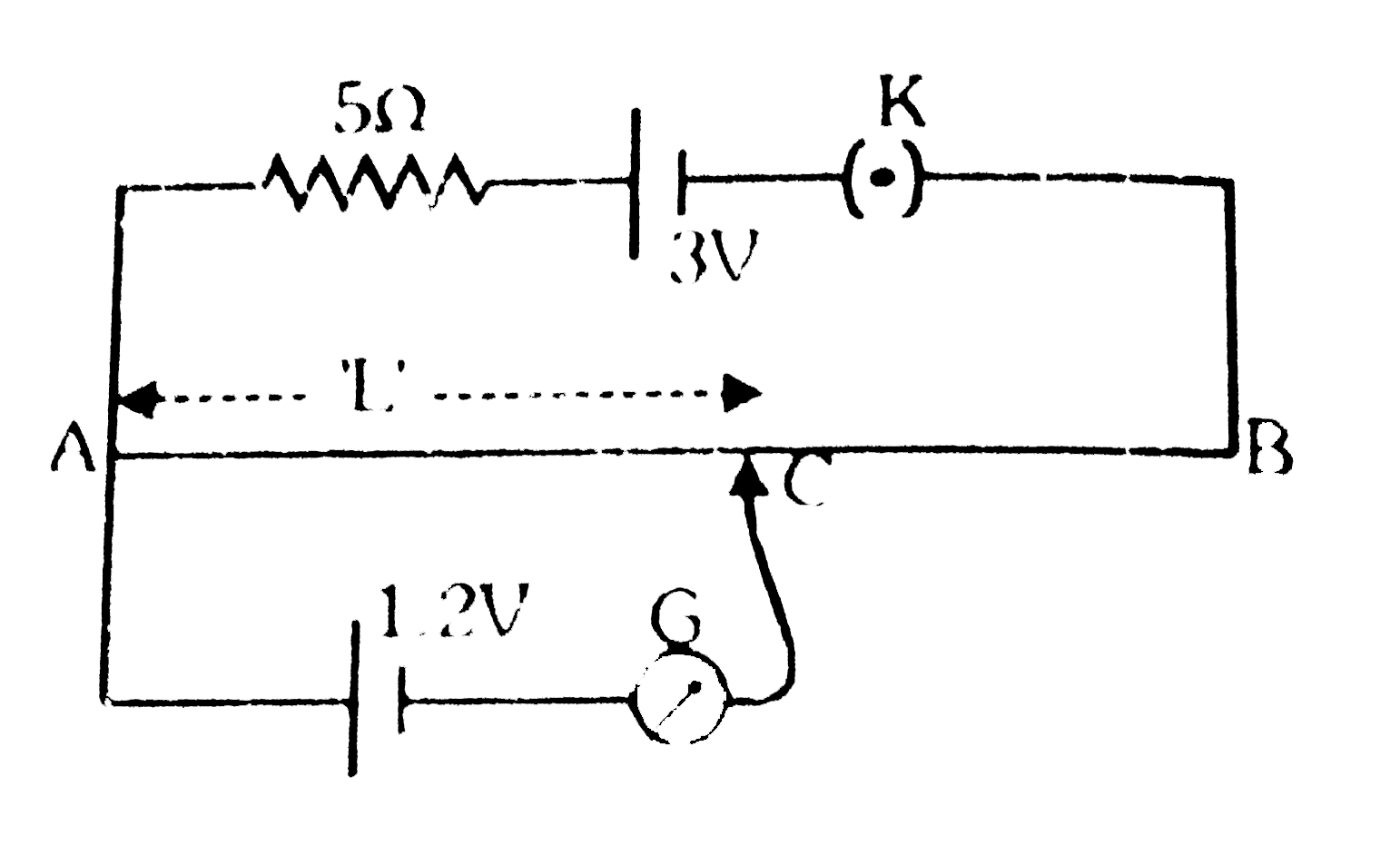
View SolutionThe potentiometer wire of length 100cm has a resistance of 10Ω. It is connected in series with a resistance of 5Ω and an accumulaot or emf 3V having negligible resistance. A source 1.2V is balanced against a length 'L' of the potentionmeter wire. find the value of L.
View Solution
Knowledge Check
A potentiometer wire of length 100 cm has a resistance of 100Ω it is connected in series with a resistance and a battery of emf 2 V and of negligible internal resistance. A source of emf 10 mV is balanced against a length of 40 cm of the potentiometer wire. what is the value of the external resistance?
A790ΩB890ΩC990ΩD1090ΩA potentiometer wire of length 100cm has a resistance of 10Ω. It is connected in series with a resistance and a cell of emf 2V and of negligible interal resistance. A source of emf 10mV is balanced against a length of 40cm of the potentiometer wire. What is the value of external resistance?
A790ΩB890ΩC990ΩD1090Ω
A potentiometer wire of length 100 cm has a resistance of 10Ω. It is connected in series with a resistance R and cell of emf 2V and negligible resistance. A source of emf 10 mV is balanced against a length of 40cm of the potentiometer wire. What is the value of R?
View SolutionA potentiometer wire is 10 m long and has a resistance of 18Ω. It is connected to a battery of emf 5 V and internal resistance 2Ω. Calculate the potential gradient along the wire.
View SolutionA potentiometer wire of length 1m and resistance 20Ω is connected in series with a 15V battery and an external resistance 40Ω . A secondary cell of emf E in the secondary circuit is balanced by 24 cm long potentiometer wire. The emf E of the cell is ,
View SolutionA potentiometer wire of length 100cm having a resistance of 10Ω is connected in series with a resistance R and a cell of emf 2V of negligible internal resistance. A source of emf

of 10mV is balanced against a length of 40cm of the potentiometer wire. What is the value of resistance R ?
View SolutionA potentiometer wire of length 100cm having a resistance of 10Ω is connected in series with a resistance R and a cell of emf 2V of negligible internal resistance. A source of emf

of 10mV is balanced against a length of 40cm of the potentiometer wire. What is the value of resistance R ?
View SolutionAB is a potentiometer wire of length 100 cm and its resistance is 10Ω. It is connected in series with a resistance R = 40 Ω and a battery of emf 2 V and negligible internal resistance. If a source of unknown emf E is balanced by 40 cm length of the potentiometer wire, the value of E is
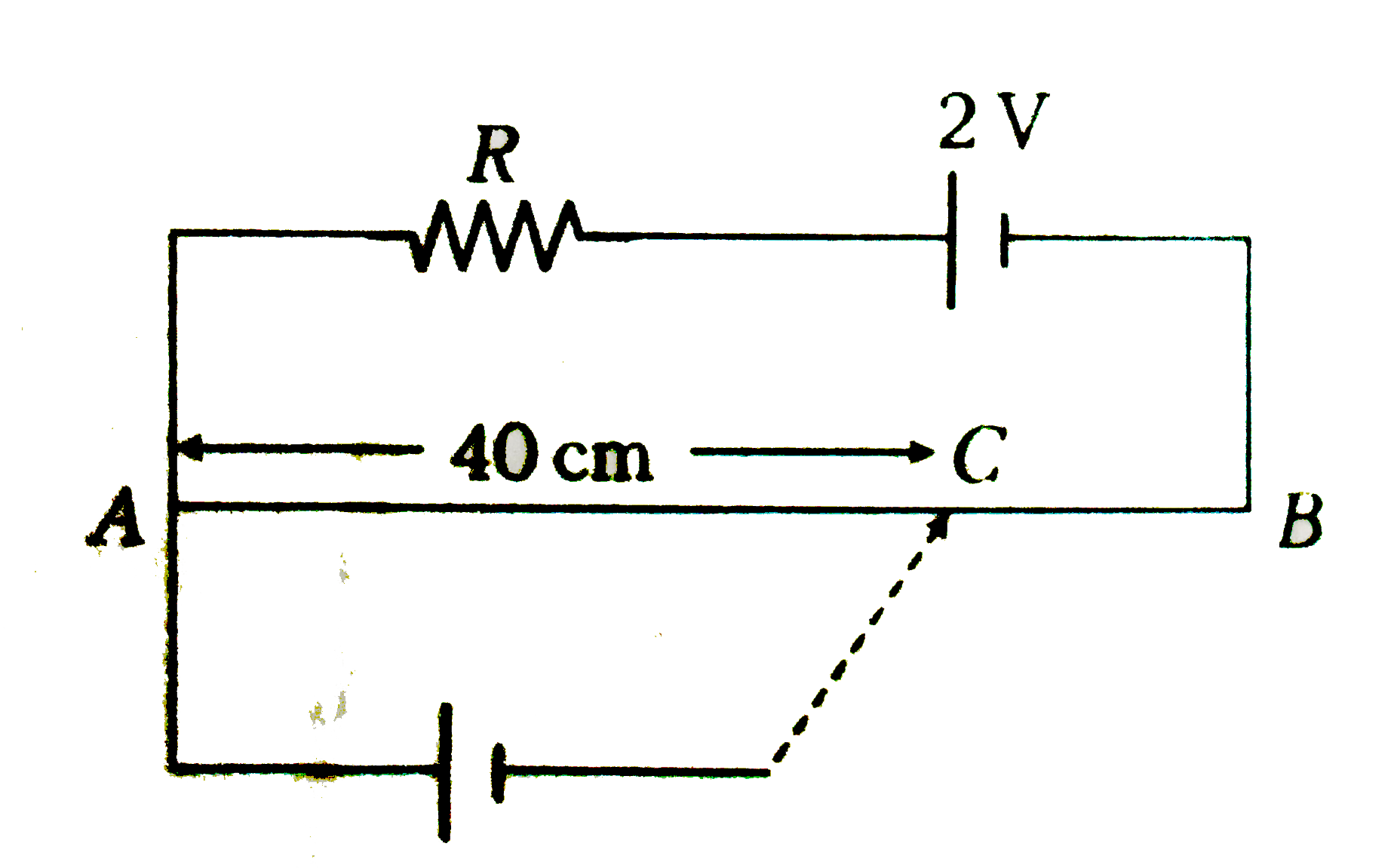
View Solution
