Prove that the parallelogram circumscribing a circle is a rhombus.
Step by step video solution for Prove that the parallelogram circumscribing a circle is a rhombus. by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
If all the side of a parallelogram touch a circle, show that the parallelogram is a rhombus. OR Prove that a parallelogram circumscribing a circle is a rhombus.
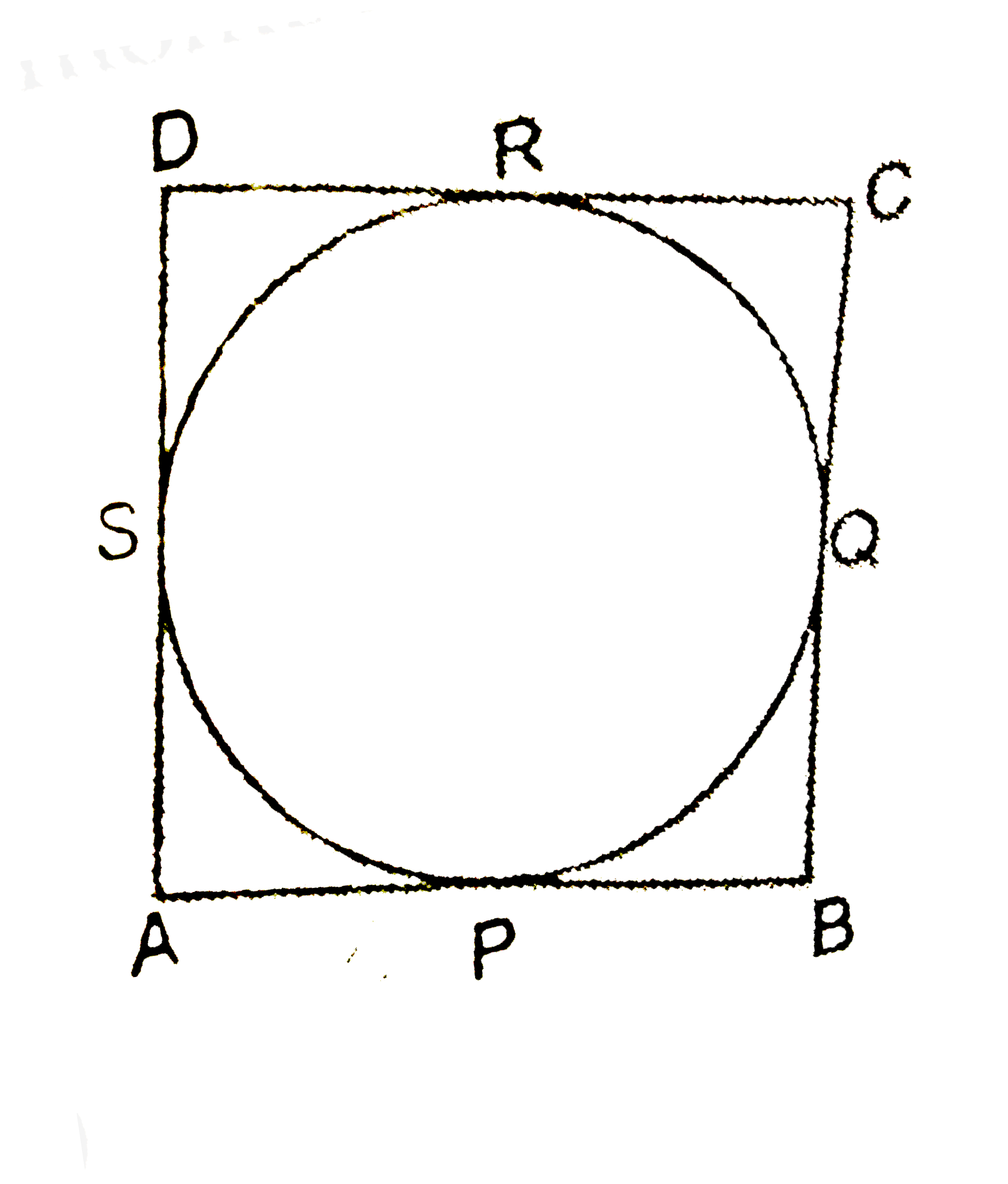
View SolutionProve that the rectangle circumscribing a circle is a square
View SolutionProve that :
the parallelogram , inscirbed in a circle , is a rectangle.
View SolutionProve that :
the rhombus , inscribed in a circle is a square.
View SolutionProve that any cyclic parallelogram is a rectangle.
View SolutionProve that any cyclic parallelogram is a rectangle.
View SolutionIf a parallelogram circumscribes a circle then prove that it must be a rhombus.
View SolutionProve that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
View SolutionProve that opposite sides of a quadrilateral circumscribing a circle subtend supplementary angles at the centre of the circle.
View SolutionProve that the centre of the circle circumscribing the cyclic rectangle ABCD is the point of intersection of its diagonals.
View Solution
