Two equal resistors are connected in series. Draw the circuit diagram for determining the resultant resistance.
To determine the resultant resistance of two equal resistors connected in series, we can follow these steps:
1. Understand the Concept of Series Connection:
- In a series connection, the same current flows through all components connected in the circuit. The total or resultant resistance can be calculated by simply adding the individual resistances.
2. Identify the Components:
- We have two equal resistors. Let's denote their resistance as \( R \).
3. Draw the Circuit Diagram:
- Start by drawing a battery (power source) to provide voltage. Label it as \( V \) volts.
- Draw the first resistor (R) connected to the positive terminal of the battery.
- Connect the second resistor (R) in series with the first resistor. This means the end of the first resistor connects to one end of the second resistor.
- Finally, connect the other end of the second resistor back to the negative terminal of the battery, completing the circuit.
4. Label the Circuit:
- Clearly label both resistors as \( R \) and \( R \).
- Indicate the current \( I \) flowing through the circuit, which is the same through both resistors since they are in series.
5. Calculate the Resultant Resistance:
- Since both resistors are equal and in series, the resultant resistance \( R_{total} \) can be calculated using the formula:
\(
R_{total} = R + R = 2R
\)
Circuit Diagram:
±−−−(R)−−−−(R)−−−−+|∣||∣|±−−−−−−−−∣⊢−−−−−−−+V
- In a series connection, the same current flows through all components connected in the circuit. The total or resultant resistance can be calculated by simply adding the individual resistances.
- We have two equal resistors. Let's denote their resistance as \( R \).
- Start by drawing a battery (power source) to provide voltage. Label it as \( V \) volts.
- Draw the first resistor (R) connected to the positive terminal of the battery.
- Connect the second resistor (R) in series with the first resistor. This means the end of the first resistor connects to one end of the second resistor.
- Finally, connect the other end of the second resistor back to the negative terminal of the battery, completing the circuit.
- Clearly label both resistors as \( R \) and \( R \).
- Indicate the current \( I \) flowing through the circuit, which is the same through both resistors since they are in series.
- Since both resistors are equal and in series, the resultant resistance \( R_{total} \) can be calculated using the formula:
\(
R_{total} = R + R = 2R
\)
±−−−(R)−−−−(R)−−−−+|∣||∣|±−−−−−−−−∣⊢−−−−−−−+V
Similar Questions
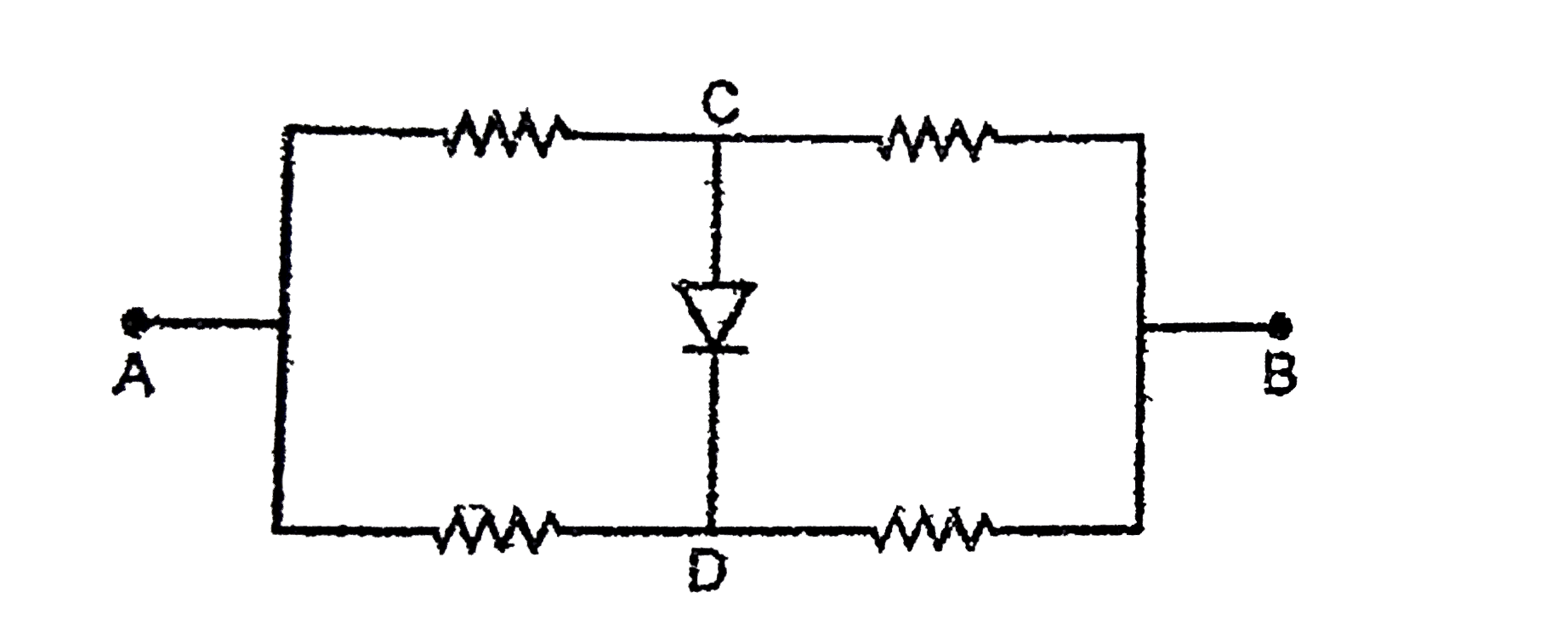
Four equal resistors, each of resistance 10 ohm are connected as shown in the adjoining circuit diagram. Then the equivalent resistance between points A and B is-View Solution(a) Resistors given as R1,R2andR3 are connected in series to a battery V. Draw the circuit diagram showing the arrangement. Derive an expression for the equivalent resistance of the combination.
(b) If R1=10Ω,R2=20ΩandR3=30Ω, calculate the effective resistance when they are connected in series to a battery of 6 V. Also find the current flowing in the circuit.View Solution
Knowledge Check
- Question 1 - Select One
Two 2Ω resistances are connected in parallel in the circuit X and in series in circuit Y. The batteries in the two circuits are identical and have zero internal resistance. Assume that the energy transferred to resistor A in circuit X within a certain time is W. The energy transferred to resistor B in circuit Y in the same time will be
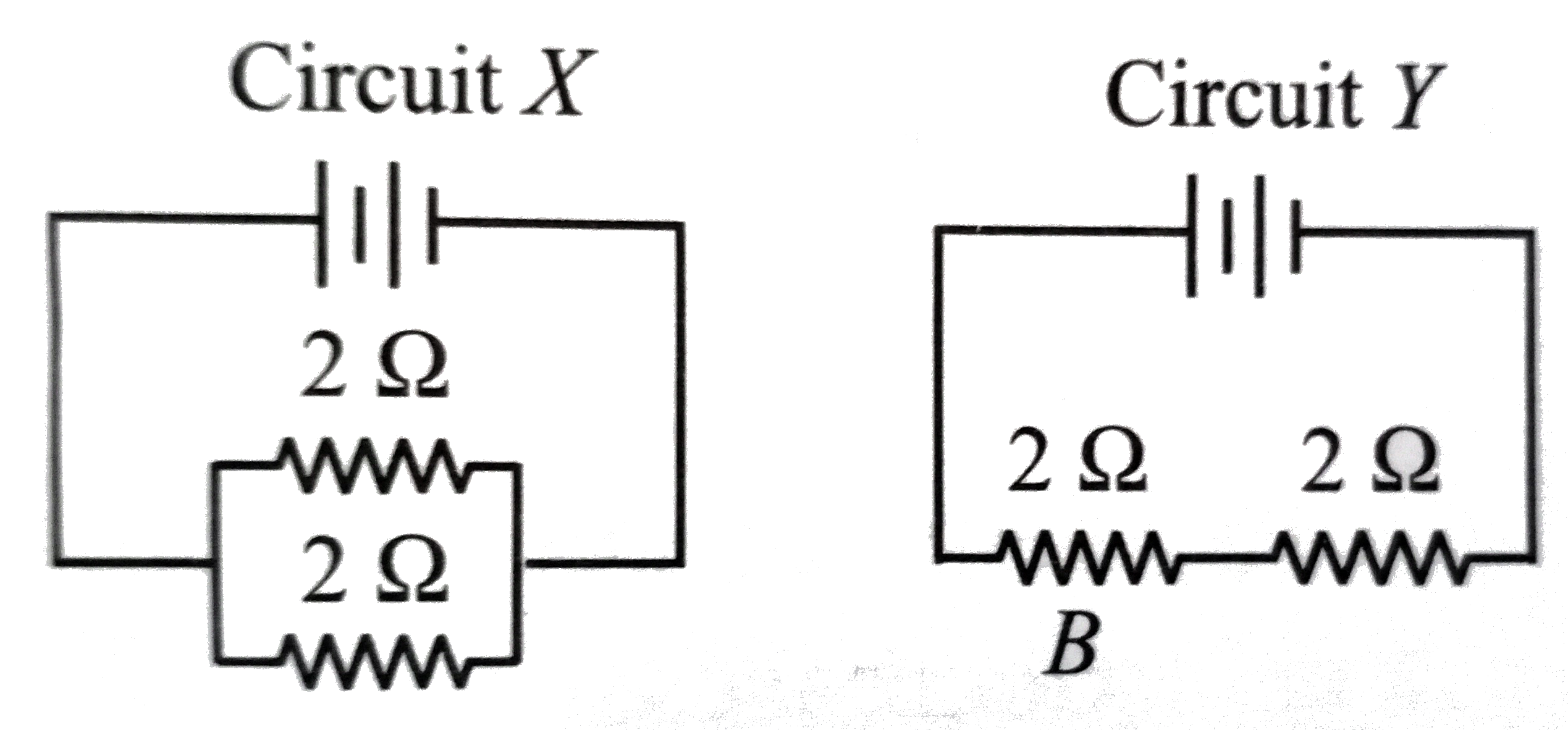 A14WB12WC2WD4W
A14WB12WC2WD4W
n equal resistors are first connected in series and then connected in parallel. What is the ratio of the maximum to the minimum resistance ?
View SolutionOut of five resistance of RΩ each 3 are connected in parallel and are joined to the rest 2 in series. Find the resultant resistance.
View SolutionThree resistors of 5Ω,10Ωand15Ω are connected in series and the combination is connected to battery of 30 V. Ammeter and Voltmeter are connected in the circuit. Draw a circuit diagram to connect all the devices in proper correct order. What is the corrent flowing and potential difference across 10Ω resistance?
View SolutionA cell supplies a current of 1.2 A through two resistors each of 2 Ω connected in parallel. When the resistors are connected in series, it supplies a current of 0.4 A. Calculate :
the internal resistance,View SolutionSixteen resistor, each resistane 16Ω are connected in the circuit as shown in fig. the net resistance between A and B is
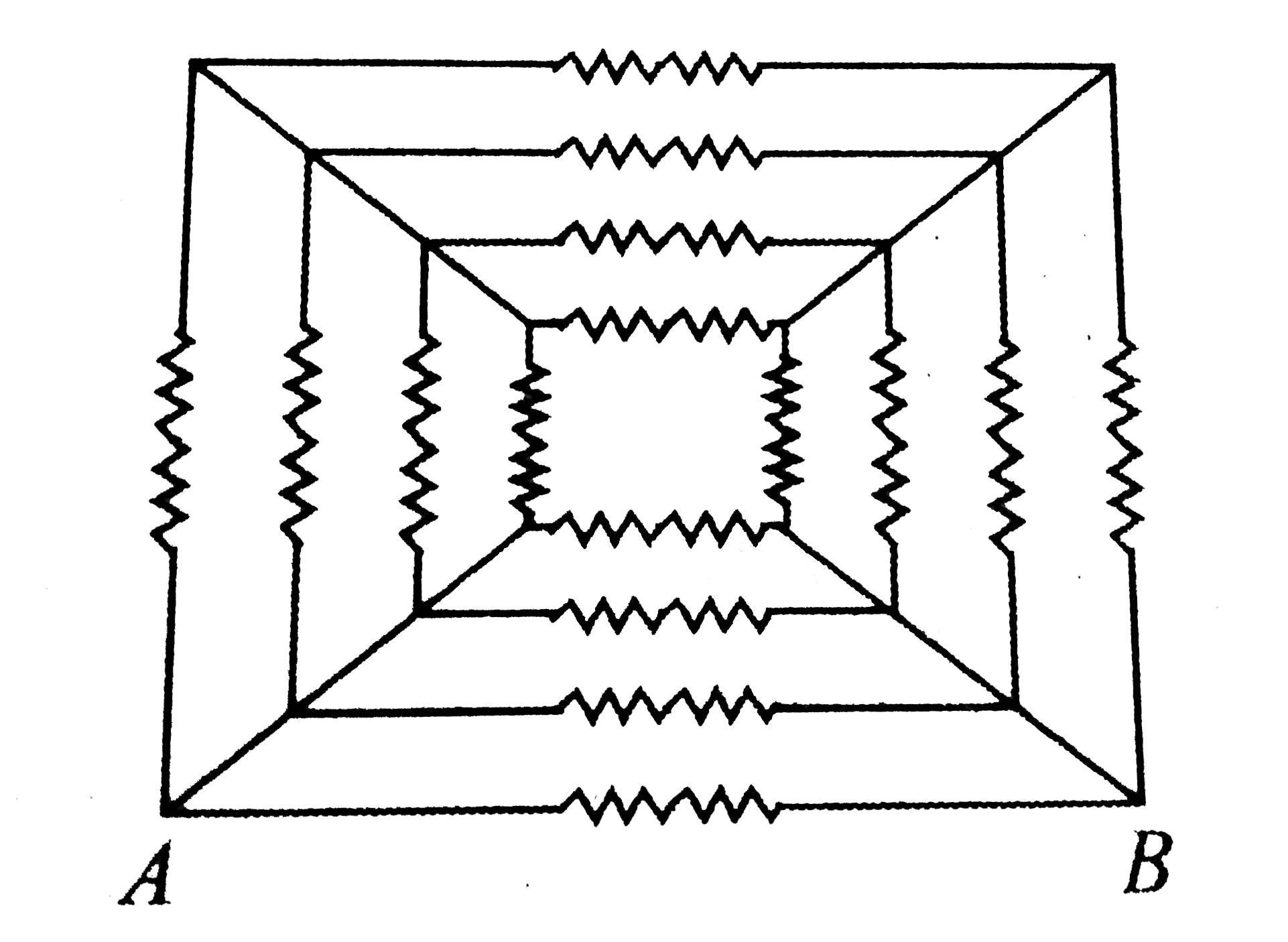 View Solution
View SolutionHow would you connect two resistors in series ? Draw a diagram. Calculate the total equivalent resistance.
View SolutionFour equal resistors, each of resistance 10Ω, are connected as shown in the circuit diagram. The equivalent resistance between A and B is
View Solution

