How would you connect two resistors in series ? Draw a diagram. Calculate the total equivalent resistance.
To connect two resistors in series and calculate the total equivalent resistance, follow these steps:
Step 1: Connect the Resistors
Connect the two resistors, R1 and R2, end-to-end. This means that the end of R1 is connected to the beginning of R2.
Diagram:
±−−−−R1−−−−−+∣|∣|±−−−−R2−−−−−+
In the diagram, the two resistors are connected in series.
Step 2: Understand the Voltage and Current Relationships
When resistors are connected in series, the same current (I) flows through both resistors. The total voltage (V) across the series combination is the sum of the voltage drops across each resistor.
Step 3: Write the Voltage Equation
The voltage drop across each resistor can be expressed as:
- Voltage drop across R1: \( V_1 = I \times R_1 \)
- Voltage drop across R2: \( V_2 = I \times R_2 \)
The total voltage (V) across the series combination is:
\( V = V_1 + V_2 \)
\( V = I \times R_1 + I \times R_2 \)
Step 4: Factor Out the Current
Factoring out the current (I) from the equation gives:
\( V = I \times (R_1 + R_2) \)
Step 5: Calculate the Total Equivalent Resistance
From Ohm's Law, we know that:
\( V = I \times R_{equivalent} \)
Setting the two equations for V equal to each other:
\( I \times R_{equivalent} = I \times (R_1 + R_2) \)
Since the current (I) is the same in both cases, we can cancel it out:
\( R_{equivalent} = R_1 + R_2 \)
Final Answer:
The total equivalent resistance \( R_{equivalent} \) for two resistors in series is:
\( R_{equivalent} = R_1 + R_2 \)
---
Connect the two resistors, R1 and R2, end-to-end. This means that the end of R1 is connected to the beginning of R2.
±−−−−R1−−−−−+∣|∣|±−−−−R2−−−−−+
In the diagram, the two resistors are connected in series.
When resistors are connected in series, the same current (I) flows through both resistors. The total voltage (V) across the series combination is the sum of the voltage drops across each resistor.
The voltage drop across each resistor can be expressed as:
- Voltage drop across R1: \( V_1 = I \times R_1 \)
- Voltage drop across R2: \( V_2 = I \times R_2 \)
\( V = V_1 + V_2 \)
\( V = I \times R_1 + I \times R_2 \)
Factoring out the current (I) from the equation gives:
\( V = I \times (R_1 + R_2) \)
From Ohm's Law, we know that:
\( V = I \times R_{equivalent} \)
Setting the two equations for V equal to each other:
\( I \times R_{equivalent} = I \times (R_1 + R_2) \)
\( R_{equivalent} = R_1 + R_2 \)
The total equivalent resistance \( R_{equivalent} \) for two resistors in series is:
\( R_{equivalent} = R_1 + R_2 \)
Topper's Solved these Questions
- CURRENT ELECTRICITYBook:ICSEChapter:CURRENT ELECTRICITYExercise:EXERCISE-8(B) (MULTIPLE CHOICE TYPE)
Explore 3 Videos
- CURRENT ELECTRICITYBook:ICSEChapter:CURRENT ELECTRICITYExercise:EXERCISE-8(B) (NUMERICALS )
Explore 54 Videos
- CURRENT ELECTRICITYBook:ICSEChapter:CURRENT ELECTRICITYExercise:EXERCISE-8(A)(NUMERICALS )
Explore 16 Videos
- CALORIMETRYBook:ICSEChapter:CALORIMETRYExercise:EXERCISE-11(B) (NUMERICALS)
Explore 15 Videos
- ELECTRO-MAGNETISM Book:ICSEChapter:ELECTRO-MAGNETISM Exercise:EXERCISE-10 (C) NUMERICALS
Explore 1 Video
Similar Questions
You have three resistors of values 2Ω,3Ωand5Ω. How will you join them so that the total resistance is more than 7Ω?
Calculate the equivalent resistance.
View SolutionThree resistors are connected to a 6 V battery as shown in the figure
Calculate:
the equivalent resistance of the circuit
View SolutionA constant 60 V supply is connected across the two resistors as shown in diagram. Calculate the reading of the voltmeter which has a resistance of 200 KΩ
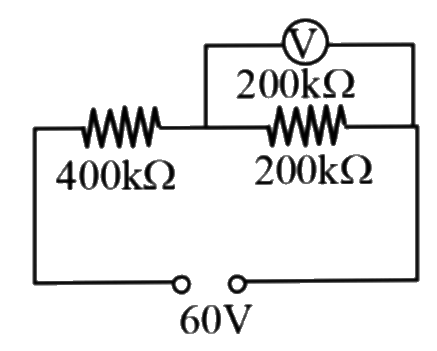
View SolutionTwo resistors of 4 Ω and 6 Ω are connected in parallel. The combination is connected across a 6 V battery of negligible resistance. (i) Draw a circuit diagram. (ii) Calculate : (a) the total resistance of the circuit,
View SolutionThree resistors are connected to a 6 V battery as shown in the figure given below :
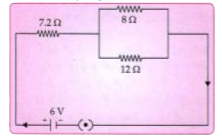
Calculate :
the equivalent resistance of the circuit.
View SolutionThe equivalent resistance of two resistor connected in series is 6Ω and their equivalent resistance is 43Ω. What are the values of resistances ?
View SolutionYou have three resistors of values 2Ω,3Ω and 5 Ω. How will you join them so that the total resistance is less than 1 Ω ? Draw a diagram and find the total resistance.
View SolutionTwo equal resistors are connected in series. Draw the circuit diagram for determining the resultant resistance.
View SolutionHow would you calculate the equivalent mass of a salt ?
View SolutionIdnetical resistor each of resistance r are connected as shown in figure. Calculate equivalent resistance between A and E.
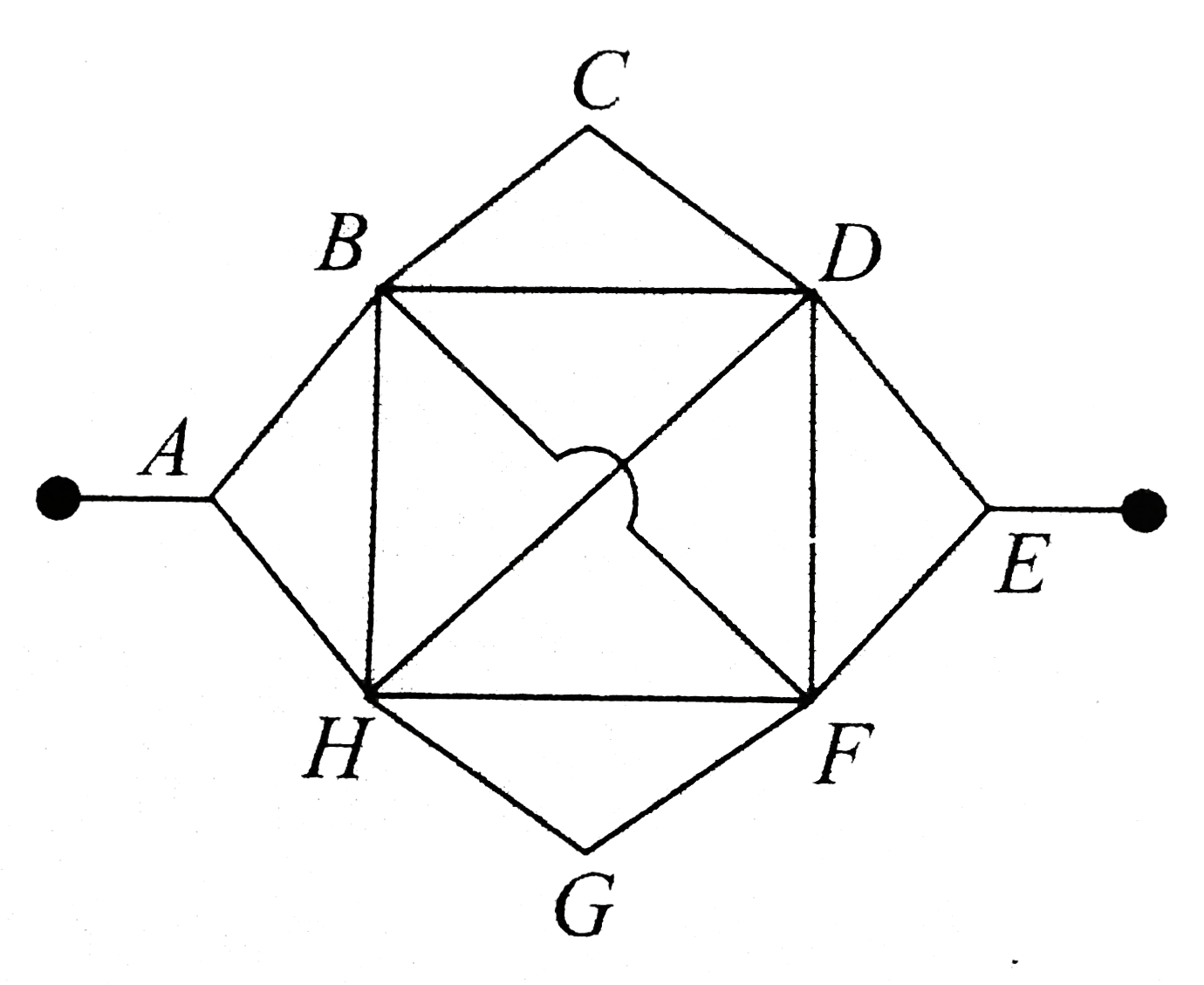
View Solution
