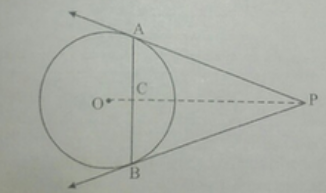
In the figure-3, from an external point P, two tangents PQ and PR are drawn to a circle of the radius 4 cm with centre O. If ∠ QPR = 90∘, then length of PQ is
To solve the problem, we need to find the length of the tangent PQ drawn from an external point P to a circle with a radius of 4 cm, given that the angle QPR is 90 degrees.
1. Understand the Geometry:
- We have a circle with center O and radius 4 cm.
- From point P (external to the circle), two tangents PQ and PR are drawn to the circle.
- The angle QPR is given as 90 degrees.
2. Identify the Key Properties of Tangents:
- The tangent to a circle is perpendicular to the radius at the point of tangency. Therefore, OQ (radius to point Q) is perpendicular to PQ, and OR (radius to point R) is perpendicular to PR.
3. Label the Angles:
- Since OQ is perpendicular to PQ, we have angle OQP = 90 degrees.
- Similarly, since OR is perpendicular to PR, we have angle ORP = 90 degrees.
4. Analyze the Quadrilateral OQPR:
- We know that OQ = OR (both are radii of the circle, which are equal).
- We have angle QPR = 90 degrees, and both angles OQP and ORP are also 90 degrees.
- Thus, quadrilateral OQPR forms a right angle at P.
5. Conclude the Shape:
- Since all angles are right angles and two sides (OQ and OR) are equal, quadrilateral OQPR is a square.
- In a square, all sides are equal.
6. Calculate the Length of PQ:
- The length of the radius OQ is given as 4 cm.
- Therefore, since OQ = PQ (as all sides of the square are equal), we conclude that:
PQ=OQ=4 cm
Final Answer:
The length of PQ is 4 cm.
- We have a circle with center O and radius 4 cm.
- From point P (external to the circle), two tangents PQ and PR are drawn to the circle.
- The angle QPR is given as 90 degrees.
- The tangent to a circle is perpendicular to the radius at the point of tangency. Therefore, OQ (radius to point Q) is perpendicular to PQ, and OR (radius to point R) is perpendicular to PR.
- Since OQ is perpendicular to PQ, we have angle OQP = 90 degrees.
- Similarly, since OR is perpendicular to PR, we have angle ORP = 90 degrees.
- We know that OQ = OR (both are radii of the circle, which are equal).
- We have angle QPR = 90 degrees, and both angles OQP and ORP are also 90 degrees.
- Thus, quadrilateral OQPR forms a right angle at P.
- Since all angles are right angles and two sides (OQ and OR) are equal, quadrilateral OQPR is a square.
- In a square, all sides are equal.
- The length of the radius OQ is given as 4 cm.
- Therefore, since OQ = PQ (as all sides of the square are equal), we conclude that:
PQ=OQ=4 cm
The length of PQ is 4 cm.
Similar Questions
In figure, From an external point P, Two tangents PQ and PR are drawn to a circle of radius 4 cm with centre O . If ∠QPR=90∘ then length of PQ is
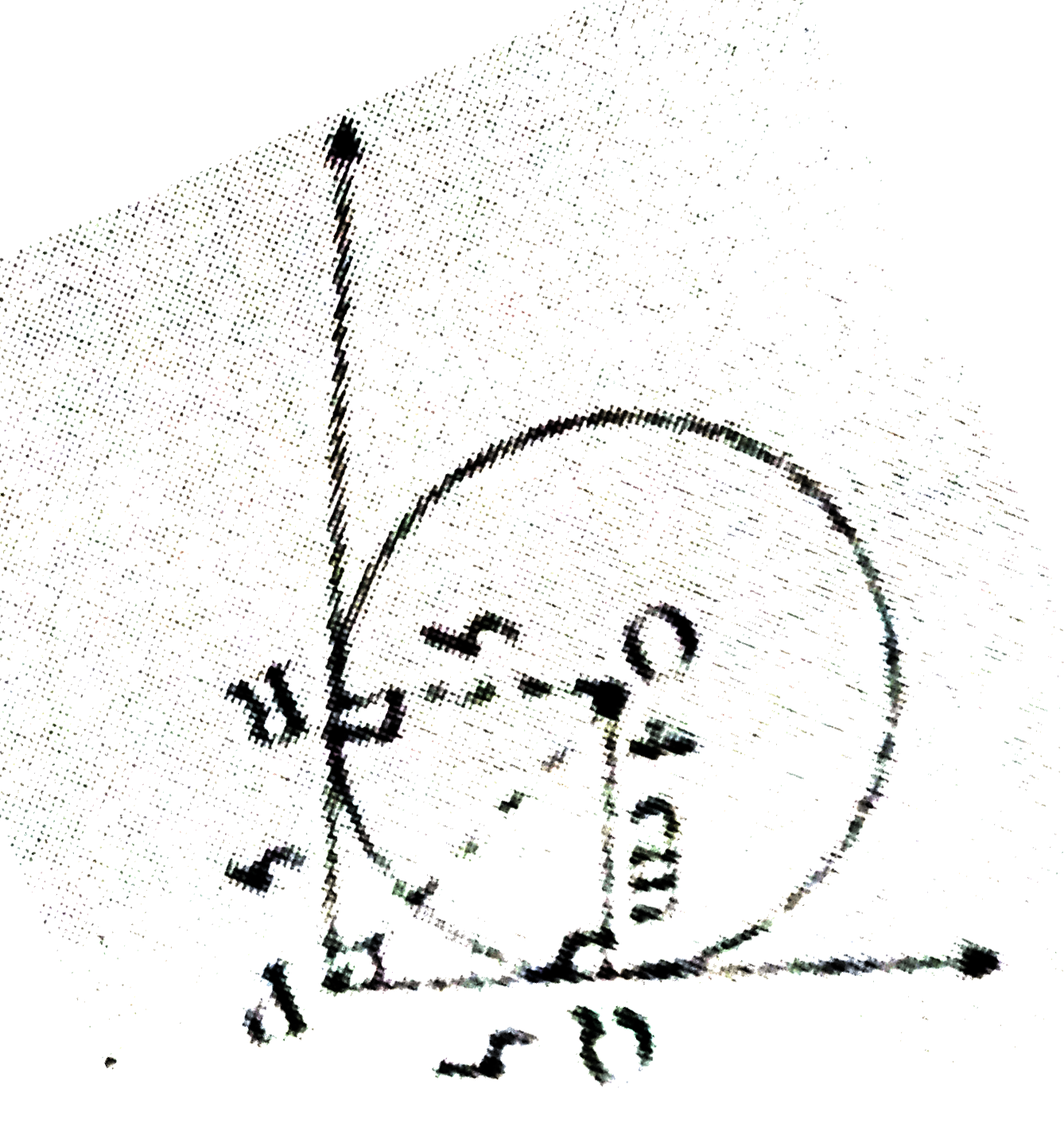
View SolutionIf Figure-1, from an external point P, two tangents PQ and PR are drawn to a circle of radius 4 cm with centre O. if ∠QPR=90∘, then length of PQ is
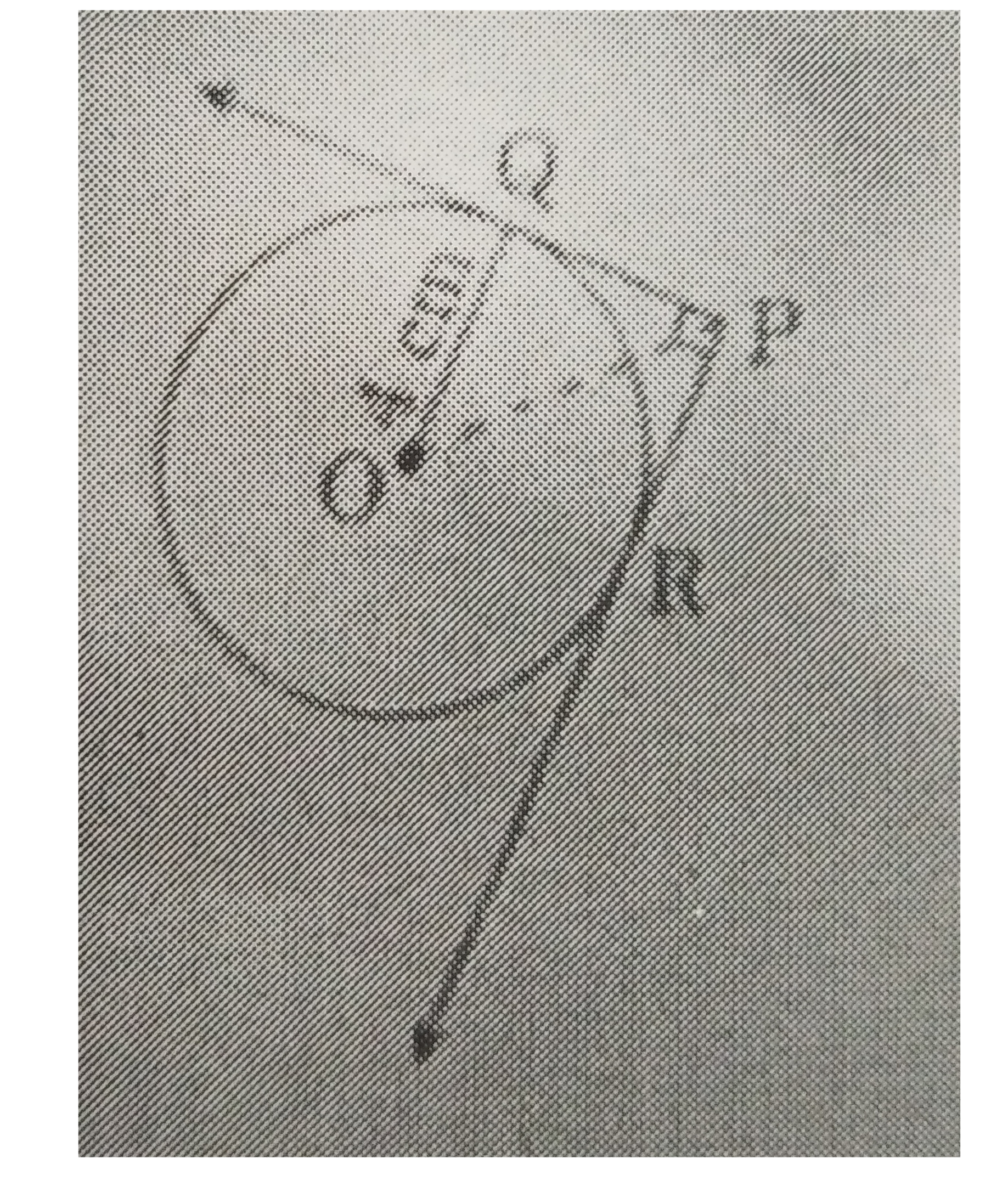
View SolutionFrom an external point P, two tangents PA and PB are drawn to the circle with centre O. Prove that OP is the perpendicular bisector of AB.
View SolutionIn the given figure, from an external point P,two tangents PT and PS are drawn to a circle with centre O and radius r.If OP=2r, show that ∠OTS=∠OST=30∘
View Solutiontwo tangents RQ and RP are drawn from an external point R to the circle with centre O. If ∠PRQ=120∘ , then prove that OR= PR+ RQ
View SolutionFrom a point P, two tangents PA and PB are drawn to a circle with centre O and radius r. If OP=2r, show that △APB is equilateral.
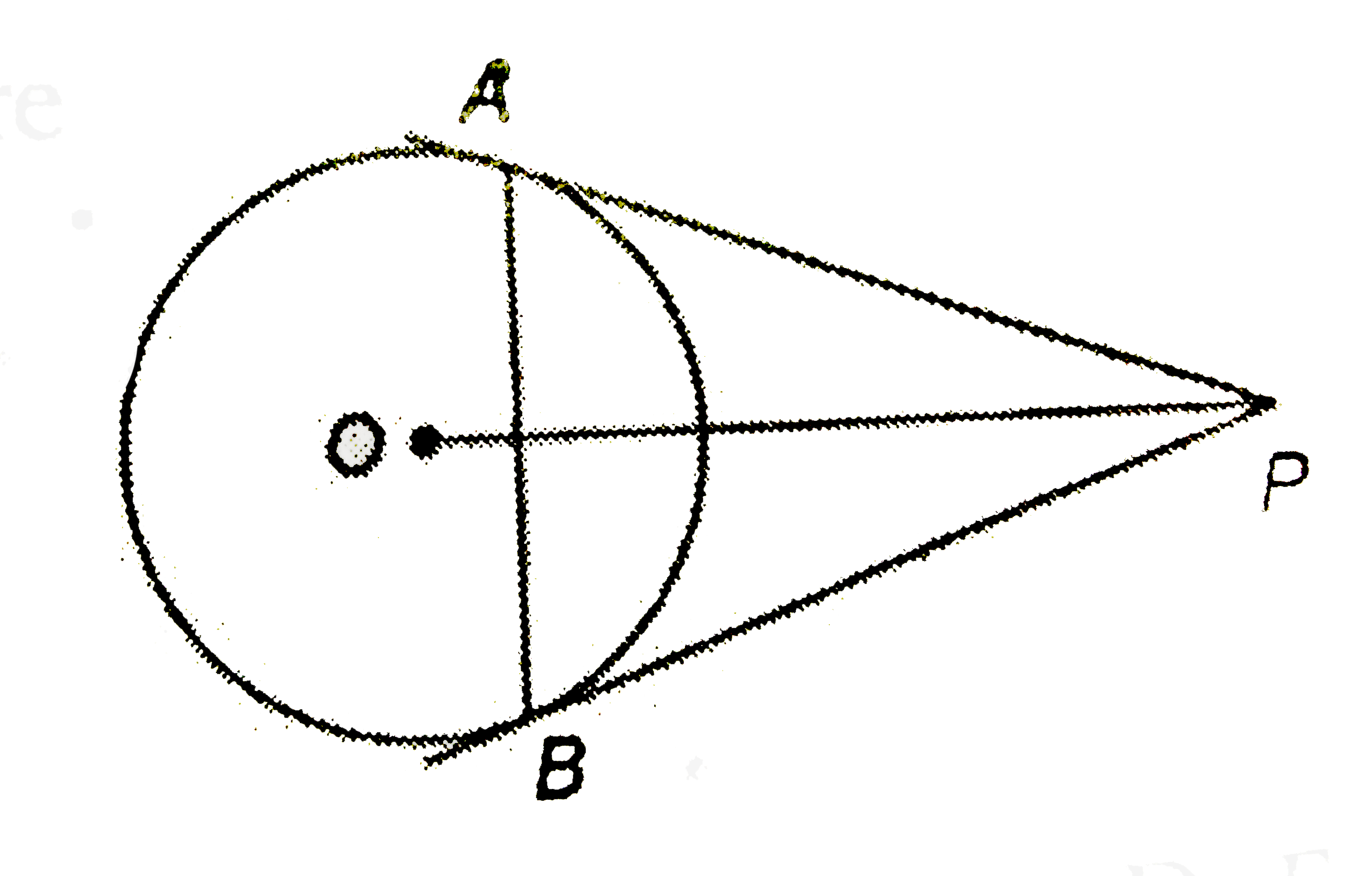
View SolutionFrom a point P , two tangents PA and PB are drawn to a circle with centre O . If OP= diameter of the circle, show that △APB is equilateral.
View SolutionIn Figure, there are two concentric circles with centre O of radii 5cmand3cm . From an external point P, tangents PAandPB are drawn to these circles. If AP=12cm, find the length of BP.
View SolutionIn the given figure, two tangents PQ and PR are drawn to a circle with centre O from an external point P, Prove that ∠QPR=2∠OQR.
View SolutionIn the given figure, two tangents PQ and PR are drawn to a circle with centre C from an external point P, Prove that ∠QPR=2∠OQR.
View Solution
