If (log)√3(|z|2−|z|+12+|z|)>2, then locate the region in the Argand plane which represents z.
To solve the inequality log√3(|z|2−|z|+12+|z|)>2, we will follow these steps:
Step 1: Rewrite the logarithmic inequality
We start by rewriting the logarithmic inequality in exponential form:
|z|2−|z|+12+|z|>3
This is because √32=3.
Step 2: Clear the fraction
Next, we will multiply both sides by 2+|z| (noting that 2+|z|>0):
|z|2−|z|+1>3(2+|z|)
This simplifies to:
|z|2−|z|+1>6+3|z|
Step 3: Rearrange the inequality
Now, we will move all terms to one side:
|z|2−|z|−3|z|+1−6>0
This simplifies to:
|z|2−4|z|−5>0
Step 4: Factor the quadratic expression
Next, we factor the quadratic:
(|z|−5)(|z|+1)>0
Step 5: Analyze the factors
The product (|z|−5)(|z|+1)>0 will be positive in two cases:
1. |z|−5>0 and |z|+1>0 (which is always true since |z|≥0)
2. |z|−5<0 and |z|+1<0 (not possible since |z|+1 is always positive)
Thus, we only consider the first case:
|z|>5
Step 6: Interpret the result in the Argand plane
The inequality |z|>5 indicates that the point z lies outside the circle of radius 5 centered at the origin in the Argand plane.
Conclusion
The region in the Argand plane that represents z is the area outside the circle of radius 5 centered at the origin.
---
We start by rewriting the logarithmic inequality in exponential form:
|z|2−|z|+12+|z|>3
This is because √32=3.
Next, we will multiply both sides by 2+|z| (noting that 2+|z|>0):
|z|2−|z|+1>3(2+|z|)
This simplifies to:
|z|2−|z|+1>6+3|z|
Now, we will move all terms to one side:
|z|2−|z|−3|z|+1−6>0
This simplifies to:
|z|2−4|z|−5>0
Next, we factor the quadratic:
(|z|−5)(|z|+1)>0
The product (|z|−5)(|z|+1)>0 will be positive in two cases:
1. |z|−5>0 and |z|+1>0 (which is always true since |z|≥0)
2. |z|−5<0 and |z|+1<0 (not possible since |z|+1 is always positive)
|z|>5
The inequality |z|>5 indicates that the point z lies outside the circle of radius 5 centered at the origin in the Argand plane.
The region in the Argand plane that represents z is the area outside the circle of radius 5 centered at the origin.
Topper's Solved these Questions
Similar Questions
Knowledge Check
The locus of the point z is the Argand plane for which |z+1|2+|z−1|2=4 is a
AStraight lineBPair of straight linesCParabolaDCircle
The pressure-volume of various thermodynamic process is shown in graphs:
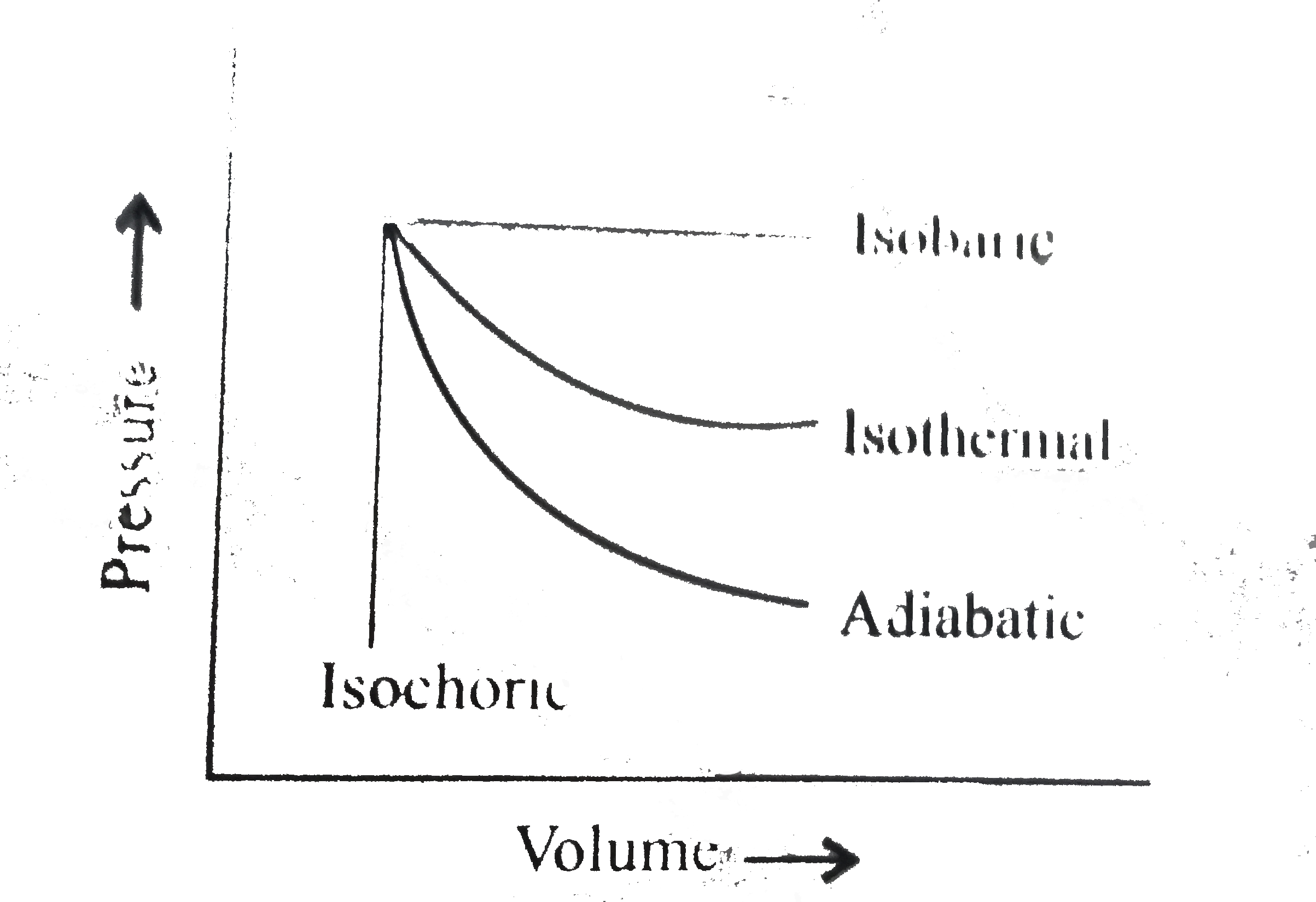
Work is the mole of transference of energy. It has been observed that reversible work done by the system is the maximum obtainable work.
wrev>wirr
The works of isothermal and adiabatic processes are different from each other.
wisothermal reversible=2.303nRTlog10(V2V1)
=2.303nRTlog10(P2P1)
wadiabatic reversible=CV(T1−T2)
A thermodynamic system goes in a cyclic process as represented in the following P−V diagram:
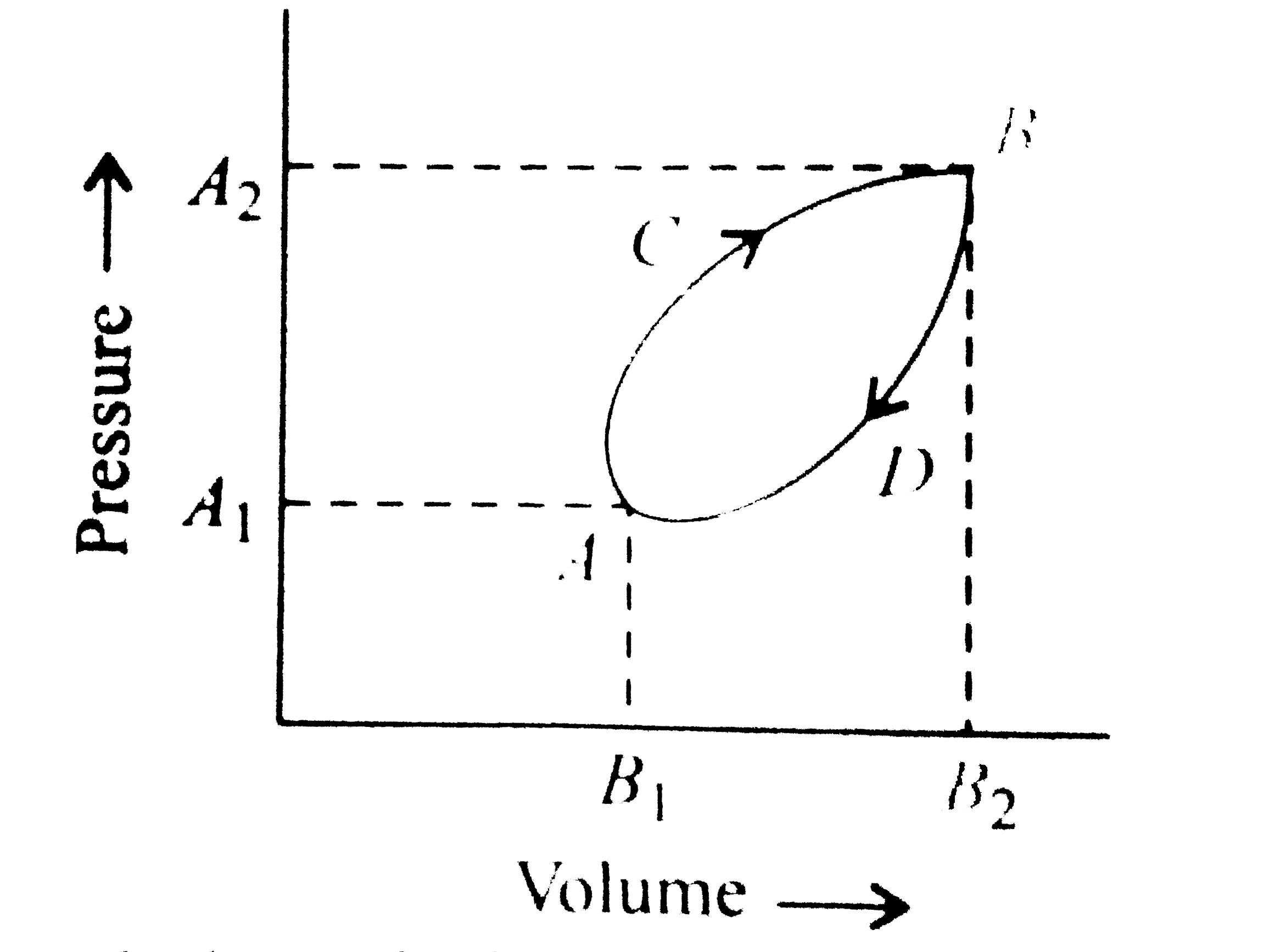
The net work done during the complete cycle is given by the area
View SolutionLocate the region in the Argand plane determined by z2+z2+2∣∣z2∣∣<(8i(z−z)) .
View SolutionIf |z+3i|+|z−i|=8, then the locus of z, in the Argand plane, is
View SolutionIf logtan30∘[2|z|2+2|z|−3|z|+1]<−2 then |z|=
View SolutionIf z=ilog(2−√3) then cosz
View SolutionIf z is a comlex number in the argand plane, the equation |z−2|+|z+2|=8 represents
View SolutionIf the points z1,z2,z3 are the vertices of an equilateral triangle in the Argand plane, then which one of the following is not correct?
View Solution
