If then locus of z is
To solve the problem, we need to analyze the inequality given in the question step by step.
Step 1: Rewrite the logarithmic inequality
We start with the inequality:
log√3∣∣
∣∣|z|2−|z|+1|z|+2∣∣
∣∣<2
Taking the antilogarithm, we have:
∣∣
∣∣|z|2−|z|+1|z|+2∣∣
∣∣<32
which simplifies to:
∣∣
∣∣|z|2−|z|+1|z|+2∣∣
∣∣<9
Step 2: Remove the absolute value
Since both the numerator and denominator are positive for all |z|≥0, we can drop the absolute value:
|z|2−|z|+1|z|+2<9
Step 3: Cross-multiply
Cross-multiplying gives:
|z|2−|z|+1<9(|z|+2)
Expanding the right side:
|z|2−|z|+1<9|z|+18
Step 4: Rearranging the inequality
Rearranging the terms leads to:
|z|2−10|z|−17<0
Step 5: Finding the roots of the quadratic equation
To solve the quadratic inequality, we first find the roots of the equation:
|z|2−10|z|−17=0
Using the quadratic formula:
|z|=−b±√b2−4ac2a=10±√(−10)2−4⋅1⋅(−17)2⋅1
Calculating the discriminant:
√100+68=√168=2√42
Thus, the roots are:
|z|=10±2√422=5±√42
Step 6: Analyzing the quadratic inequality
The quadratic |z|2−10|z|−17<0 opens upwards (since the coefficient of |z|2 is positive). Therefore, the solution to the inequality is between the roots:
5−√42<|z|<5+√42
Step 7: Interpreting the result
Since |z| represents the modulus of the complex number z, the locus of z is the region between two circles centered at the origin with radii 5−√42 and 5+√42.
Final Result
The locus of z is the interior of the annular region between the circles of radius 5−√42 and 5+√42.
---
We start with the inequality:
log√3∣∣
∣∣|z|2−|z|+1|z|+2∣∣
∣∣<2
Taking the antilogarithm, we have:
∣∣
∣∣|z|2−|z|+1|z|+2∣∣
∣∣<32
which simplifies to:
∣∣
∣∣|z|2−|z|+1|z|+2∣∣
∣∣<9
Since both the numerator and denominator are positive for all |z|≥0, we can drop the absolute value:
|z|2−|z|+1|z|+2<9
Cross-multiplying gives:
|z|2−|z|+1<9(|z|+2)
Expanding the right side:
|z|2−|z|+1<9|z|+18
Rearranging the terms leads to:
|z|2−10|z|−17<0
To solve the quadratic inequality, we first find the roots of the equation:
|z|2−10|z|−17=0
Using the quadratic formula:
|z|=−b±√b2−4ac2a=10±√(−10)2−4⋅1⋅(−17)2⋅1
Calculating the discriminant:
√100+68=√168=2√42
Thus, the roots are:
|z|=10±2√422=5±√42
The quadratic |z|2−10|z|−17<0 opens upwards (since the coefficient of |z|2 is positive). Therefore, the solution to the inequality is between the roots:
5−√42<|z|<5+√42
Since |z| represents the modulus of the complex number z, the locus of z is the region between two circles centered at the origin with radii 5−√42 and 5+√42.
The locus of z is the interior of the annular region between the circles of radius 5−√42 and 5+√42.
Similar Questions
If (log)√3(|z|2−|z|+12+|z|)>2, then locate the region in the Argand plane which represents z.
View SolutionIf log √3(|z|2−|z|+12+|z|)>2, then the locus of z is
View SolutionIf logtan30∘[2|z|2+2|z|−3|z|+1]<−2 then |z|=
View SolutionThe pressure-volume of various thermodynamic process is shown in graphs:
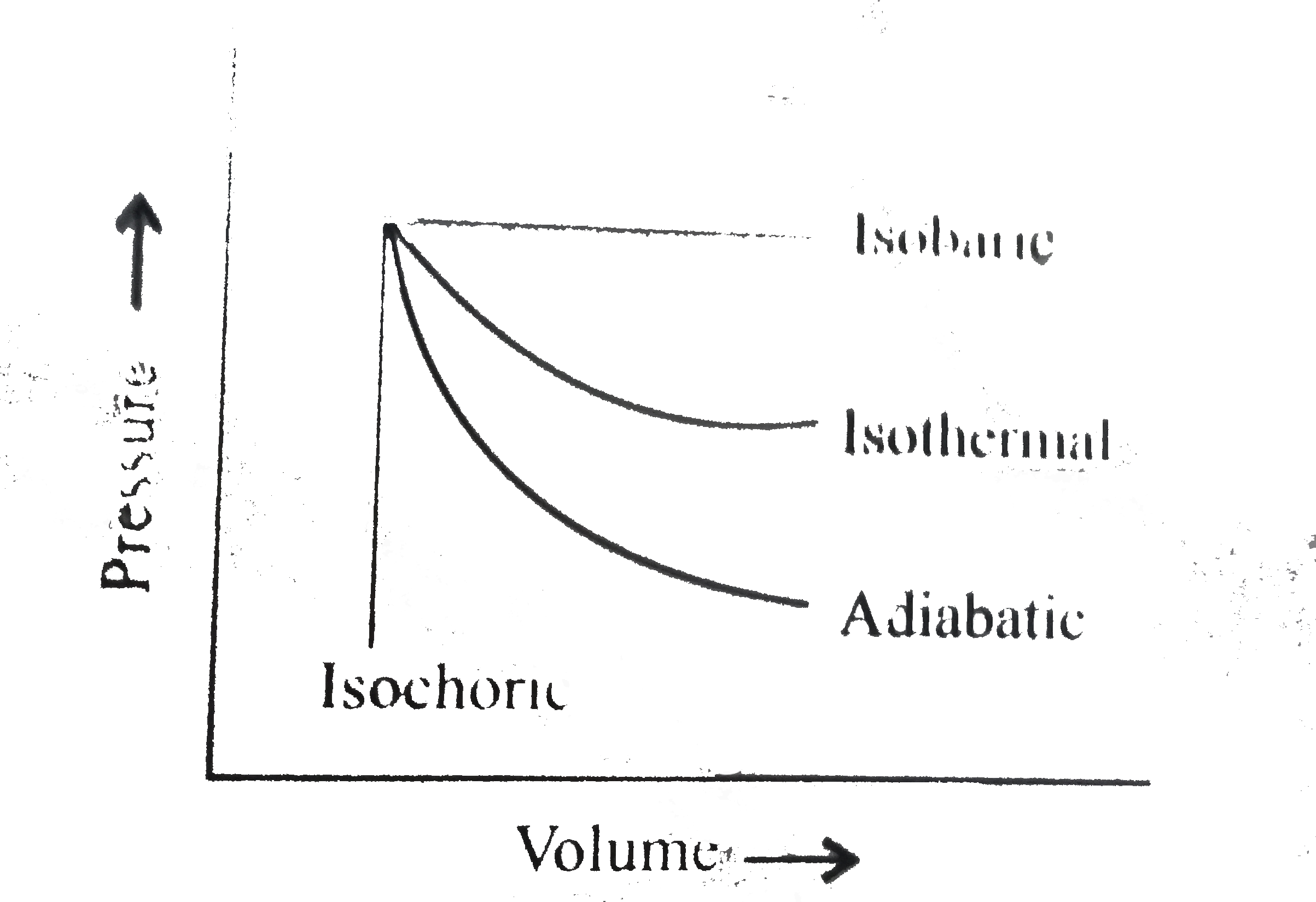
Work is the mole of transference of energy. It has been observed that reversible work done by the system is the maximum obtainable work.
wrev>wirr
The works of isothermal and adiabatic processes are different from each other.
wisothermal reversible=2.303nRTlog10(V2V1)
=2.303nRTlog10(P2P1)
wadiabatic reversible=CV(T1−T2)
A thermodynamic system goes in a cyclic process as represented in the following P−V diagram:
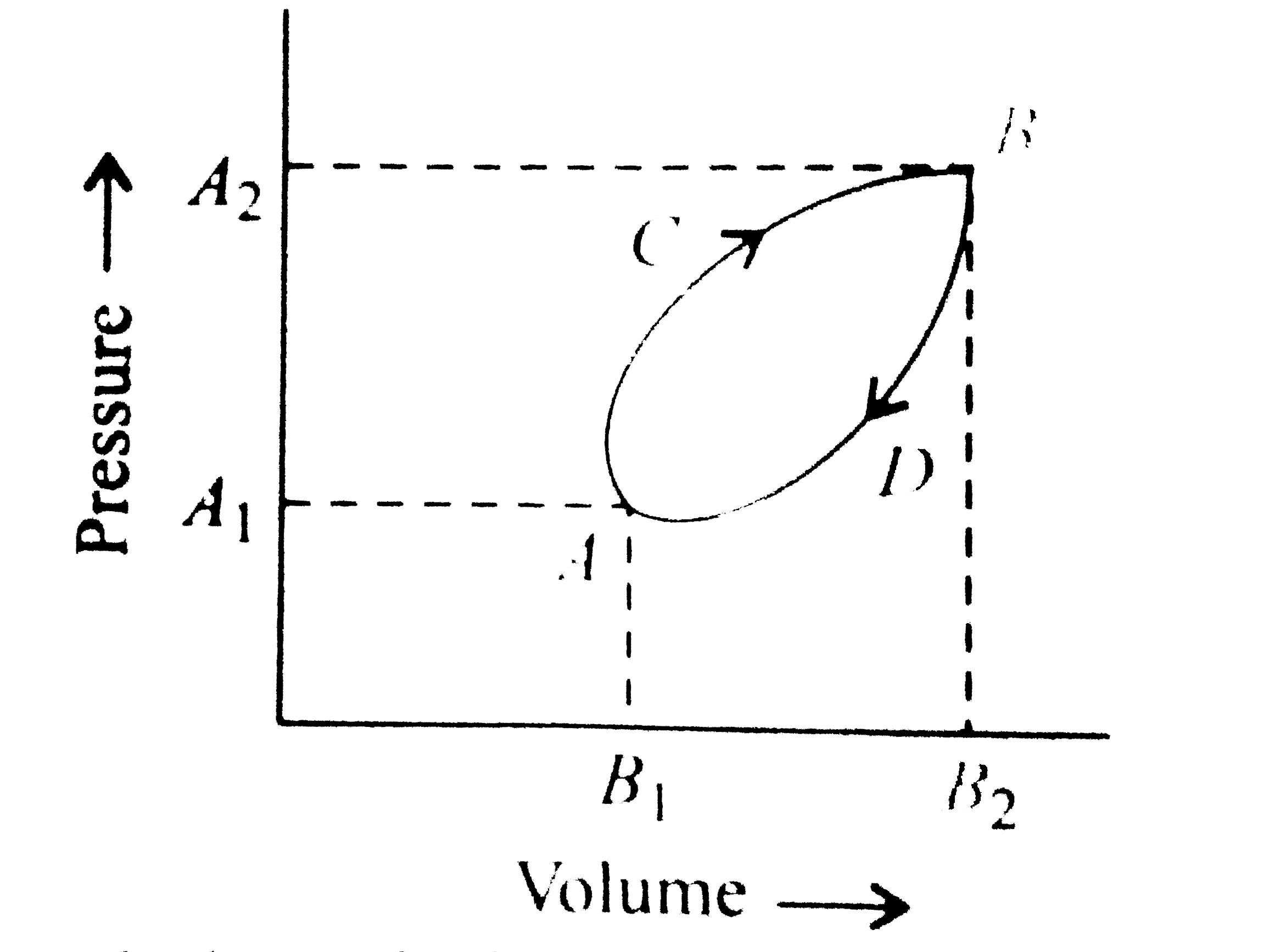
The net work done during the complete cycle is given by the area
View SolutionIf z=ilog(2−√3) then cosz
View SolutionIf log13|z+1|>log13|z−1| then prove that Re(z) < 0.
View SolutionIf arg ⎛⎜⎝z1−z|z|z|z|⎞⎟⎠=π2and∣∣∣z|z|−z1∣∣∣=3, then |z1| equals to a. √3 b. 2√2 c. √10 d. √26
View SolutionIf z=ilog(2−√3), then cosz= a.−1 b. −12 c. 1 d. 2
View Solution∫dzz√z2−1
View SolutionIf |z1|=√2,|z2|=√3and|z1+z2|=√(5−2√3) then arg (z1z2) (not neccessarily principal) is (a) 3π4 (b) 2π3 (c) 5π4 (d) 5π2
View Solution