If log √3(|z|2−|z|+12+|z|)>2, then the locus of z is
|z|=5
|z|lt 5
|z| gt5
|z|=0
The correct Answer is:B
To solve the inequality log√3(|z|2−|z|+12+|z|)>2, we will follow these steps:
Step 1: Rewrite the logarithmic inequality
We start with the given inequality:
log√3(|z|2−|z|+12+|z|)>2
To eliminate the logarithm, we can use the property of logarithms:
|z|2−|z|+12+|z|>(√3)2
Since (√3)2=3, we rewrite the inequality as:
|z|2−|z|+12+|z|>3
Step 2: Cross-multiply to eliminate the fraction
Cross-multiplying gives us:
|z|2−|z|+1>3(2+|z|)
This simplifies to:
|z|2−|z|+1>6+3|z|
Step 3: Rearrange the inequality
Rearranging the terms leads to:
|z|2−|z|−3|z|+1−6>0
This simplifies to:
|z|2−4|z|−5>0
Step 4: Factor the quadratic expression
Now we can factor the quadratic:
|z|2−4|z|−5=(|z|−5)(|z|+1)
Thus, the inequality becomes:
(|z|−5)(|z|+1)>0
Step 5: Determine the intervals
To find the intervals where this product is positive, we need to consider the roots:
1. |z|−5=0 gives |z|=5
2. |z|+1=0 gives |z|=−1 (not applicable since modulus cannot be negative)
The critical points divide the number line into intervals:
- |z|<−1 (not applicable)
- −1<|z|<5
- |z|>5
Step 6: Test the intervals
We test the intervals:
1. For |z|<5: Choose |z|=0 → (0−5)(0+1)<0 (not valid)
2. For |z|>5: Choose |z|=6 → (6−5)(6+1)>0 (valid)
Conclusion
The solution to the inequality is:
|z|>5
This means the locus of z is outside the circle of radius 5 centered at the origin in the complex plane.
---
We start with the given inequality:
log√3(|z|2−|z|+12+|z|)>2
To eliminate the logarithm, we can use the property of logarithms:
|z|2−|z|+12+|z|>(√3)2
Since (√3)2=3, we rewrite the inequality as:
|z|2−|z|+12+|z|>3
Cross-multiplying gives us:
|z|2−|z|+1>3(2+|z|)
This simplifies to:
|z|2−|z|+1>6+3|z|
Rearranging the terms leads to:
|z|2−|z|−3|z|+1−6>0
This simplifies to:
|z|2−4|z|−5>0
Now we can factor the quadratic:
|z|2−4|z|−5=(|z|−5)(|z|+1)
Thus, the inequality becomes:
(|z|−5)(|z|+1)>0
To find the intervals where this product is positive, we need to consider the roots:
1. |z|−5=0 gives |z|=5
2. |z|+1=0 gives |z|=−1 (not applicable since modulus cannot be negative)
- |z|<−1 (not applicable)
- −1<|z|<5
- |z|>5
We test the intervals:
1. For |z|<5: Choose |z|=0 → (0−5)(0+1)<0 (not valid)
2. For |z|>5: Choose |z|=6 → (6−5)(6+1)>0 (valid)
The solution to the inequality is:
|z|>5
This means the locus of z is outside the circle of radius 5 centered at the origin in the complex plane.
Topper's Solved these Questions
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - Assignment (Section -C) (objective Type Questions ( more thena one options are correct )
35 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - Assignment (Section -D) Linked comprehension Type Questions
14 Videos
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - Assignment (Section -A) (objective Type Questions ( one option is correct)
47 Videos
- BINOMIAL THEOREMBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - BINOMIAL THEOREMEXERCISE - Assignment (section-J) Objective type question (Aakash Challengers Questions)
4 Videos
- CONIC SECTIONSBOOK - AAKASH INSTITUTE ENGLISHCHAPTER - CONIC SECTIONSEXERCISE - SECTION - J ( Aakash Challengers Questions )
16 Videos
Similar Questions
If log√3∣∣ ∣∣|z|2−|z|+1∣∣|z|+2∣∣ ∣∣<2 then locus of z is
View SolutionIf (log)√3(|z|2−|z|+12+|z|)>2, then locate the region in the Argand plane which represents z.
View SolutionIf Imz(z−12z+1)=−4, then locus of z is
View SolutionIf Im2z+1iz+1=−2, then locus of z, is
View SolutionThe pressure-volume of various thermodynamic process is shown in graphs:
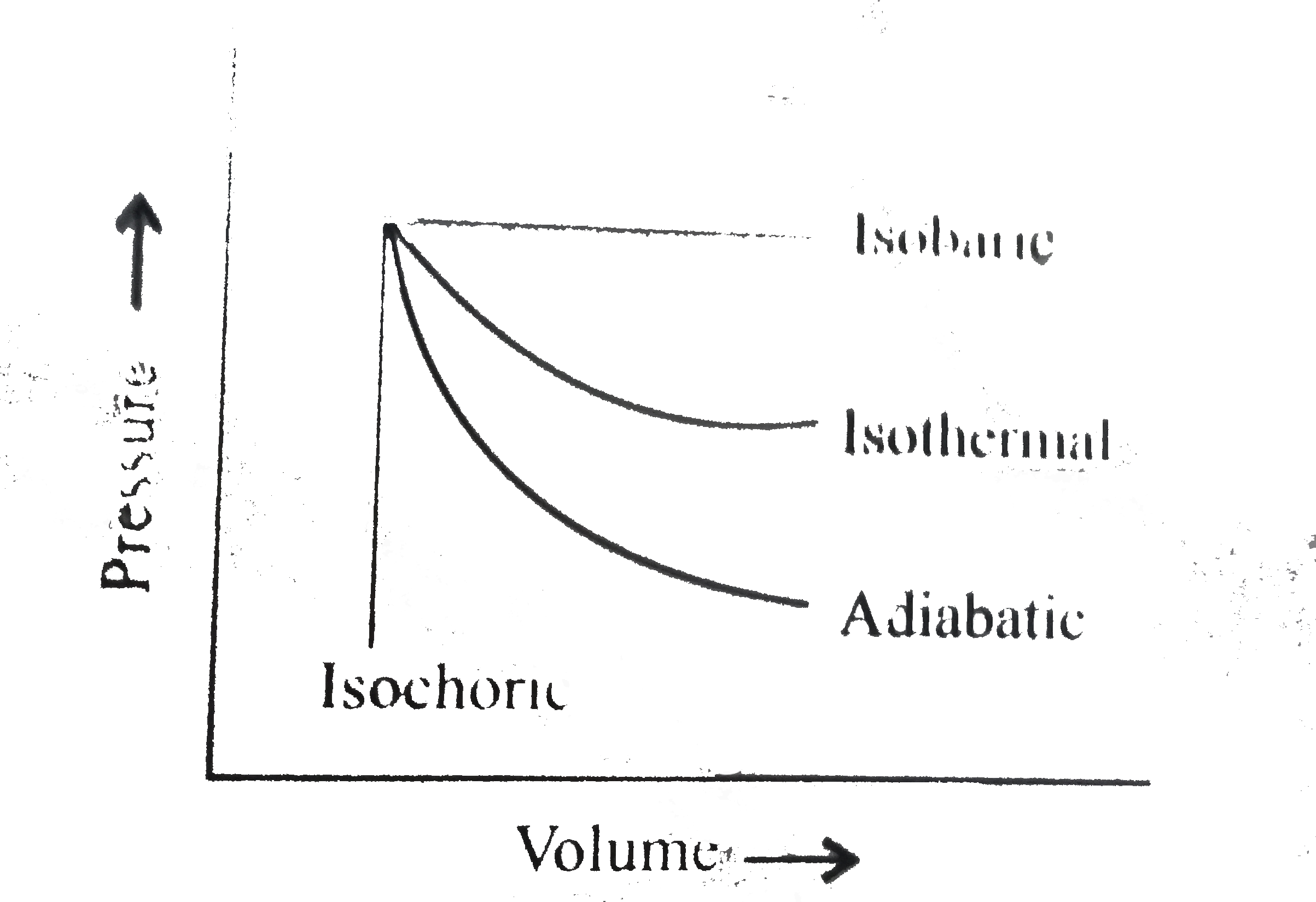
Work is the mole of transference of energy. It has been observed that reversible work done by the system is the maximum obtainable work.
wrev>wirr
The works of isothermal and adiabatic processes are different from each other.
wisothermal reversible=2.303nRTlog10(V2V1)
=2.303nRTlog10(P2P1)
wadiabatic reversible=CV(T1−T2)
A thermodynamic system goes in a cyclic process as represented in the following P−V diagram:
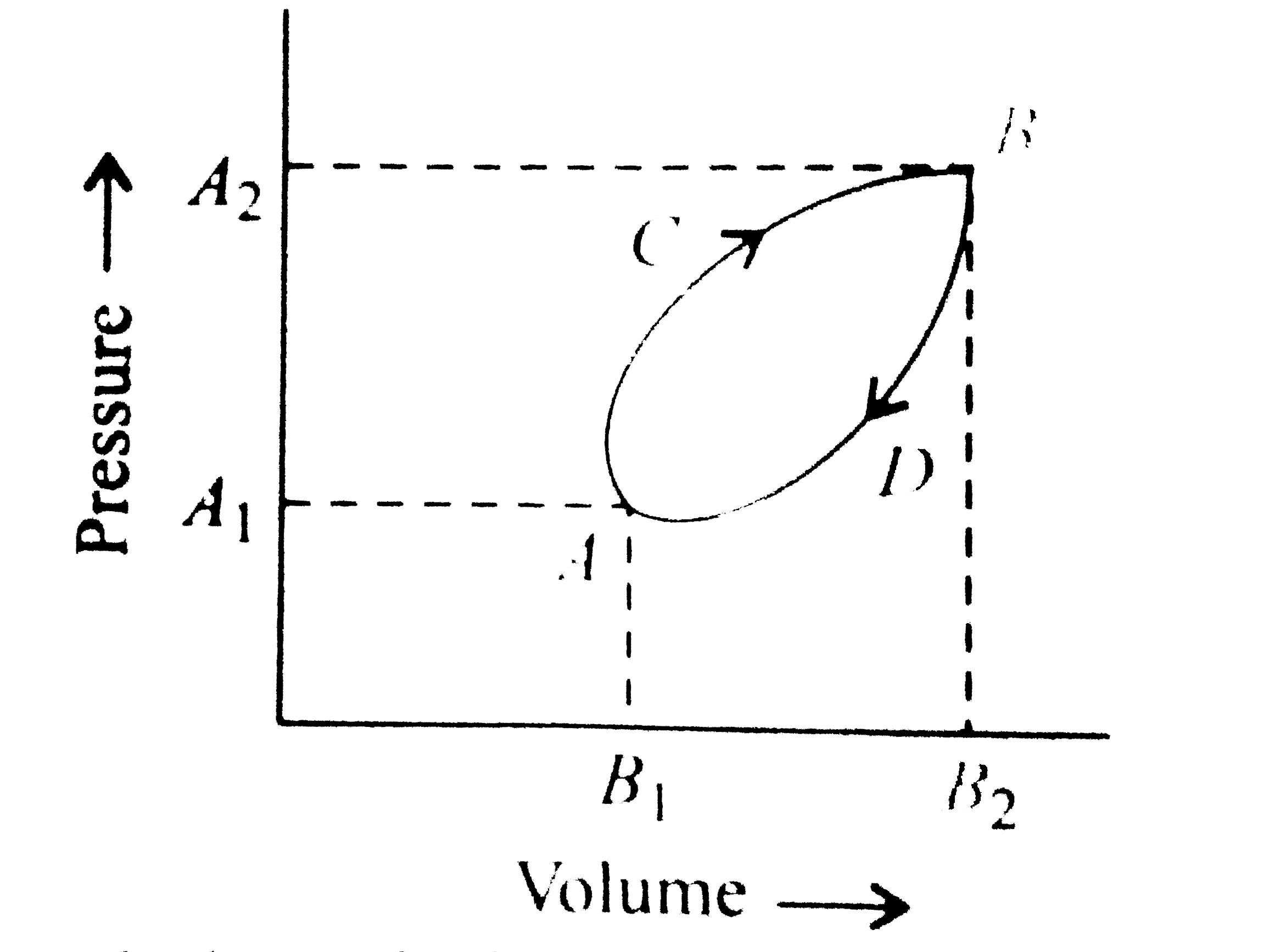
The net work done during the complete cycle is given by the area
View SolutionIf logtan30∘[2|z|2+2|z|−3|z|+1]<−2 then |z|=
View SolutionIf z=x+iy is a complex number satisfying ∣∣∣z+i2∣∣∣2=∣∣∣z−i2∣∣∣2 , then the locus of z is
View SolutionIf z=x+iy and real part (z−12z+i)=1 then locus of z is
View SolutionStatement-1 : The locus of z , if arg(z−1z+1)=π2 is a circle.
and
Statement -2 : ∣∣∣z−2z+2∣∣∣=π2, then the locus of z is a circle.
View SolutionIf |z|=1 and let ω=(1−z)21−z2 , then prove that the locus of ω is equivalent to |z−2|=|z+2|
View Solution
