Find the time required for a cylindrical tank of radius r and height H to empty through a round hole of area a at the bottom. The flow through the hole is according to the law v(t)=k√2gh(t) , where v(t) and h(t) , are respectively, the velocity of flow through the hole and the height of the water level above the hole at time t, and g is the acceleration due to gravity.
To find the time required for a cylindrical tank of radius r and height H to empty through a round hole of area a at the bottom, we will use the principles of fluid dynamics and differential equations. The velocity of flow through the hole is given by the equation:
v(t)=k√2gh(t)
where h(t) is the height of the water level above the hole at time t, and g is the acceleration due to gravity.
1. Set Up the Problem:
- The volume of water in the cylindrical tank is given by:
V=πr2h
- The rate of change of volume with respect to time is:
dVdt=ddt(πr2h)=πr2dhdt
2. Flow Rate Out of the Hole:
- The flow rate Q through the hole is given by:
Q=Av(t)=Ak√2gh(t)
- Since the water is flowing out, we have:
dVdt=−Q
- Therefore, we can write:
πr2dhdt=−Ak√2gh
3. Rearranging the Equation:
- Rearranging gives:
dhdt=−Akπr2√2gh
4. Separating Variables:
- We can separate the variables h and t:
dh√h=−Ak√2gπr2dt
5. Integrating Both Sides:
- Integrate both sides:
∫dh√h=−Ak√2gπr2∫dt
- The left side integrates to:
2√h=−Ak√2gπr2t+C
6. Finding the Constant of Integration:
- At t=0, h=H:
2√H=C
- Thus, we have:
2√h=−Ak√2gπr2t+2√H
7. Solving for Time t:
- Rearranging gives:
t=−πr2Ak√2g(2√h−2√H)
8. Finding the Time to Empty the Tank:
- When the tank is empty, h=0:
t=−πr2Ak√2g(2√0−2√H)=2πr2√HAk√2g
Final Result:
The time required for the cylindrical tank to empty is given by:
t=2πr2√HAk√2g
- The volume of water in the cylindrical tank is given by:
V=πr2h
- The rate of change of volume with respect to time is:
dVdt=ddt(πr2h)=πr2dhdt
- The flow rate Q through the hole is given by:
Q=Av(t)=Ak√2gh(t)
- Since the water is flowing out, we have:
dVdt=−Q
- Therefore, we can write:
πr2dhdt=−Ak√2gh
- Rearranging gives:
dhdt=−Akπr2√2gh
- We can separate the variables h and t:
dh√h=−Ak√2gπr2dt
- Integrate both sides:
∫dh√h=−Ak√2gπr2∫dt
- The left side integrates to:
2√h=−Ak√2gπr2t+C
- At t=0, h=H:
2√H=C
- Thus, we have:
2√h=−Ak√2gπr2t+2√H
- Rearranging gives:
t=−πr2Ak√2g(2√h−2√H)
- When the tank is empty, h=0:
t=−πr2Ak√2g(2√0−2√H)=2πr2√HAk√2g
The time required for the cylindrical tank to empty is given by:
t=2πr2√HAk√2g
Similar Questions
A hemi-spherical tank of radius 2 m is initially full of water and has an outlet of 12cm2 cross-sectional area at the bottom. The outlet is opened at some instant. The flow through the outlet is according to the law v(t)=0.6√2gh(t), where v(t) and h(t) are, respectively, the velocity of the flow through the outlet and the height of water level above the outlet and the height of water level above the outlet at time t, and g is the acceleration due to gravity. Find the time it takes to empty the tank.
View SolutionFind the time required for a cylindrical tank of radius 2.5 m and height 3 m to empty through a round hole of 2.5 cm with a velocity 2.5√h m/s, h being the depth of the water in the tank.
View SolutionFind the time required for a cylindrical tank of radius 2.5 m and height 3 m to empty through a round hole of radius 2.5 cm with a velocity 2.5√h m/s, h being the depth of the water in the tank.
View SolutionA large tank filled with water to a height h is to be emptied through a small hole at the bottom. The ratio of times taken for the level of water to fall from h to h2 and from h2 to zero is
View SolutionA water tank has a hole at a distance of 7 m from free water surface. Find the velocity of water through the hole. If the radius of the hole is 2 mm what is the rate of flow of water?
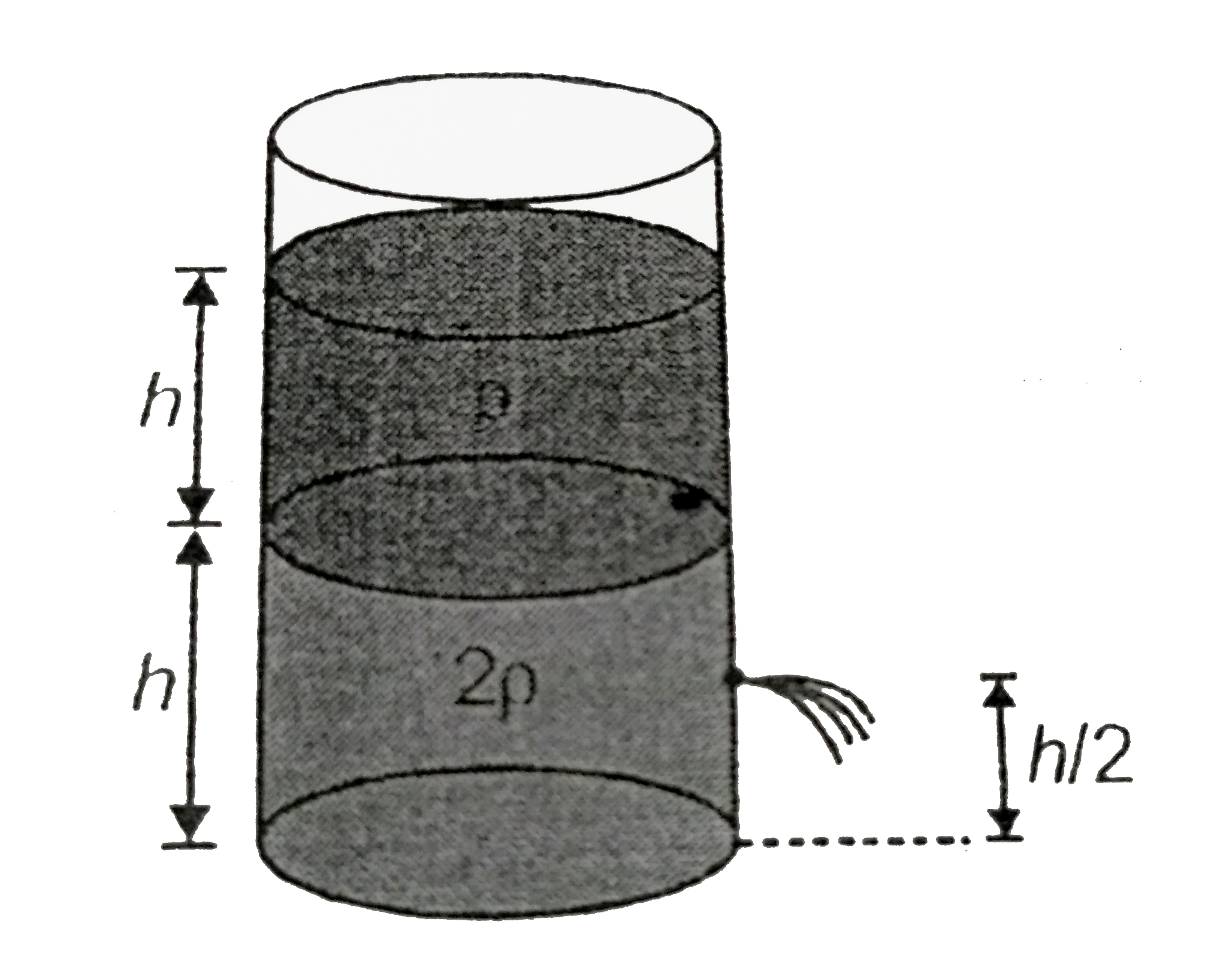
View SolutionA vessel is filled with two different liquids of densities ρand2ρ respectively as shown in the figure. The velocity of flow of liquid through a hole at height h2 from bottom is
View SolutionThe contribution in the total current flowing through a semiconductor due to electrons and holes are 34 and 14 respectively. If the drift velocity of electrons is 52 times that of holes at this temperature, then the ratio of concentration of electrons and holes is
View SolutionWater and mercury are filled in two cylindrical vessels up to same height. Both vessels have a hole in the wall near the bottom. The velocity of water and mercury coming out of the holes are v1andv2 respectively. Then
View SolutionA body is projected veritclaly upwards.The times corresponding to height h while ascending and while descending are t1 and t2 respectively.
Then, the velocity of projection will be (take g as acceleration due to gravity)
View SolutionA body is projected vertically upwatds at time t=0 and is it seen at a height H at time t1 and t2 second during its flight. The maximum height attainet is (g is acceleration due to garavity).
View Solution
