Lines L1≡ax+by+c=0 and L2≡lx+my+n=0 intersect at the point P and make an angle θ with each other. Find the equation of a line different from L2 which passes through P and makes the same angle θ with L1.
To find the equation of a line L that is different from L2, passes through the intersection point P of lines L1 and L2, and makes the same angle θ with L1, we can follow these steps:
1. Identify the Given Lines:
The equations of the lines are given as:
L1:Ax+By+C=0
L2:Lx+My+N=0
2. Equation of the Required Line:
Since the required line L passes through the intersection of L1 and L2, we can express its equation as a linear combination of L1 and L2:
L:Ax+By+C+λ(Lx+My+N)=0
Here, λ is a parameter.
3. Using the Angle Bisector Theorem:
The line L will be an angle bisector of the angles formed by L1 and L2. Therefore, the angle between L1 and L will be the same as the angle between L1 and L2.
4. Equidistance Condition:
For any point (x1,y1) on L, the distance from this point to L1 and L2 must be equal. This gives us the condition:
Ax1+By1+C√A2+B2=Lx1+My1+N√L2+M2
5. Substituting the Point Condition:
Since (x1,y1) lies on L1, we have:
Ax1+By1+C=0
Substituting this into the equidistance condition gives:
0=Lx1+My1+N√L2+M2
6. Solving for λ:
From the equidistance condition, we can derive a relationship involving λ:
Ax1+By1+C+λ(Lx1+My1+N)√A2+B2=0
This leads to:
λ=−A2+B22AL+BM
7. Substituting λ Back:
Substitute the value of λ back into the equation of line L:
L:Ax+By+C−A2+B22AL+BM(Lx+My+N)=0
8. Final Equation:
Rearranging gives us the final equation of the line L:
(2AL+BM)(Ax+By+C)−(A2+B2)(Lx+My+N)=0
The equations of the lines are given as:
L1:Ax+By+C=0
L2:Lx+My+N=0
Since the required line L passes through the intersection of L1 and L2, we can express its equation as a linear combination of L1 and L2:
L:Ax+By+C+λ(Lx+My+N)=0
Here, λ is a parameter.
The line L will be an angle bisector of the angles formed by L1 and L2. Therefore, the angle between L1 and L will be the same as the angle between L1 and L2.
For any point (x1,y1) on L, the distance from this point to L1 and L2 must be equal. This gives us the condition:
Ax1+By1+C√A2+B2=Lx1+My1+N√L2+M2
Since (x1,y1) lies on L1, we have:
Ax1+By1+C=0
Substituting this into the equidistance condition gives:
0=Lx1+My1+N√L2+M2
From the equidistance condition, we can derive a relationship involving λ:
Ax1+By1+C+λ(Lx1+My1+N)√A2+B2=0
This leads to:
λ=−A2+B22AL+BM
Substitute the value of λ back into the equation of line L:
L:Ax+By+C−A2+B22AL+BM(Lx+My+N)=0
Rearranging gives us the final equation of the line L:
(2AL+BM)(Ax+By+C)−(A2+B2)(Lx+My+N)=0
Topper's Solved these Questions
Similar Questions
lines L1:ax+by+c=0 and L2:lx+my+n=0 intersect at the point P and make a angle θ between each other. find the equation of a line Ldifferent from L2 which passes through P and makes the same angle θ with L1
View SolutionFind the equation of the straight line through the point (α,β) and perpendiculasr to the line lx+my+n=0.
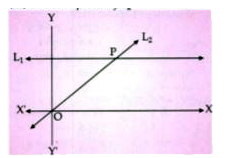
View SolutionGiven equation of line L1 is y=4
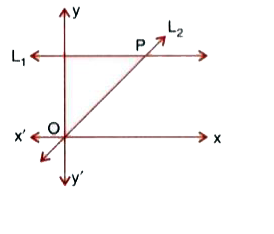
Write the slope of line L1 if L2 is the bisector of angle O.
View SolutionTwo lines l and m interset at the O and P is Point on a line n Passing through the point O such that P is equidistant from l and m. Prove that n is the bisectof the angle formed by l and m
View SolutionThe equation of line l1 is y=2x+3, and the equation of line l2 is y=2x-5.
View Solutionthe lines L1 and L2 denoted by 3x2+10xy+8y2+14x+22y+15=0 intersect at the point P and have gradients M1 and M2 respectively.The acute angles between them is θ . which of the following relations hold good ?
View SolutionThe direction cosines of the lines bisecting the angle between the line whose direction cosines are l1,m1,n1andl2,m2,n2 and the angle between these lines is θ, are
View SolutionGiven equation of line L1 is y = 4 and line L2 is the bisector of angle O.
Write the coordinates of point P.
View SolutionIn Fig. 30, lines l1 and l2 intersect at O, forming angles as shown in the figure. If x=450, find the values of y,z and u.
View SolutionA variable line passing through the point P(0, 0, 2) always makes angle 60∘ with z-axis, intersects the plane x+y+z=1. Find the locus of point of intersection of the line and the plane.
View Solution
