Question

Two lines l and m interset at the O and P is Point on a line n Passing through the point O such that P is equidistant from l and m. Prove that n is the bisectof the angle formed by l and m

Explanation

Given two lines l and m intersect at the point O and P is a point on a line n Passing though O such that P is equidistant from l and m i.e PQ=PR

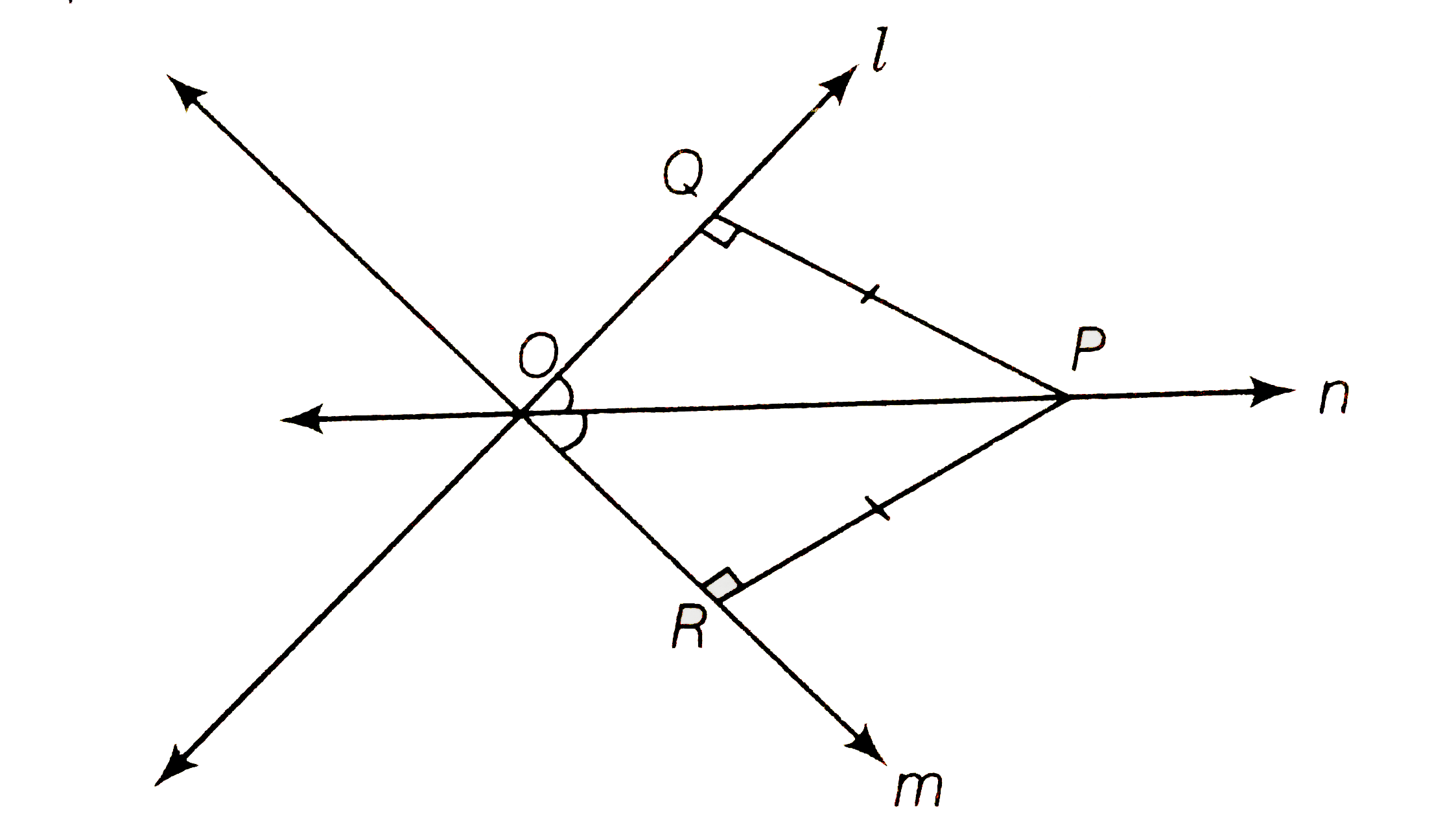
To prove n is the bisector of the angle formed by l and m i.e n is the bisector of `angle QOR`.
Proof in `Delta OQP and Delta ORP`
`angle PQO=angle PRO=90^(@)`
[since P in equlidistant from l and m so PQ and PR should be Perpendicular to lines l and m repectively ]
OP =OP [Common side ]
PQ=PR [given ]
`therefore Delta OQP cong Delta ORP ` [by RHS congruence rule ]
`rArr angle POQ =angle POR ` [by CPCT]
Hence ,n is the bisector of `angle QOR`