ABCD is a rectangle. A circle passing through vertex C touches the sides AB and AD at M and N respectively. If the distance lof the line MN from the vertex C is P units then the area of rectangle ABCD is
To solve the problem, we need to find the area of rectangle ABCD given that a circle passing through vertex C touches sides AB and AD at points M and N respectively, and the distance from the line MN to vertex C is P units.
1. Understanding the Geometry:
- We have a rectangle ABCD.
- The circle passes through point C and touches side AB at point M and side AD at point N.
- The distance from point C to the line MN is given as P units.
2. Identifying Angles:
- Since MN is tangent to the circle at points M and N, the angles formed at points M and N with respect to line segments CM and CN are both 90 degrees.
- Therefore, we can denote the angles as follows:
- Angle CMP = 90 degrees
- Angle CNP = 90 degrees
3. Using Triangle Similarity:
- By the properties of tangents and the rectangle, we can establish relationships using triangle similarity.
- From triangles formed by the points C, M, and N, we can set up the following ratios based on similar triangles:
- CDCP=DNPM=CNCM (Equation 1)
- From triangles CBM and CBN, we can also establish:
- CBCB=BMBN=CMCN (Equation 2)
4. Equating Ratios:
- From Equation 1 and Equation 2, we can relate the segments:
- CDCP=CMCN
- By cross-multiplying, we obtain:
- CP2=CD⋅CB
5. Substituting Known Values:
- We know that CP=P (given in the problem).
- Therefore, substituting this into our equation gives:
- P2=CD⋅CB
6. Finding the Area of Rectangle ABCD:
- The area of rectangle ABCD is given by the product of its length and width:
- Area = CB⋅CD
- From our previous step, we have:
- P2=Area
7. Conclusion:
- Thus, the area of rectangle ABCD is P2.
Final Answer:
The area of rectangle ABCD is P2.
---
- We have a rectangle ABCD.
- The circle passes through point C and touches side AB at point M and side AD at point N.
- The distance from point C to the line MN is given as P units.
- Since MN is tangent to the circle at points M and N, the angles formed at points M and N with respect to line segments CM and CN are both 90 degrees.
- Therefore, we can denote the angles as follows:
- Angle CMP = 90 degrees
- Angle CNP = 90 degrees
- By the properties of tangents and the rectangle, we can establish relationships using triangle similarity.
- From triangles formed by the points C, M, and N, we can set up the following ratios based on similar triangles:
- CDCP=DNPM=CNCM (Equation 1)
- From triangles CBM and CBN, we can also establish:
- CBCB=BMBN=CMCN (Equation 2)
- From Equation 1 and Equation 2, we can relate the segments:
- CDCP=CMCN
- By cross-multiplying, we obtain:
- CP2=CD⋅CB
- We know that CP=P (given in the problem).
- Therefore, substituting this into our equation gives:
- P2=CD⋅CB
- The area of rectangle ABCD is given by the product of its length and width:
- Area = CB⋅CD
- From our previous step, we have:
- P2=Area
- Thus, the area of rectangle ABCD is P2.
The area of rectangle ABCD is P2.
Topper's Solved these Questions
- COMPLEX NUMBERS AND QUADRATIC EQUATIONSBOOK - CENGAGE ENGLISHCHAPTER - COMPLEX NUMBERS AND QUADRATIC EQUATIONSEXERCISE - All Questions
886 Videos
- DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS BOOK - CENGAGE ENGLISHCHAPTER - DIFFERENT PRODUCTS OF VECTORS AND THEIR GEOMETRICAL APPLICATIONS EXERCISE - Multiple correct answers type
11 Videos
Similar Questions
A circle passing through the vertex C of a rectangle ABCD and touching its sides AB and AD at M and N, respectively. If the distance from C to the line segment MN is equal to 5 units, then find the area of the reactangle ABCD.
View SolutionABCD is a rectangle and P, Q, R and S are mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rhombus.
View Solution
Knowledge Check
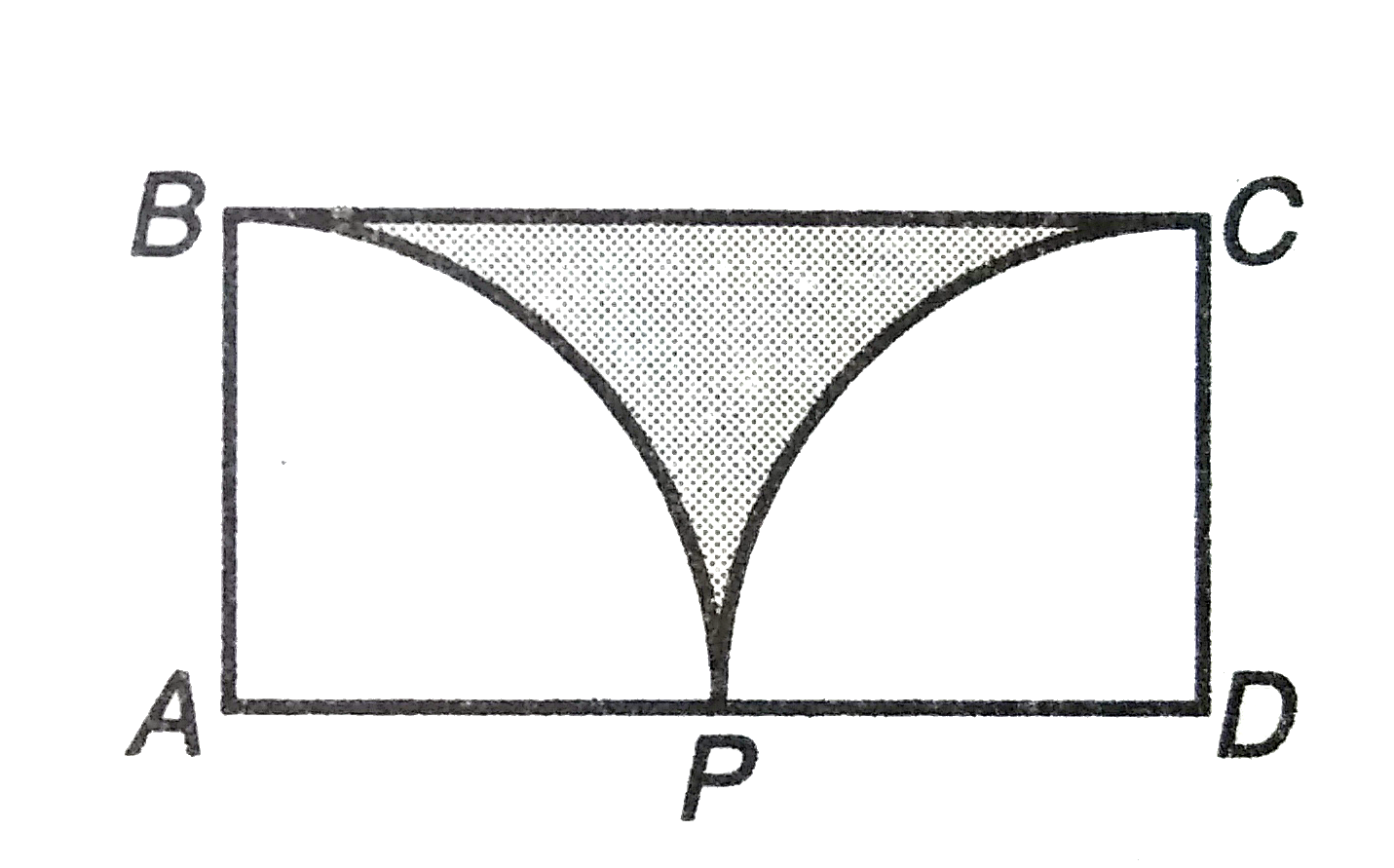
In rectangle ABCD above, arcs BP and CP are quarter circles with centers at points A and D, respectively. If the area of each quarter circle is π, what is the area of the shaded region?A4−π2B4−πC8−πD8−2π
ABC is a right triangle, right - angled at the vertex A. A circle is drawn to touch the sides AB and AC at points P and Q respectively such that the other end points of the diameters passing through P and Q lie on the side BC. IF AB = 6 units, then the area (in sq. units) of the circular sector which lies inside the triangle is
View SolutionABCD is a parallelogram . The circle passing through the vertices. A, B and C intersects CD (or CD produced) at E. Prove that AE=AD.
View SolutionLet ABCD be a rectangle such that AB=10 units and BC=8 units. Taking AB and AD as x and y axis respectively, find the coordinates of A, B, C and D.
View SolutionP is a point inside the triangle ABC. Lines are drawn through P, parallel to the sides of the triangle.The three resulting triangles with the vertex at P have areas 4,9and49sq. units. The area of the triangle ABC is -
View SolutionA circle touches a rectangle ABCD of side lengths 2a and 2b at M and N on sides AB and AD respectively , it also passes through the point C. If perpendicular distance of the line MN from points C is 6 cm. Then value of ab is ____
View SolutionABCD is a square of side length 2 units. C1 is the circle touching all the sides of the square ABCD and C2 is the circumcircle of square ABCD. L is a fixed line in the same plane and R is fixed point. If a circle is such that it touches the line L and the circle C1 externally, such that both the circles are on the same side of the line, then the locus of centre of the circle is
View SolutionABCD is a rhombus and P, Q, R and S are wthe mid-points of the sides AB, BC, CD and DA respectively. Show that the quadrilateral PQRS is a rectangle.
View Solution
