Find the angel between any two diagonals of a cube.
To find the angle between any two diagonals of a cube, we can follow these steps:
Step 1: Define the cube and its vertices
Let’s consider a cube with vertices defined in a 3D coordinate system. We can place one vertex at the origin (0, 0, 0) and the other vertices at the following coordinates, assuming the side length of the cube is 'a':
- A (0, 0, 0)
- B (a, 0, 0)
- C (a, a, 0)
- D (0, a, 0)
- E (0, 0, a)
- F (a, 0, a)
- G (a, a, a)
- H (0, a, a)
Step 2: Identify the diagonals
We will consider the diagonals O-G (from A to G) and A-D (from A to D). The coordinates for these points are:
- O (0, 0, 0)
- G (a, a, a)
- D (0, a, 0)
Step 3: Find the direction vectors of the diagonals
To find the angle between the two diagonals, we first need to determine their direction vectors.
1. Vector OG:
Vector OG=G−O=(a,a,a)−(0,0,0)=(a,a,a)
2. Vector AD:
Vector AD=D−A=(0,a,0)−(0,0,0)=(0,a,0)
Step 4: Calculate the dot product of the vectors
The dot product of two vectors u=(u1,u2,u3) and v=(v1,v2,v3) is given by:
u⋅v=u1v1+u2v2+u3v3
For our vectors:
OG⋅AD=(a,a,a)⋅(0,a,0)=0⋅a+a⋅a+a⋅0=a2
Step 5: Find the magnitudes of the vectors
The magnitude of a vector u=(u1,u2,u3) is given by:
|u|=√u21+u22+u23
1. Magnitude of OG:
|OG|=√a2+a2+a2=√3a2=a√3
2. Magnitude of AD:
|AD|=√02+a2+02=√a2=a
Step 6: Use the dot product to find the cosine of the angle
The cosine of the angle θ between the two vectors is given by:
cosθ=OG⋅AD|OG||AD|
Substituting the values we found:
cosθ=a2(a√3)(a)=a23a2=13
Step 7: Find the angle θ
To find the angle θ:
θ=cos−1(13)
Conclusion
The angle between any two diagonals of a cube is θ=cos−1(13).
---
Let’s consider a cube with vertices defined in a 3D coordinate system. We can place one vertex at the origin (0, 0, 0) and the other vertices at the following coordinates, assuming the side length of the cube is 'a':
- A (0, 0, 0)
- B (a, 0, 0)
- C (a, a, 0)
- D (0, a, 0)
- E (0, 0, a)
- F (a, 0, a)
- G (a, a, a)
- H (0, a, a)
We will consider the diagonals O-G (from A to G) and A-D (from A to D). The coordinates for these points are:
- O (0, 0, 0)
- G (a, a, a)
- D (0, a, 0)
To find the angle between the two diagonals, we first need to determine their direction vectors.
Vector OG=G−O=(a,a,a)−(0,0,0)=(a,a,a)
Vector AD=D−A=(0,a,0)−(0,0,0)=(0,a,0)
The dot product of two vectors u=(u1,u2,u3) and v=(v1,v2,v3) is given by:
u⋅v=u1v1+u2v2+u3v3
OG⋅AD=(a,a,a)⋅(0,a,0)=0⋅a+a⋅a+a⋅0=a2
The magnitude of a vector u=(u1,u2,u3) is given by:
|u|=√u21+u22+u23
|OG|=√a2+a2+a2=√3a2=a√3
|AD|=√02+a2+02=√a2=a
The cosine of the angle θ between the two vectors is given by:
cosθ=OG⋅AD|OG||AD|
Substituting the values we found:
cosθ=a2(a√3)(a)=a23a2=13
To find the angle θ:
θ=cos−1(13)
The angle between any two diagonals of a cube is θ=cos−1(13).
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - CONCEPT APPLICATION EXERCISE 3.215videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - CONCEPT APPLICATION EXERCISE 3.319videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE ENGLISHCHAPTER - THREE-DIMENSIONAL GEOMETRY EXERCISE - ARCHIVES INTEGER TYPE1videos
THREE-DIMENSIONAL GEOMETRY
- BOOK - CENGAGE ENGLISHCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions294videos
THREE DIMENSIONAL GEOMETRY
- BOOK - CENGAGE ENGLISHCHAPTER - TRIGONOMETRIC EQUATIONSEXERCISE - Archives (Matrix Match Type)1videos
TRIGONOMETRIC EQUATIONS
Similar Questions
Find the angle between any two diagonals of a cube.
View Solution(i) Find the angle bewteen the lines whose direction ratios are 1, 2, 3 and - 3 , 2 , 1
(ii) Find the angle between two diagonals of a cube.View SolutionShow that the angle between two diagonals of a cube is cos−1√13.
View SolutionShow that the angle between two diagonals of a cube is cos−1(13).
View SolutionAn ellipse is inscribed in a reactangle and the angle between the diagonals of the reactangle is tan−1(2√2), then find the ecentricity of the ellipse
View SolutionThe shortest distance between a diagonal of a unit cube and the edge skew to it, is
View SolutionThe angle between diagonal of a cube and diagonal
of a face of the cube will beView SolutionThree metal cubes with edges 6cm , 8cm and 10cm respectively are melted together and formed in to a single cube. Find the diagonal of this cube.
View SolutionTwelve wires of equal resistacne R are connected to form a cube . The effective resistance between two diagonal ends A and E will be .
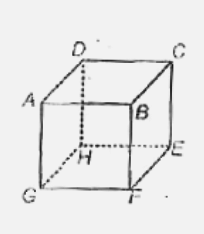 View Solution
View SolutionTwelve resistors of equal value R are connected in the form of a cube. Effective resistance between diagonals of the cube is
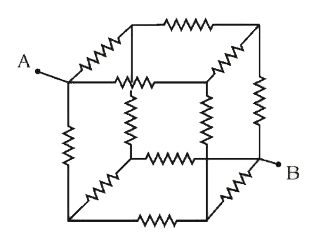 View Solution
View Solution

