Consider the following sets:
A= set ofnaturai numbers which are multiples of 2
B= set ofnaturai numbers which are multiples of 3
C= set ofnaturai numbers which are multiples of 5
Then find the following set
(i)A∪B(ii)B∪C(iii)A−B(iv)B−C(v)A∩C<br>(vi)A∩B∩C(vii)(A∪B)−C
To solve the problem, we will define the sets A, B, and C based on the given information and then perform the required set operations step by step.
Step 1: Define the Sets
- Set A: The set of natural numbers which are multiples of 2.
A={2,4,6,8,10,12,14,16,18,20,…}
- Set B: The set of natural numbers which are multiples of 3.
B={3,6,9,12,15,18,21,24,27,30,…}
- Set C: The set of natural numbers which are multiples of 5.
C={5,10,15,20,25,30,35,40,45,50,…}
Step 2: Find the Required Sets
(i) A∪B
This set contains all natural numbers that are multiples of 2 or 3.
- Result: A∪B={2,3,4,6,8,9,10,12,14,15,16,18,20,21,24,27,30,…}
(ii) B∪C
This set contains all natural numbers that are multiples of 3 or 5.
- Result: B∪C={3,5,6,9,10,12,15,18,20,21,24,25,30,35,40,…}
(iii) A−B
This set contains natural numbers that are multiples of 2 but not multiples of 3.
- Result: A−B={2,4,8,10,14,16,20,22,26,28,…}
(iv) B−C
This set contains natural numbers that are multiples of 3 but not multiples of 5.
- Result: B−C={3,6,9,12,18,21,24,27,…}
(v) A∩C
This set contains natural numbers that are multiples of both 2 and 5.
- Result: A∩C={10,20,30,40,50,…}
(vi) A∩B∩C
This set contains natural numbers that are multiples of 2, 3, and 5.
- Result: A∩B∩C={30,60,90,120,150,…}
(vii) (A∪B)−C
This set contains all natural numbers that are multiples of 2 or 3 but not multiples of 5.
- Result: (A∪B)−C={2,3,4,6,8,12,14,16,18,21,24,27,…}
Summary of Results
1. A∪B={2,3,4,6,8,9,10,12,14,15,16,18,20,21,24,27,30,…}
2. B∪C={3,5,6,9,10,12,15,18,20,21,24,25,30,35,40,…}
3. A−B={2,4,8,10,14,16,20,22,26,28,…}
4. B−C={3,6,9,12,18,21,24,27,…}
5. A∩C={10,20,30,40,50,…}
6. A∩B∩C={30,60,90,120,150,…}
7. (A∪B)−C={2,3,4,6,8,12,14,16,18,21,24,27,…}
- Set A: The set of natural numbers which are multiples of 2.
A={2,4,6,8,10,12,14,16,18,20,…}
B={3,6,9,12,15,18,21,24,27,30,…}
C={5,10,15,20,25,30,35,40,45,50,…}
(i) A∪B
This set contains all natural numbers that are multiples of 2 or 3.
- Result: A∪B={2,3,4,6,8,9,10,12,14,15,16,18,20,21,24,27,30,…}
This set contains all natural numbers that are multiples of 3 or 5.
- Result: B∪C={3,5,6,9,10,12,15,18,20,21,24,25,30,35,40,…}
This set contains natural numbers that are multiples of 2 but not multiples of 3.
- Result: A−B={2,4,8,10,14,16,20,22,26,28,…}
This set contains natural numbers that are multiples of 3 but not multiples of 5.
- Result: B−C={3,6,9,12,18,21,24,27,…}
This set contains natural numbers that are multiples of both 2 and 5.
- Result: A∩C={10,20,30,40,50,…}
This set contains natural numbers that are multiples of 2, 3, and 5.
- Result: A∩B∩C={30,60,90,120,150,…}
This set contains all natural numbers that are multiples of 2 or 3 but not multiples of 5.
- Result: (A∪B)−C={2,3,4,6,8,12,14,16,18,21,24,27,…}
1. A∪B={2,3,4,6,8,9,10,12,14,15,16,18,20,21,24,27,30,…}
2. B∪C={3,5,6,9,10,12,15,18,20,21,24,25,30,35,40,…}
3. A−B={2,4,8,10,14,16,20,22,26,28,…}
4. B−C={3,6,9,12,18,21,24,27,…}
5. A∩C={10,20,30,40,50,…}
6. A∩B∩C={30,60,90,120,150,…}
7. (A∪B)−C={2,3,4,6,8,12,14,16,18,21,24,27,…}
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - SET THEORY AND REAL NUMBER SYSTEM EXERCISE - Solved Exp11videos
SET THEORY AND REAL NUMBER SYSTEM
- BOOK - CENGAGE ENGLISHCHAPTER - SET THEORY AND REAL NUMBER SYSTEM EXERCISE - Concept Application Exercise 1.112videos
SET THEORY AND REAL NUMBER SYSTEM
- BOOK - CENGAGE ENGLISHCHAPTER - SCALER TRIPLE PRODUCTS EXERCISE - DPP 2.311videos
SCALER TRIPLE PRODUCTS
- BOOK - CENGAGE ENGLISHCHAPTER - SOLUTIONS AND PROPERTIES OF TRIANGLE EXERCISE - Comprehension Type6videos
SOLUTIONS AND PROPERTIES OF TRIANGLE
Similar Questions
Write the following sets in roster form :
the set of whole numbers which are multiples of 5View SolutionFrom the given diagram find:
(i) A∪B
(ii) A'∩B
(iii) A-B
(iv) B-A
(v) (A∪B)'
 View Solution
View SolutionIf sets A=[−4,1]andB=[0,3), then find the following sets:
(a) A∩B (b) A∪B (c ) A−B
(d) B−A (e ) (A∪B)' (f) (A∩B)'View SolutionUse the given diagram to find:
(i) A∪(B∩C)
(ii) B−(A−C)
(iii) A-B
(iv) A∩B'
is A∩B'=A−B?
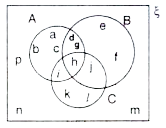 View Solution
View SolutionFrom the adjoining Venn diagram , find the following sets :
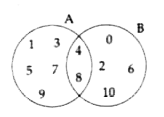
(i) A (ii) B (III) A∪B (iv) A∩BView SolutionIf A={1,2,3,4},B={3,4,5},C={1,5,6}, then findof the following :
(i) A∪B
(ii) A∪(B∩C)
(ii) A−B
(iv) A−(B∪C).View SolutionConsider the following sets:
A = set all rectangles in the same plane
B = set all squares in the same plane
C = set all parellegrams in the same plane
Find the following sets : (i)A−B(ii)C−A(iii)A∩C(iv)B∩C(v)B∪C(Vi)A∩B∪CView SolutionIf A{1,2,3},B={3,4}andC={4,5,6}, then find the each of the following :
(i) A∩(B∪C)
(ii) (A−B)∪C
(iii) (A∪B)−C
(iv) (A∩B)∪C.View SolutionProve A∪(B∩C)=(A∪B)∩(A∪C)
View SolutionThe set of the counting numbers which are multiple of 6 and less than 50 is written in set builder form as
View Solution

