Write a possible rational function f that has a vertical asymptote at x = 2, a horizontal asymptote y = 3 and a zero at x = -5. Also draw the graph of the function.
Video Solution
Text Solution
Since h has a hole at x = 5 , both the numerator and the denominator have a zero at x = 5. Also the vertical asymptote at at x = -1 means the denominator has a zero at x = -1. An x-intercept at x = 2 means the numerator has a zero at x = 2 . Finally, the horizontal asymptote y = 2 means that the numerator and the denominator have equal degree and the ratio of their leading coefficients is equal to 2. Hence
h(x)=2(x−5)(x−2)(x−5)(x+1)
The graph of h is shown below, check the characteristics.
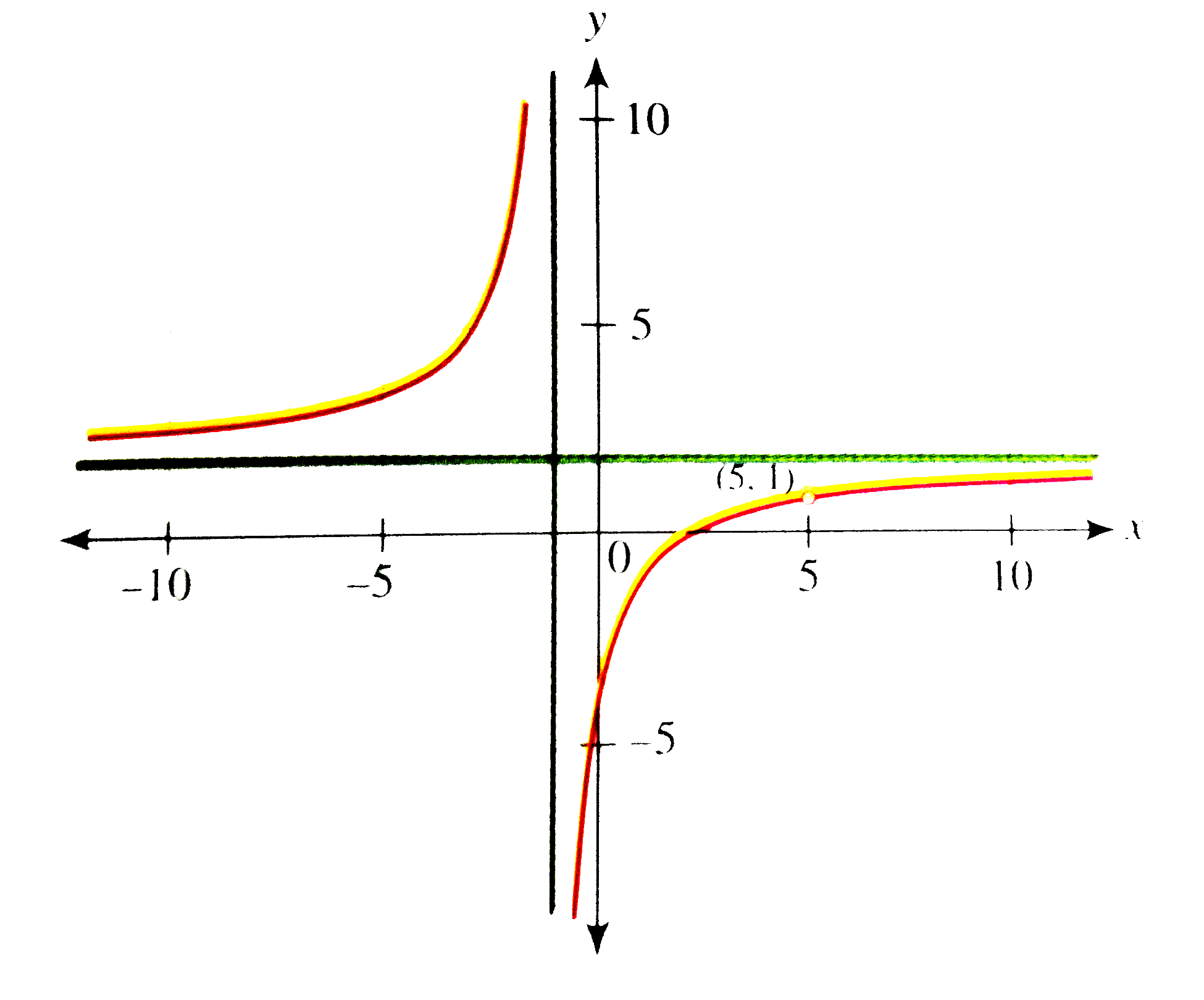
|
Updated on:21/07/2023
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONSEXERCISE - Exercises17videos
GRAPHS OF POLYNOMIAL AND RATIONAL FUNCTIONS
- BOOK - CENGAGE ENGLISHCHAPTER - GRAPHICAL TRANSFORMATIONSEXERCISE - ILLUSTRATION78videos
GRAPHICAL TRANSFORMATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - GRAPHS OF ELEMENTARY FUNCTIONS EXERCISE - EXERCISES34videos
GRAPHS OF ELEMENTARY FUNCTIONS
Similar Questions
Write a rational function g with vertical asymptotes at x = 3 and x = -3, a horizontal asymptote at y = -4 and with no x-intercept.
View SolutionWrite a rational function f with a slant asymptote y=x+4, a vertical asymptote at x = 5 and one of the zeros at x = 2.
View SolutionDraw the graph of the function f(x)=xx
View SolutionDraw the graph of the function f(x)=|x−1|
View SolutionDraw the graph of the function f(x)=−x|x|.
View SolutionDraw the graph of the following function.
2f(x)View SolutionDraw the graph of the following function.
−f(x)View SolutionDraw the graph of the following function.
f(−x)View SolutionDraw the graph of the function: f(x)=log|x|
View SolutionDraw the graph of the function: |f(x)|=tanx
View Solution

