The number of triangles that the four lines y=x+3,y=2x+3,y=3x+2, and y+x=3 form is (a) 4 (b) 2 (c) 3 (d) 1
4
2
3
1
The correct Answer is:C
The lines y=x+3, y=2x+3 and y+x=3 are concurrent at (0,3).
Rough sketch of the lines is as shown in the figure.
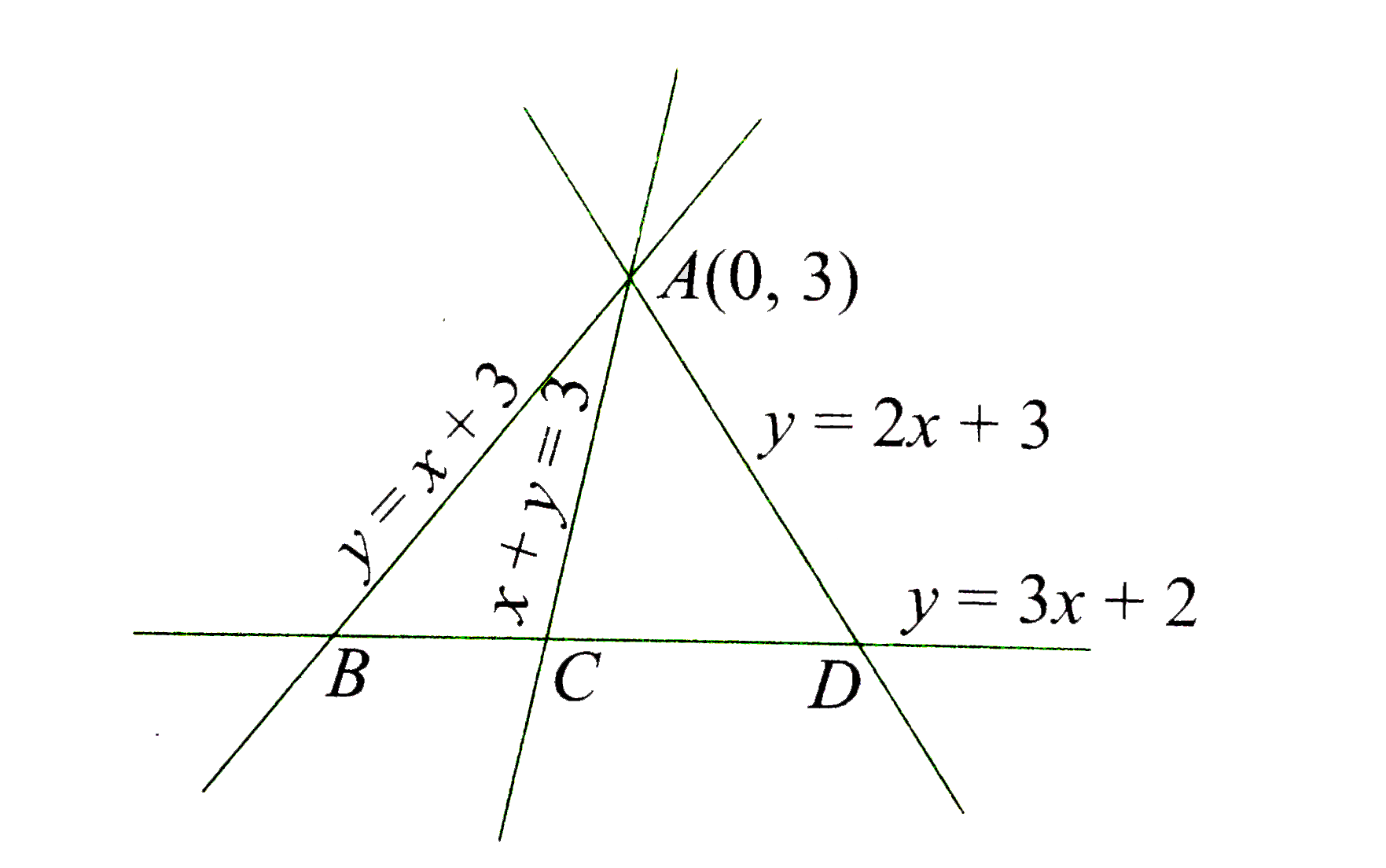
From the figure, three triangles ABD, ABC nad ACD can be formed.
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (MULTIPLE CORRECT ANSWERS TYPE)30videos
STRAIGHT LINES
- BOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - EXERCISE (LINKED COMPREHENSION TYPE)27videos
STRAIGHT LINES
- BOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - CONCEPT APPLICATION EXERCISE 2.65videos
STRAIGHT LINES
- BOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINEEXERCISE - Multiple Correct Answers Type8videos
STRAIGHT LINE
- BOOK - CENGAGE ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - JEE ADVANCED (Numerical Value Type )1videos
THEORY OF EQUATIONS
Similar Questions
Find the area of the triangle formed by the lines y=x,y=2x,y=3x+4
View SolutionFind the area of the triangle formed by the lines y=x,y=2x,y=3x+4
View SolutionThe number of circles that touch all the 3 lines 2x+y=3, 4x−y=3, x+y=2 is
View SolutionThe circumcenter of the triangle formed by the line y=x,y=2x, and y=3x+4 is
View SolutionThe triangle formed by the lines x+y=0,3x+y=4,x+3y=4 is
View SolutionFind the area of triangle formed by the lines : y=0, x=2 and x+2y=3.
View SolutionThe orthocenter of the triangle formed by lines x+y=1,2x+3y=6and4x−y+4=0 lines in quadrant number
View SolutionThe number of values of a for which the lines 2x+y−1=0 , ax+3y−3=0, and 3x+2y−2=0 are concurrent is (a).0 (b) 1 (c) 2 (d) infinite
View SolutionThe sides of an equilateral triangles are given by x+ 3y , 3x+ 2y - 2 and 4x+12y+1
View SolutionThe normal to the curve x2=4y passing (1,2) is
(A) x+y=3
(B) xy=3
(C) x+y=1
(D) xy=1View Solution

