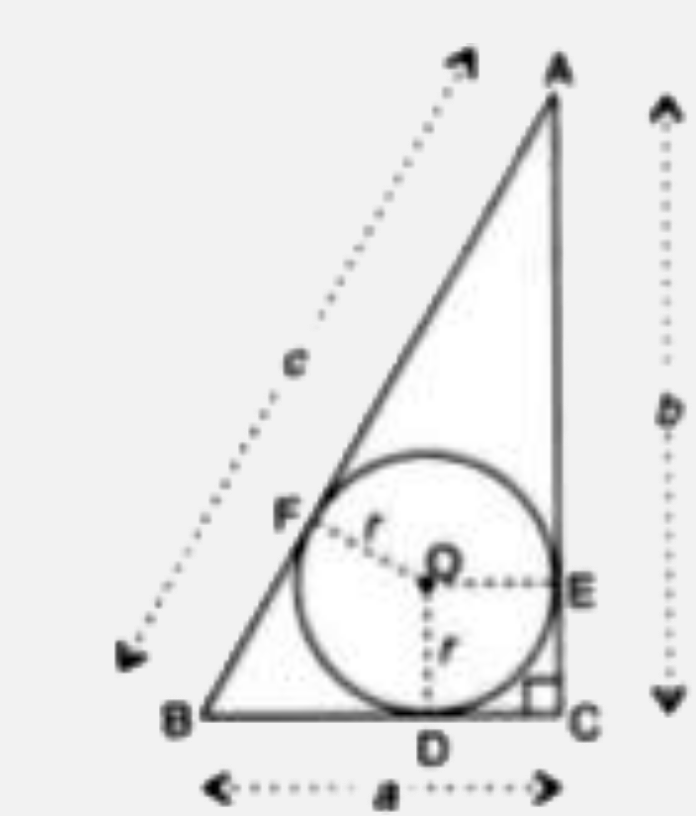
In ABC, as semicircle is inscribed, which lies on the side ⋅ If x is the lengthof the angle bisector through angle C, then prove that the radius of the semicircle is xsin(C2).

From the figure
(12)ra+(12)rb=(12)absinC
or r(a+b)=2Δ
or r=2Δa+b=absinCa+b
Also x=2aba+bcos.C2 [length of angle bisector]
From Eq. (i), r=2×(1/2)absinCa+b
=2absin.C2cos.C2a+b
=2abcos.C2a+bsin.C2
=xsinC2
Topper's Solved these Questions
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Illustration
86 Videos
- PROPERTIES AND SOLUTIONS OF TRIANGLEBOOK - CENGAGE ENGLISHCHAPTER - PROPERTIES AND SOLUTIONS OF TRIANGLEEXERCISE - Concept application exercise 5.1
12 Videos
- PROGRESSION AND SERIESBOOK - CENGAGE ENGLISHCHAPTER - PROGRESSION AND SERIESEXERCISE - ARCHIVES (MATRIX MATCH TYPE )
1 Videos
- RELATIONS AND FUNCTIONSBOOK - CENGAGE ENGLISHCHAPTER - RELATIONS AND FUNCTIONSEXERCISE - Archives(Matrix Match Type)
1 Videos
Similar Questions
In a ΔABC, a semicircle is inscribed, whose diameter lies on the side c. Then the radius of the semicircle is (Where Δ is the area of the triangle ABC)
View SolutionLet a≤b≤c be the lengths of the sides of a triangle. If a2+b2<c2,thenprovetˆ∠Cisobtuse.
View SolutionIn an acute angled triangle ABC, a semicircle with radius ra is constructed with its base on BC and tangent to the other two sides. rbandrc are defined similarly. If r is the radius of the incircle of triangle ABC then prove that 2r=1ra+1rb+1rc.
View SolutionTwo chords AB and AC of a circle are equal. Prove that the centre of the circle lies on the angle bisector of ∠BAC.
View SolutionTwo chords AB and AC of a circle are equal. Prove that the centre of the circle lies on the angle bisector of ∠BAC.
View SolutionIf a,b and c are sides of a ritht triangle where c is the hypotenuse, prove that the radius of the circle which touches the sides of the triangle is r=a+b−c2.
View SolutionIf A, B, C are the interior angles of a triangle ABC , prove that tan(B+C)2=cotA2
View SolutionIf A,B,andC are three points on the hyperbola xy=c2 such that AB subtends a right angle at C, then prove that AB is parallel to the normal to the hyperbola at point C.
View SolutionIf A,B,andC are three points on the hyperbola xy=c2 such that AB subtends a right angle at C, then prove that AB is parallel to the normal to the hyperbola at point C.
View SolutionIf a+b+c≠0 and |abcbcacab|=0 , then prove that a=b=⋅
View Solution
