Question

Let ${\displaystyle C_1\text{and}{C}_2}$ be the graphs of the functions ${\displaystyle y=x^2\text{and}y=2x,}$ respectively, where ${\displaystyle 0\le x\le 1.\text{Let}{C}_3}$ be the graph of a function y=f(x), where ${\displaystyle 0\le x\le 1,f\left(0\right)=0.}$ For a point P on ${\displaystyle C_1,}$ let the lines through P, parallel to the axes, meet ${\displaystyle C_2\text{and}{C}_3}$ at Q and R, respectively (see figure). If for every position of ${\displaystyle P\left(on{C}_1\right),}$ the areas of the shaded regions OPQ and ORP are equal, determine the function f(x).

Explanation

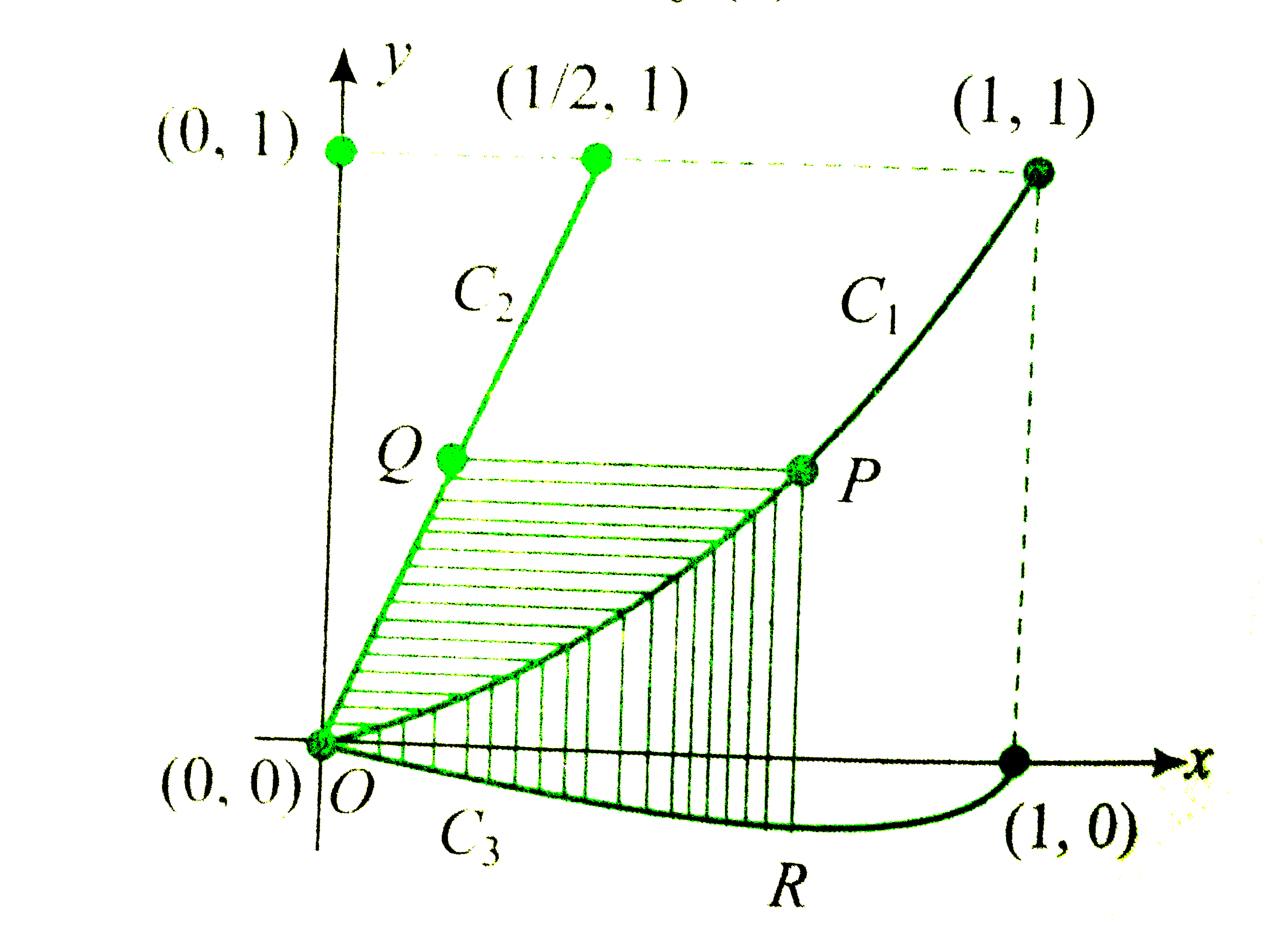
Let P be on `C_(1),y=x^(2) be (t,t^(2))`
`therefore" y co-ordinate of Q is also "t^(2)`
`"Now, Q on y =2x where "y=t^(2)`
`therefore" "x=t^(2)//2`
`therefore" "Q((t^(2))/(2),t^(2))`
For point R, x=t and it is on y=f(x)
`therefore" "R(t,f(t))`
Given that,
Area OPQ = Area OPR
`rArr" "int_(0)^(t^(2))(sqrt(y)-(y)/(2))dy=int_(0)^(t)(x^(2)-f(x))dx`
Differentiating both sides w.r.t. t, we get
`(sqrt(t^(2))-(t^(2))/(2))(2t)=t^(2)-f(t)`
`rArr" "f(t)=t^(3)-t^(2)`
`rArr" "f(x)=x^(3)-x^(2)`