a and b form the consecutive sides of a regular hexagon ABCDEF.

Video Solution

Text Solution

Verified by Experts

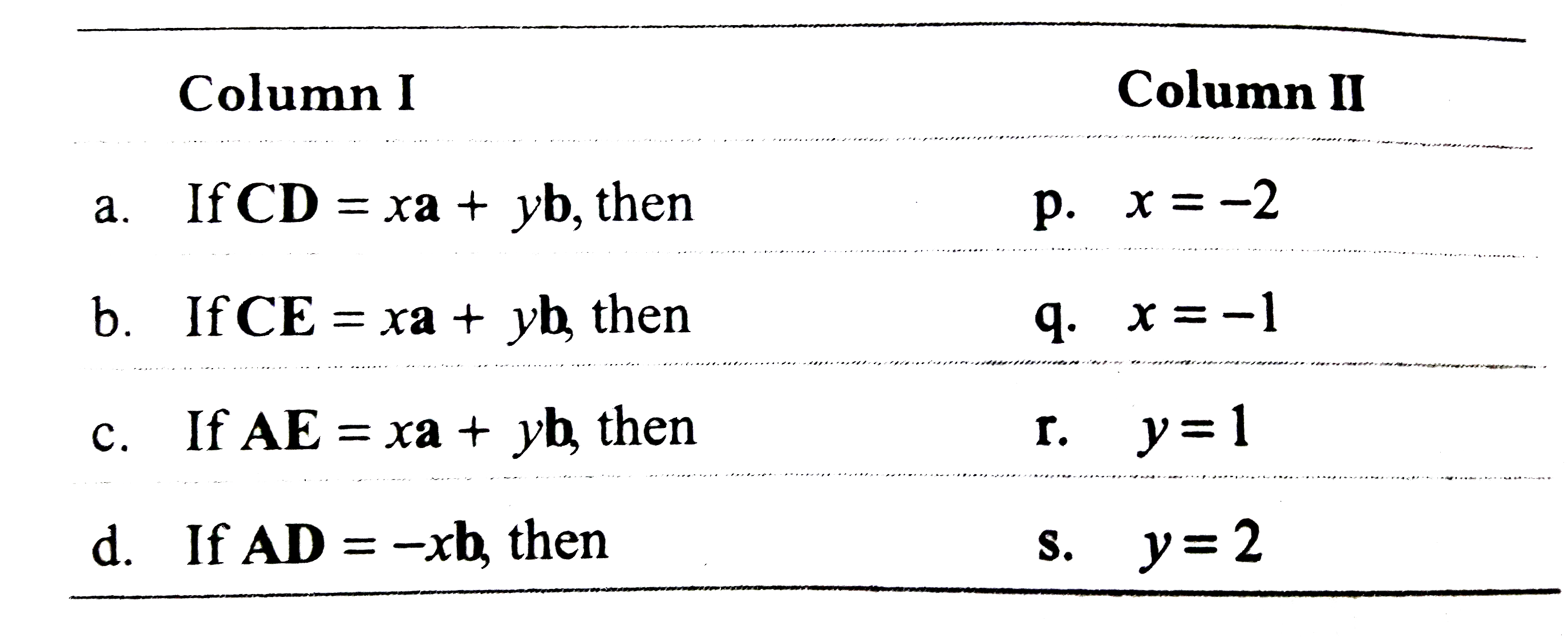
The correct Answer is: $a \to q, r; b \to p, r; c \to q, s; d \to p$

$- - \to A B = \to a, - - \to B C = \to b$ ltvrgt $∴ - - \to A C = - - \to A B + - - \to B C = \to a + \to a$ (i)
$- - \to A D = 2 - - \to B C = 2 \to b$ (ii)
(because AD is parallel to BC and twice its length).
$- - \to C D = - - \to A D - - - \to A C = 2 \to b - (\to a + \to b)$
$= \to b - \to a$
$- - \to F A = - - - \to C D = \to a - \to b$ (iii)
$- - \to D E = - - - \to A B = - \to a$ (iv)
$- - \to E F = - - - \to B C = - \to b$ (v)
$- - \to A E = - - \to A D + - - \to D E = 2 \to b - \to a$ (vi)
$- - \to C E = - - \to C D + - - \to D E = \to b - \to a - \to a = \to b - 2 \to a$ (vii)

Updated on:21/07/2023

|

Class 12 MATHS VECTOR ALGEBRA

Topper's Solved these Questions

Similar Questions

CENGAGE ENGLISH-INTRODUCTION TO VECTORS -MATRIX-MATCH TYPE

Home
Ask Doubt
Profile