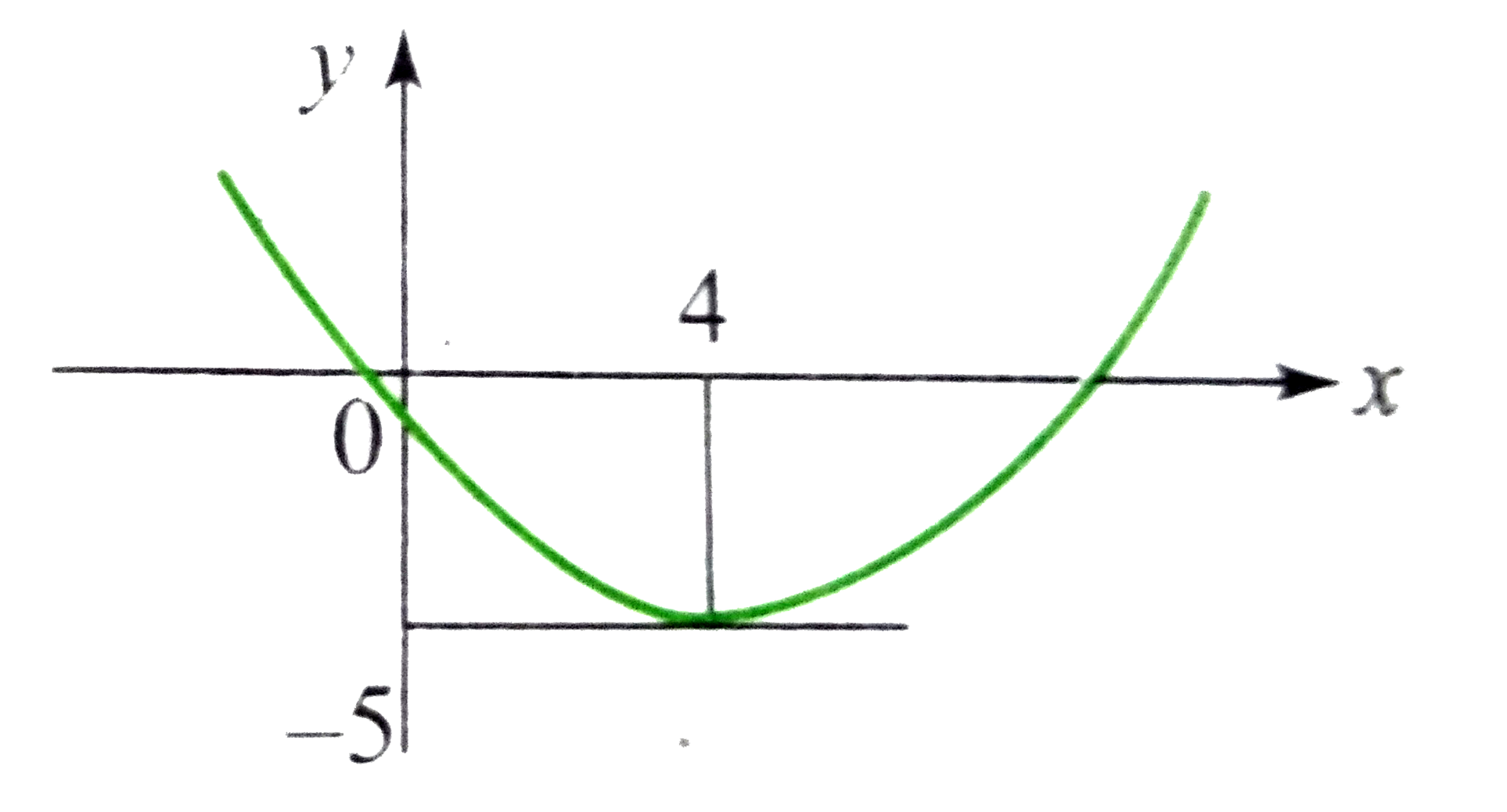
The graph of the quadratic trinomial u=ax2+bx+c has its vertex at (4, -5) and two x-intercepts, one positive and one negative. Which of the following holds good? a. a>0 b. b<0 c. c<0 d. 8a=b
a>0
b<0
c<0
8a=b
The correct Answer is:1,2,3
From figure,
a>0
−b2a=4or−b2a>0
∴b<0
f(0)=c<0
Also , −b2a=4or8a+b=0
Topper's Solved these Questions
- BOOK - CENGAGE ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - Linked Comprechension Type37videos
THEORY OF EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - MATRIX MATCH TYPE6videos
THEORY OF EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - THEORY OF EQUATIONSEXERCISE - Single Correct Answer Type : Exercise89videos
THEORY OF EQUATIONS
- BOOK - CENGAGE ENGLISHCHAPTER - STRAIGHT LINESEXERCISE - ARCHIVES (NUMERICAL VALUE TYPE)1videos
STRAIGHT LINES
- BOOK - CENGAGE ENGLISHCHAPTER - THREE DIMENSIONAL GEOMETRYEXERCISE - All Questions294videos
THREE DIMENSIONAL GEOMETRY
Similar Questions
If a,b,c,d,e are positive numbers, then which of the following holds good
View SolutionThe graph of a quadratic polynomial y=ax2+bx+c,a,b,εR is shown. Find its vertex,roots and D.
View SolutionIf graph of quadratic polynomial ax2+bx+c cuts positive direction of y-axis, then what is the sign of c ?
View SolutionIf the graph of quadratic polynomial ax2+bx+c cuts negative direction of y-axis, then what is the sign of c ?
View SolutionIf the quadratic equation ax2+bx+c=0(a>0) has sec2θandcosec2θ as its roots, then which of the following must hold good? b+c=0 b. b2−4ac≥0 c. ≥4a d. 4a+b≥0
View SolutionThe roots of the quadratic equation (a+b−2c)x2+(2a−b−c)x+(a−2b+c)=0 are
View SolutionIf the quadratic equation ax2+bx+c=0(a>0) has sec2θandcosec2θ as its roots, then which of the following must hold good? (a.) b+c=0 (b.) b2−4ac≥0 (c.) c ≥4a (d.) 4a+b≥0
View SolutionIf a,b∈R,a≠0 and the quadratic equation ax2−bx+1=0 has imaginary roots, then (a+b+1) is a. positive b. negative c. zero d. Dependent on the sign of b
View SolutionA quadratic trinomial P(x)=ax2+bx+c is such that the equation P(x)=x has o real roots. Prove that in this case equation P(P(x))=x has no real roots either.
View SolutionIf one of the zeroes of a quadratic polynomial of the form x2+ax+b is the negative of the other, then it
View Solution

