Find the mean deviation from the mean for the following data: Size:, 20, 21, 22, 23, 24 frequency, 6, 4, 5, 1, 4
To find the mean deviation from the mean for the given data, we will follow these steps:
Step 1: Organize the Data
We have the following sizes (x_i) and their corresponding frequencies (f_i):
| Size (x_i) | Frequency (f_i) |
|------------|------------------|
| 20 | 6 |
| 21 | 4 |
| 22 | 5 |
| 23 | 1 |
| 24 | 4 |
Step 2: Calculate the Total Frequency (Σf_i)
We need to sum the frequencies:
Σfi=6+4+5+1+4=20
Step 3: Calculate Σ(f_i * x_i)
Now we calculate the product of each size and its frequency, then sum those products:
fi⋅xi:6⋅20=1204⋅21=845⋅22=1101⋅23=234⋅24=96
Now summing these products:
Σ(fi⋅xi)=120+84+110+23+96=433
Step 4: Calculate the Mean (x̄)
Using the formula for the mean:
¯x=Σ(fi⋅xi)Σfi=43320=21.65
Step 5: Calculate the Deviations (d_i)
Now we calculate the absolute deviations from the mean for each size:
di=|xi−¯x|
Calculating each deviation:
d1=|20−21.65|=1.65d2=|21−21.65|=0.65d3=|22−21.65|=0.35d4=|23−21.65|=1.35d5=|24−21.65|=2.35
Step 6: Calculate f_i * d_i
Now we calculate the product of each frequency and its corresponding deviation:
fi⋅di:6⋅1.65=9.94⋅0.65=2.65⋅0.35=1.751⋅1.35=1.354⋅2.35=9.4
Now summing these products:
Σ(fi⋅di)=9.9+2.6+1.75+1.35+9.4=25
Step 7: Calculate the Mean Deviation
Finally, we calculate the mean deviation using the formula:
Mean Deviation=Σ(fi⋅di)Σfi=2520=1.25
Final Answer
The mean deviation from the mean for the given data is 1.25.
---
We have the following sizes (x_i) and their corresponding frequencies (f_i):
|------------|------------------|
| 20 | 6 |
| 21 | 4 |
| 22 | 5 |
| 23 | 1 |
| 24 | 4 |
We need to sum the frequencies:
Now we calculate the product of each size and its frequency, then sum those products:
Using the formula for the mean:
Now we calculate the absolute deviations from the mean for each size:
Now we calculate the product of each frequency and its corresponding deviation:
Finally, we calculate the mean deviation using the formula:
The mean deviation from the mean for the given data is 1.25.
Topper's Solved these Questions
Similar Questions
Find the mean deviation from the mean for the followinng data:

View SolutionFind the mean deviation from the median for the following data:
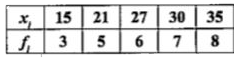
View SolutionFind the mean deviation for the mean for the following data:

View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about the mean for the following data.
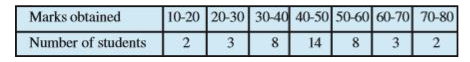
View SolutionFind the mean deviation about the mean for the following data :

View SolutionFind the mean deviation about mean for the following data:
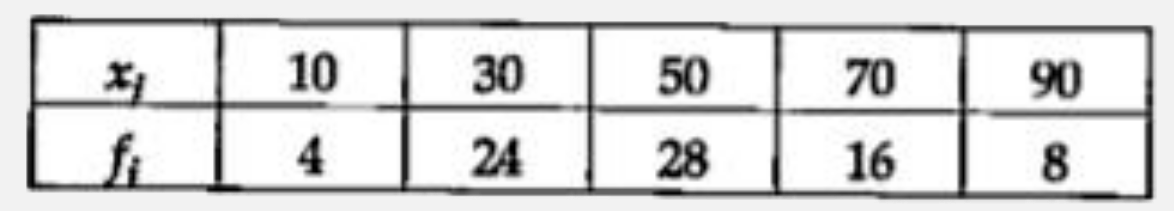
View SolutionFind the mean deviation about median for the following data:

View SolutionFind the mean deviation using median for the following datas:

View Solution
