There are 60 students in a class. The following is the frequency distribution of marks obtained by the students in a test: Marks: 0 1 2 3 4 5 Frequency x−2 , x , x2 , (x+1)2 , 2x , x+1 Determine the mean and standard deviation.
To solve the problem, we need to determine the value of x first, and then use it to calculate the mean and standard deviation of the given frequency distribution.
Step 1: Find the value of x
We know that the total frequency must equal the total number of students, which is 60. The frequencies given are:
- x−2
- x
- x2
- (x+1)2
- 2x
- x+1
We can set up the equation:
(x−2)+x+x2+(x+1)2+2x+(x+1)=60
Now, we will expand and simplify this equation:
1. Expand (x+1)2:
(x+1)2=x2+2x+1
2. Substitute back into the equation:
(x−2)+x+x2+(x2+2x+1)+2x+(x+1)=60
3. Combine like terms:
x−2+x+x2+x2+2x+1+2x+x+1=60
2x2+7x+0=60
4. Rearranging gives:
2x2+7x−60=0
Step 2: Solve the quadratic equation
Using the quadratic formula x=−b±√b2−4ac2a:
Here, a=2, b=7, and c=−60.
1. Calculate the discriminant:
b2−4ac=72−4⋅2⋅(−60)=49+480=529
2. Now apply the quadratic formula:
x=−7±√5292⋅2=−7±234
3. This gives two potential solutions:
x=164=4andx=−304=−7.5(not valid)
Thus, x=4.
Step 3: Calculate frequencies
Now substitute x=4 into the frequency distribution:
- x−2=4−2=2
- x=4
- x2=42=16
- (x+1)2=(4+1)2=25
- 2x=2⋅4=8
- x+1=4+1=5
The frequency distribution becomes:
| Marks | Frequency |
|-------|-----------|
| 0 | 2 |
| 1 | 4 |
| 2 | 16 |
| 3 | 25 |
| 4 | 8 |
| 5 | 5 |
Step 4: Calculate the Mean
The mean ¯x is calculated using the formula:
¯x=∑(fi⋅xi)∑fi
Where fi is the frequency and xi is the marks.
1. Calculate fi⋅xi:
| Marks | Frequency | fi⋅xi |
|-------|-----------|---------------------|
| 0 | 2 | 0 |
| 1 | 4 | 4 |
| 2 | 16 | 32 |
| 3 | 25 | 75 |
| 4 | 8 | 32 |
| 5 | 5 | 25 |
2. Sum of fi:
∑fi=2+4+16+25+8+5=60
3. Sum of fi⋅xi:
∑(fi⋅xi)=0+4+32+75+32+25=168
4. Now calculate the mean:
¯x=16860=2.8
Step 5: Calculate the Standard Deviation
The standard deviation σ is calculated using the formula:
σ=√∑(fi⋅(xi−¯x)2)∑fi
1. Calculate (xi−¯x)2:
| Marks | Frequency | xi−¯x | (xi−¯x)2 | fi⋅(xi−¯x)2 |
|-------|-----------|----------------------|--------------------------|-----------------------------------|
| 0 | 2 | 0−2.8=−2.8 | 7.84 | 15.68 |
| 1 | 4 | 1−2.8=−1.8 | 3.24 | 12.96 |
| 2 | 16 | 2−2.8=−0.8 | 0.64 | 10.24 |
| 3 | 25 | 3−2.8=0.2 | 0.04 | 1.00 |
| 4 | 8 | 4−2.8=1.2 | 1.44 | 11.52 |
| 5 | 5 | 5−2.8=2.2 | 4.84 | 24.20 |
2. Sum of fi⋅(xi−¯x)2:
∑(fi⋅(xi−¯x)2)=15.68+12.96+10.24+1.00+11.52+24.20=75.60
3. Now calculate the standard deviation:
σ=√75.6060=√1.26≈1.12
Final Results
- Mean: 2.8
- Standard Deviation: 1.12
- x
- x2
- (x+1)2
- 2x
- x+1
(x+1)2=x2+2x+1
(x−2)+x+x2+(x2+2x+1)+2x+(x+1)=60
x−2+x+x2+x2+2x+1+2x+x+1=60
2x2+7x+0=60
2x2+7x−60=0
b2−4ac=72−4⋅2⋅(−60)=49+480=529
x=−7±√5292⋅2=−7±234
x=164=4andx=−304=−7.5(not valid)
- x=4
- x2=42=16
- (x+1)2=(4+1)2=25
- 2x=2⋅4=8
- x+1=4+1=5
|-------|-----------|
| 0 | 2 |
| 1 | 4 |
| 2 | 16 |
| 3 | 25 |
| 4 | 8 |
| 5 | 5 |
|-------|-----------|---------------------|
| 0 | 2 | 0 |
| 1 | 4 | 4 |
| 2 | 16 | 32 |
| 3 | 25 | 75 |
| 4 | 8 | 32 |
| 5 | 5 | 25 |
∑fi=2+4+16+25+8+5=60
∑(fi⋅xi)=0+4+32+75+32+25=168
¯x=16860=2.8
|-------|-----------|----------------------|--------------------------|-----------------------------------|
| 0 | 2 | 0−2.8=−2.8 | 7.84 | 15.68 |
| 1 | 4 | 1−2.8=−1.8 | 3.24 | 12.96 |
| 2 | 16 | 2−2.8=−0.8 | 0.64 | 10.24 |
| 3 | 25 | 3−2.8=0.2 | 0.04 | 1.00 |
| 4 | 8 | 4−2.8=1.2 | 1.44 | 11.52 |
| 5 | 5 | 5−2.8=2.2 | 4.84 | 24.20 |
∑(fi⋅(xi−¯x)2)=15.68+12.96+10.24+1.00+11.52+24.20=75.60
σ=√75.6060=√1.26≈1.12
- Standard Deviation: 1.12
Topper's Solved these Questions
Similar Questions
There are 60 students in a class. The following is the frequency distribution of marks obtained by the students in a test: Marks:, 0, 1, 2, 3, 4, 5 Frequency , x−2 , x , x2 , (x+1)2 , 2x , x+1. Determine mean and standard deviation of the marks.
View SolutionThere are 60 students in a class. The followng is the frequency distribution of marks obtained by the students in a test.
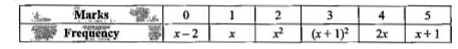
where x is positive integer. Determine the mean of the marks.
View Solution
Knowledge Check
The frequency distribution for x is an given below. What is the range of f? <br>{:(x,f),(0,1),(1,5),(2,4),(3,4):}
A0B1C3D4
If for somex∈R, the frequency distribution of the marks obtained by 20 students in a test is:
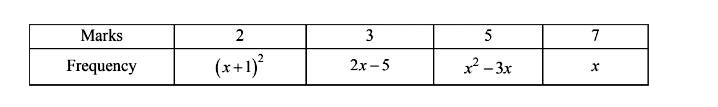
Then the mean of the marks is:
View SolutionFind the mode of the following frequency distribution of marks obtained by 50 students. Marks obtained 0−10 10−20 20−30 30−40 40−50 No. of students 5 12 20 10 3
View SolutionFind the median for the following frequency distribution :
Marks obtained(xi)293234274237Number of students(fi)25101284
View SolutionThe mean deviation about the median of the following distribution is
Marks obtained1011121415Number of students23834
View SolutionFollowing is the distribution fo marks obtained by 50 students in a test.
Marks more than01020304050No. of Students50464020103
If 60% of the students pass the test, find the minimum marks obtained by a pass students.
View SolutionThe frequency distribution of marks obtained by 28 students in a test carrying 40 marks is given below:
Marks:0−1010−2020−3030−40Number of students:6xy6
If the mean of the above data is 20, then the difference between x and y is
View SolutionFind The standard deviation of the following frequency distribution is
X234567f491614116
View Solution
