A toy is in the shape of a right circular cylinder with a hemisphere on one end and a cone on the other. The height and radius of the cylindrical part are 13cm and 5cm respectively. The radii of the hemispherical and conical parts are the same as that of the cylindrical part. Calculate the surface area of the toy if height of the conical part is 12cm.
To find the surface area of the toy, which consists of a right circular cylinder, a hemisphere, and a cone, we will follow these steps:
Step 1: Identify the dimensions
- Height of the cylindrical part (H_cylinder) = 13 cm
- Radius (R) = 5 cm (same for all parts)
- Height of the conical part (H_cone) = 12 cm
Step 2: Calculate the surface area of the cylindrical part
The formula for the curved surface area of a cylinder is given by:
Curved Surface Area of Cylinder=2πRH
Substituting the values:
Curved Surface Area of Cylinder=2×227×5×13
Calculating this:
=2×227×5×13=28607cm2
Step 3: Calculate the surface area of the conical part
First, we need to find the slant height (L) of the cone using the Pythagorean theorem:
L=√R2+H2cone
Substituting the values:
L=√52+122=√25+144=√169=13cm
Now, the formula for the curved surface area of a cone is:
Curved Surface Area of Cone=πRL
Substituting the values:
Curved Surface Area of Cone=227×5×13
Calculating this:
=14307cm2
Step 4: Calculate the surface area of the hemispherical part
The formula for the curved surface area of a hemisphere is:
Curved Surface Area of Hemisphere=2πR2
Substituting the values:
Curved Surface Area of Hemisphere=2×227×52
Calculating this:
=2×227×25=11007cm2
Step 5: Calculate the total surface area of the toy
The total surface area is the sum of the curved surface areas of the cylinder, cone, and hemisphere:
Total Surface Area=Curved Surface Area of Cylinder+Curved Surface Area of Cone+Curved Surface Area of Hemisphere
Substituting the values:
Total Surface Area=28607+14307+11007
Calculating this:
=53907=770cm2
Final Answer
The total surface area of the toy is 770cm2.
---
- Height of the cylindrical part (H_cylinder) = 13 cm
- Radius (R) = 5 cm (same for all parts)
- Height of the conical part (H_cone) = 12 cm
The formula for the curved surface area of a cylinder is given by:
Curved Surface Area of Cylinder=2πRH
Substituting the values:
Curved Surface Area of Cylinder=2×227×5×13
Calculating this:
=2×227×5×13=28607cm2
First, we need to find the slant height (L) of the cone using the Pythagorean theorem:
L=√R2+H2cone
Substituting the values:
L=√52+122=√25+144=√169=13cm
Now, the formula for the curved surface area of a cone is:
Curved Surface Area of Cone=πRL
Substituting the values:
Curved Surface Area of Cone=227×5×13
Calculating this:
=14307cm2
The formula for the curved surface area of a hemisphere is:
Curved Surface Area of Hemisphere=2πR2
Substituting the values:
Curved Surface Area of Hemisphere=2×227×52
Calculating this:
=2×227×25=11007cm2
The total surface area is the sum of the curved surface areas of the cylinder, cone, and hemisphere:
Total Surface Area=Curved Surface Area of Cylinder+Curved Surface Area of Cone+Curved Surface Area of Hemisphere
Substituting the values:
Total Surface Area=28607+14307+11007
Calculating this:
=53907=770cm2
The total surface area of the toy is 770cm2.
Topper's Solved these Questions
- BOOK - RD SHARMA ENGLISHCHAPTER - SURFACE AREA AND VOLUME OF A RIGHT CIRCULAR CYLINDER CONEEXERCISE - All Questions129videos
SURFACE AREA AND VOLUME OF A RIGHT CIRCULAR CYLINDER CONE
- BOOK - RD SHARMA ENGLISHCHAPTER - TABULAR REPRESENTATION OF STATISTICAL DATA EXERCISE - All Questions70videos
TABULAR REPRESENTATION OF STATISTICAL DATA
Similar Questions
A toy is in the shape of a right circular cylinder with a hemisphere on one end and a cone on the other. The height and radius of the cylindrical par are 13cm and 5cm respectively. The radii of the hemispherical and conical parts re the same as that of the cylindrical part. Calculate the surface area of the toy if height of the conical part is 12 cm.
View SolutionA solid toy is in the form of a right circular cylinder with a hemispherical shape at one end and a cone at the other end. Their common diameter is 4.2 cm. and the height of the cylindrical and conical portions are 12 cm and 7 cm respectively. Find the volume of the solid toy. (Use π=22/7 )
View SolutionA solid is in the form of a right circular cylinder, with a hemisphere at one end and a cone at the other end. The radius of the common base is 3.5 cm and the heights of the cylindrical and conical portions are 10 cm and 6 cm, respectively. Find the total surface area of the solid. (Use π=22/7 )
View SolutionFind the total surface area of a right circular cone with radius 6cm and height 8cm.
View SolutionThe following figure represents a solid consiting of right circular cylinder with a hemisphere at one end and a cone at the other. Their common radius is 7 cm. The height of the cylinder and cone are each of 4 cm. Find the volume of the solid.
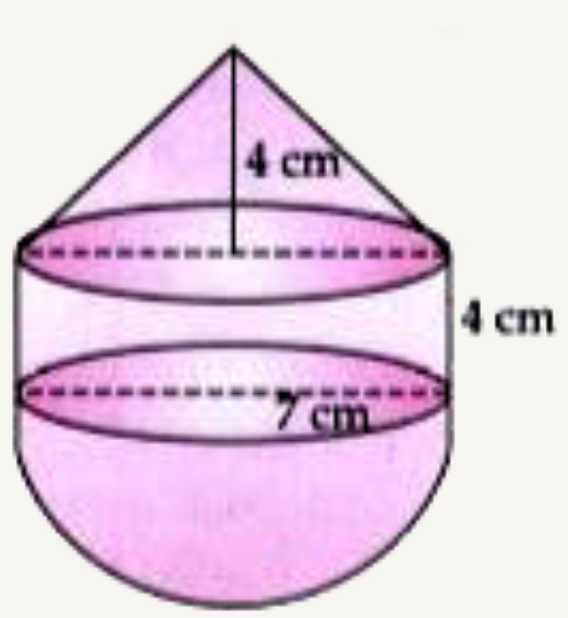 View Solution
View SolutionA tent is in the shape of a cylinder surmounted by a conical top. If the height and diameter of the cylindrical part are 2.1 m and 4 m respectively, and the slant height of the top is 2.8 m, find the area of the canvas used for making the tent. Also find the rate of the canvas with 500 per m2
View SolutionA tent is in the shape of a cylinder surmounted by a conical top. If the height and diameter of the cylindrical part are 2.1 m and 4 m respectively, and the slant height of the top is 2.8 m, find the area of the canvas used for making the tent. Also find the rate of the canvas with 500 per m2
View SolutionA circus tent is in the form of a right circular cylinder and right circular cone above it . The diameter and the height of the cylindrical part of the tent are 126 m and 5 m respectively.The totla height of the tent is 21 m. Find the total cost of the tent if the canvas used costsRs 12 per square metre.
View SolutionA rocket is in the form of a right circular cylinder closed at the lower end and surmounted by a cone with the same radius as that of the cylinder. The diamter and height of the cylinder are 6 cm and 12 cm , respectively. If the slant height of the conical portion is 5 cm, the find the total surface area and volume of the rocket. ( use π=3.14).
View SolutionThe radius of the base and the height of a right circular cone are 7 cm and 24 cm respectively. Find the volume and the total surface area of the cone.
View Solution

