Question

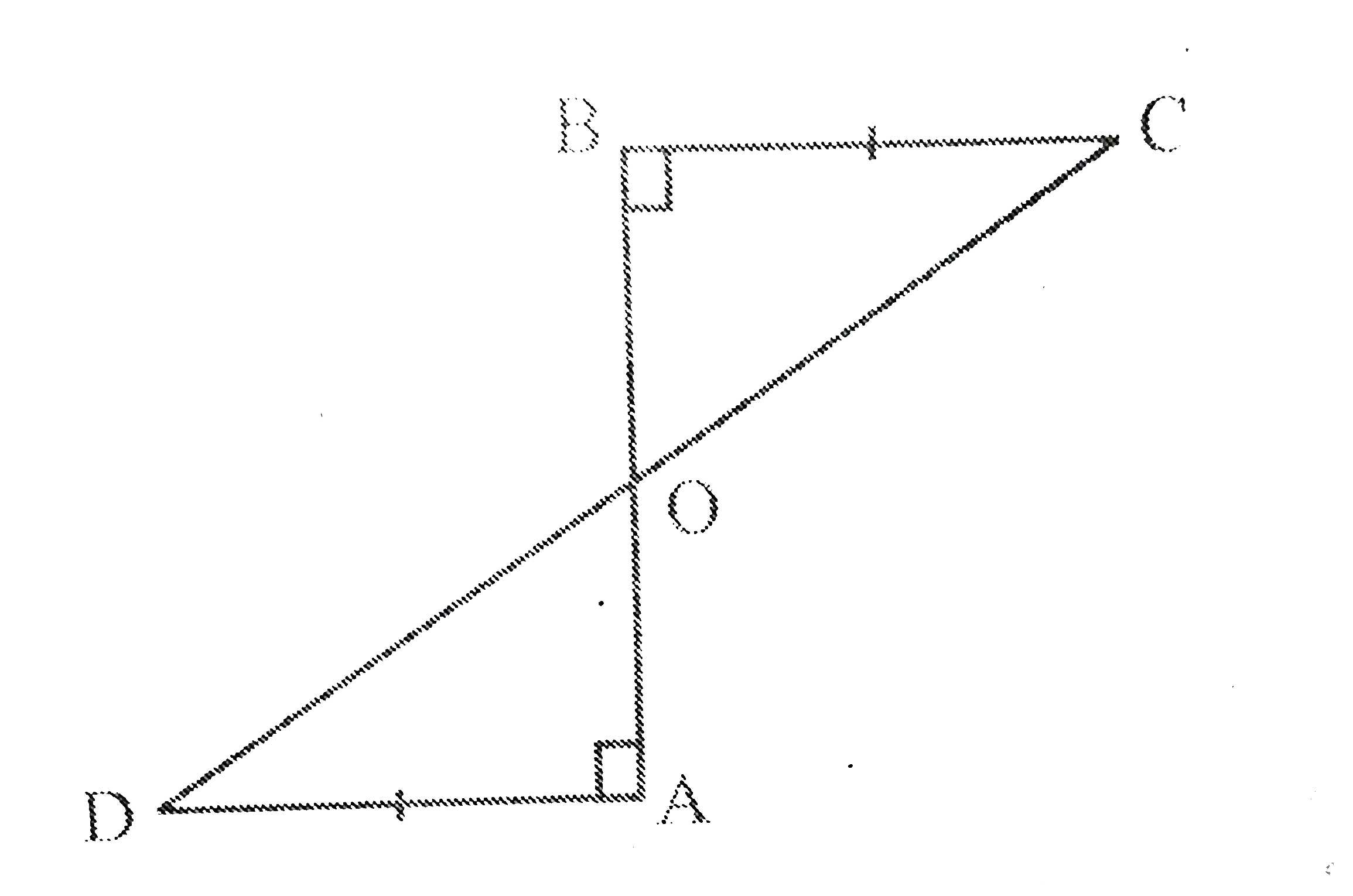
$AD$
and $BC$
are equal perpendiculars to a line segment $AB$
. Show that $CD$
bisects $AB\stackrel{.}{}$

Explanation

To prove that line segment $$CD$$ bisects line segment $$AB$$, we will follow these steps:

1. Identify the Given Information:
We are given that \( AD \) and \( BC \) are equal perpendiculars to the line segment \( AB \). This means:
- \( AD = BC \)
- \( AD \perp AB \)
- \( BC \perp AB \)

2. Draw the Diagram:
Draw a line segment \( AB \). From point \( A \), draw a perpendicular line \( AD \) and from point \( B \), draw another perpendicular line \( BC \). Label the intersection of line segments \( AD \) and \( BC \) as point \( O \).

3. Establish Angles:
Since \( AD \) and \( BC \) are perpendicular to \( AB \):
- \( \angle OAD = 90^\circ \)
- \( \angle OBC = 90^\circ \)

4. Use Vertically Opposite Angles:
Since \( AD \) and \( BC \) intersect at point \( O \), the angles \( \angle AOD \) and \( \angle BOC \) are vertically opposite angles:
- \( \angle AOD = \angle BOC \)

5. Consider Triangles:
Now, look at triangles \( AOB \) and \( BOC \):
- In triangle \( AOB \):
- \( \angle AOB = \angle AOD + \angle OAD = \angle BOC + \angle OBC \)
- Since \( AD = BC \) and both angles \( OAD \) and \( OBC \) are \( 90^\circ \), we can say:
- \( \triangle AOB \cong \triangle BOC \) by the Angle-Angle-Side (AAS) congruence theorem.

6. Conclude Corresponding Parts:
From the congruence of triangles \( AOB \) and \( BOC \):
- \( AO = BO \) (corresponding parts of congruent triangles)

7. Final Conclusion:
Since \( AO = BO \), we conclude that line segment \( CD \) bisects line segment \( AB \).

Summary:
Thus, we have shown that \( CD \) bisects \( AB \).

---