If D is any point on the base BC produced, of an isosceles triangle ABC , prove that AD>AB.
To prove that AD>AB when D is any point on the base BC produced of an isosceles triangle ABC, we can follow these steps:
1. Identify the Isosceles Triangle:
- We have triangle ABC where AB=AC (since it is given that ABC is an isosceles triangle).
2. Establish Equal Angles:
- Since AB=AC, by the properties of isosceles triangles, the angles opposite to these sides are equal. Therefore, ∠ABC=∠ACB.
3. Consider Triangle ACD:
- Now, we will consider triangle ACD. In this triangle, D is a point on the extended line of base BC.
4. Use the Exterior Angle Theorem:
- In triangle ACD, the angle ∠ACB is an exterior angle. According to the exterior angle theorem, the exterior angle ∠ACB is greater than the opposite interior angle ∠ADC. Thus, we have:
∠ACB>∠ADC
5. Relate Angles:
- Since ∠ABC=∠ACB (from step 2), we can also say:
∠ABC>∠ADC
6. Apply the Triangle Inequality:
- In triangle ACD, the side opposite to the greater angle ∠ABC is AD, and the side opposite to the smaller angle ∠ADC is AB. By the property of triangles, the side opposite to a greater angle is longer than the side opposite to a smaller angle. Therefore:
AD>AB
7. Conclusion:
- Hence, we have proved that AD>AB.
- We have triangle ABC where AB=AC (since it is given that ABC is an isosceles triangle).
- Since AB=AC, by the properties of isosceles triangles, the angles opposite to these sides are equal. Therefore, ∠ABC=∠ACB.
- Now, we will consider triangle ACD. In this triangle, D is a point on the extended line of base BC.
- In triangle ACD, the angle ∠ACB is an exterior angle. According to the exterior angle theorem, the exterior angle ∠ACB is greater than the opposite interior angle ∠ADC. Thus, we have:
∠ACB>∠ADC
- Since ∠ABC=∠ACB (from step 2), we can also say:
∠ABC>∠ADC
- In triangle ACD, the side opposite to the greater angle ∠ABC is AD, and the side opposite to the smaller angle ∠ADC is AB. By the property of triangles, the side opposite to a greater angle is longer than the side opposite to a smaller angle. Therefore:
AD>AB
- Hence, we have proved that AD>AB.
Topper's Solved these Questions
Similar Questions
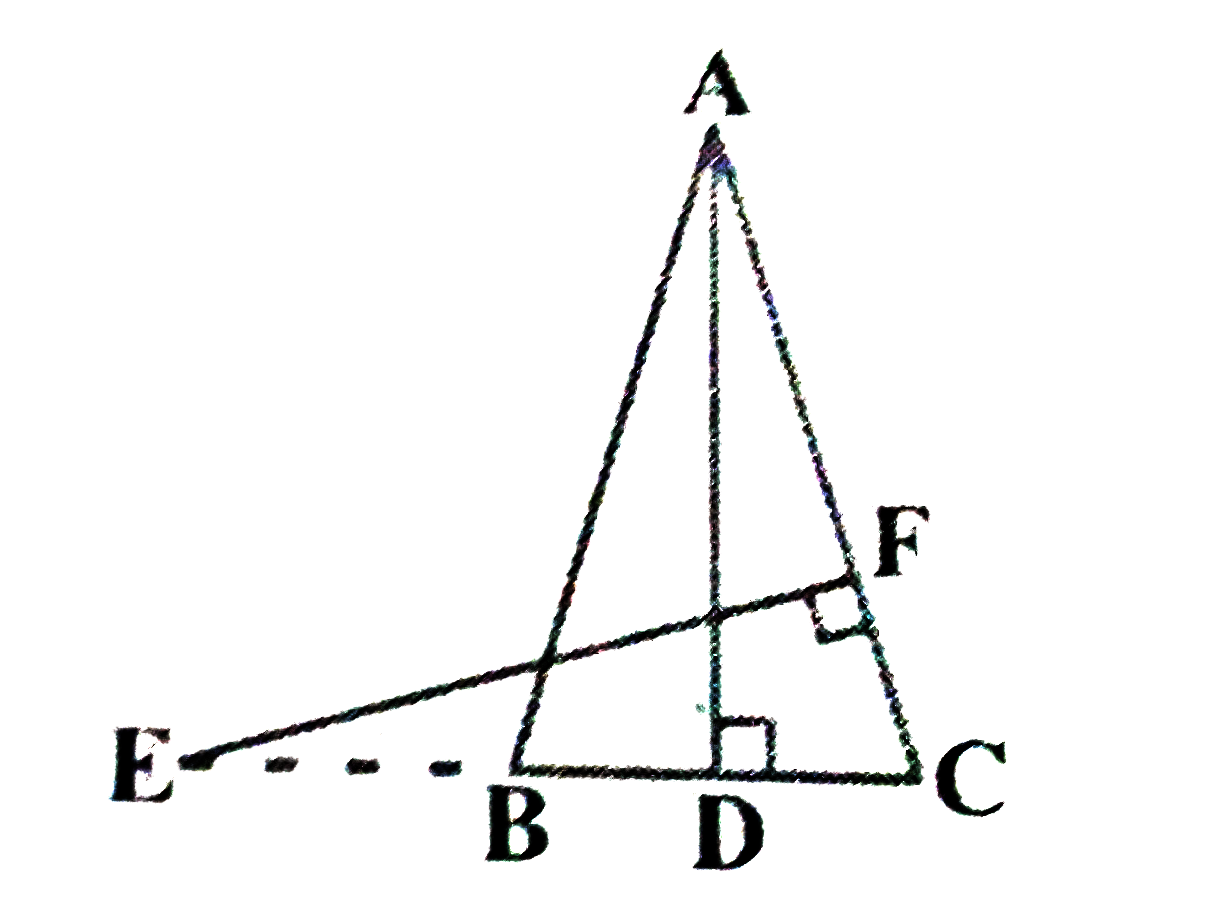
In figureE is a point on side CB produced of an isosceles △ABC with AB=AC. If AD⊥BCandEF⊥AC, prove thatΔABD~ΔECF.
 View Solution
View SolutionIn Fig. 4.124, E is a point on side CB produced of an isosceles triangle ABC with AB=AC . If AD⊥BC and EF⊥AC , prove that (i) △ABD∼△ECF (ii) AB×EF=AD×EC . (FIGURE)
View SolutionD and E are points on equal sides AB and AC of an isosceles triangle ABC such that AD=AE . Prove that B,C,D,E are concylic.
View SolutionIf D is the mid-point of the hypotenuse AC of a right triangle ABC, prove that BD=12AC
View SolutionAD and BE are respectively altitudes of an isosceles triangle ABC with AC=BC . Prove that AE=BD.
View SolutionIf ABC is an isosceles triangle and D is a point on BC such that AD⊥BC then,
View SolutionIn Figure, AD and BE are respectively altitudes of an isosceles triangle ABC with AC=BC. Prove that AE=BD
View SolutionA point D is on the side BC of an equilateral triangle ABC such that DC=14 BC . Prove that AD2=13 CD2 .
View SolutionBD and CE are bisectors of ∠B and ∠C of an isosceles ABC with AB=AC. Prove that BD=CE.
View SolutionIf D and E are points on sides AB and AC respectively of a triangle ABC such that DE∣∣BC and BD=CE . Prove that ABC is isosceles.
View Solution

