The perpendicular from the centre of a circle to a chord bisects the chord.
To prove that the perpendicular from the center of a circle to a chord bisects the chord, we can follow these steps:
1. Identify the Circle and Chord:
Let O be the center of the circle, and let AB be the chord of the circle. Let X be the point where the perpendicular from O meets the chord AB.
2. Draw the Perpendicular:
Draw the line segment OX such that it is perpendicular to the chord AB. This means that ∠OXA=∠OXB=90∘.
3. Consider Triangles:
We will consider the two triangles △OAX and △OBX.
4. Show Angles are Equal:
From the construction, we have:
- ∠OXA=90∘ (perpendicular)
- ∠OXB=90∘ (perpendicular)
5. Show Sides are Equal:
Since OA and OB are both radii of the circle, we have:
- OA=OB
6. Common Side:
The segment OX is common to both triangles, so:
- OX=OX
7. Apply the RHS Congruence Criterion:
Now we have:
- ∠OXA=∠OXB (both are 90∘)
- OA=OB (radii of the circle)
- OX=OX (common side)
By the RHS (Right angle-Hypotenuse-Side) congruence criterion, we can conclude that:
△OAX≅△OBX
8. Conclude that AX = BX:
Since the triangles are congruent, we can say that the corresponding parts are equal:
AX=BX
9. Conclusion:
Therefore, the perpendicular from the center O to the chord AB bisects the chord AB at point X.
Let O be the center of the circle, and let AB be the chord of the circle. Let X be the point where the perpendicular from O meets the chord AB.
Draw the line segment OX such that it is perpendicular to the chord AB. This means that ∠OXA=∠OXB=90∘.
We will consider the two triangles △OAX and △OBX.
From the construction, we have:
- ∠OXA=90∘ (perpendicular)
- ∠OXB=90∘ (perpendicular)
Since OA and OB are both radii of the circle, we have:
- OA=OB
The segment OX is common to both triangles, so:
- OX=OX
Now we have:
- ∠OXA=∠OXB (both are 90∘)
- OA=OB (radii of the circle)
- OX=OX (common side)
△OAX≅△OBX
Since the triangles are congruent, we can say that the corresponding parts are equal:
AX=BX
Therefore, the perpendicular from the center O to the chord AB bisects the chord AB at point X.
Topper's Solved these Questions
Similar Questions
The radius of a circle is 17-0 cm and the length of perpendicular drawn from its centre to a chord is 8 0 cm. Calculate the length of the chord.
View SolutionWhich of the following statements are true and which have are false? In each case give a valid reason for saying so p: Each radius of as circle is a chord of the circle q: The centre of a circle bisects each chord of the circle.
View Solution
Knowledge Check
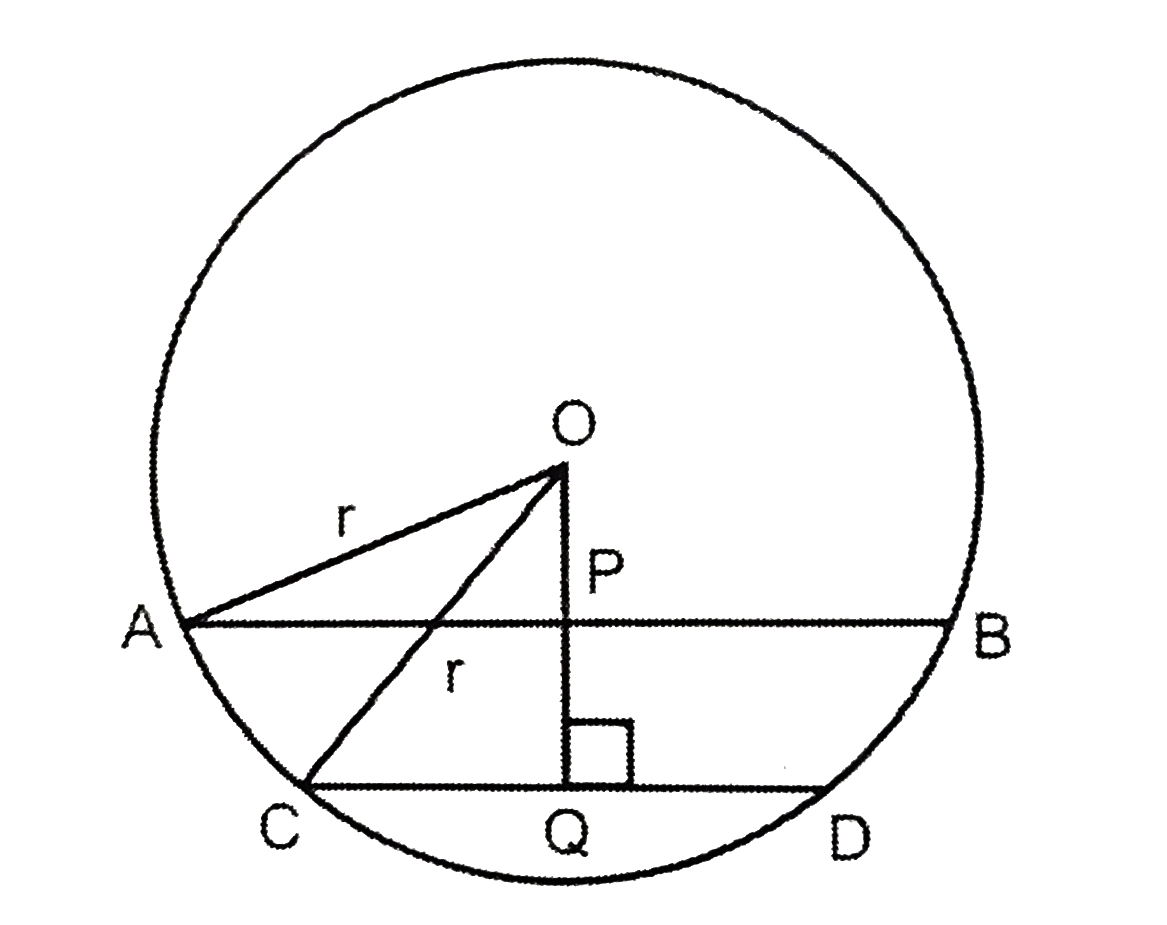
2 parallel chords 24 cm, and 18 cm, are on the same side of the centre of a circle. If the distance between the chords is 3 cm, calculate the radius of the circle.A15 cmB14 cmC13 cmD12 cm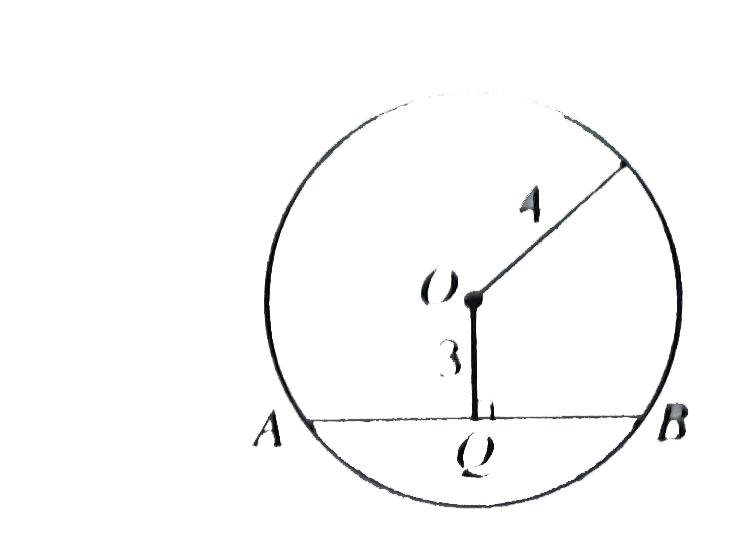
A chord ¯¯¯¯¯¯AB is 3 cm. from the center of a circle with radius 4. What is the length of chord ¯¯¯¯¯¯AB ?A√7B2√7C5D10
A chord of length 8 cm is drawn at a distance of 3 cm from the centre of a circle. Calculate the radius of the circle.
View SolutionFind the length of a chord which is at a distance of 5 cm from the centre of a circle of radius 10 cm.
View SolutionFind the length of a chord which is at a distance of 5 cm from the centre of a circle of radius 13 cm.
View Solution(Converse of Theorem 3) The line joining the centre of a circle to the mid-point of a chord is perpendicular to the chord.
View SolutionProve that the perpendicular bisector of a chord of a circle always passes through the centre.
View SolutionProve that the perpendicular bisector of a chord of a circle always passes through the centre.
View Solution