ABCD is a cyclic quadrilateral. ABandDC are produced to meet in E. Prove that EBC~EDA.
Step by step video & image solution for A B C D is a cyclic quadrilateral. A Ba n dD C are produced to meet in Edot Prove that E BC ~EDAdot by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
ABCD is a cyclic quadrilateral in which BA and CD when produced meet in E and EA=ED. Prove that: (i)ADisparalleltoBC (ii) EB=EC
View SolutionABCD is a cyclic quadrilateral in which AB and DC on being produced , meet at P such that PA = PD. Prove that AD is parallel to BC .
View SolutionABCD is a cyclic quadrilateral , Sides AB and DC produced meet at point E , whereas sides BC and AD produced meet at point F.
If ∠DCF:∠F:∠E=3:5:4 find the angles of the cyclic quadrilateral ABCD.
View SolutionIn Figure, ABCD is a cyclic quadrilateral. Find the value of a and b.
View SolutionIf ABCD is a cyclic quadrilateral in which AD∣∣BC . Prove that ∠B=∠C
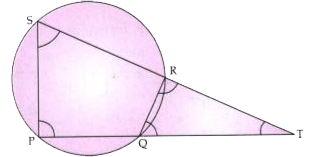
View SolutionIn the given figure PQRS is a cyclic quadrilateral PQ and SR produced meet at T.
(i) Prove ΔTPS~ΔTRQ.
View SolutionABCD is a cyclic quadrilateral whose diagonals ACandBD intersect at P . If AB=DC, Prove that : PAB≅PDC PA=PDandPC=PB ADllBC
View SolutionIn a cyclic quadrilateral ABCD , if ∠A=3∠C. Find ∠A
View SolutionThe sides BA and DC of a quadrilateral ABCD are produced as shown in Figure. Prove that a+b=x+y. Figure
View SolutionIn Figure, ABCD is a cyclic quadrilateral. A circle passing through A and B meets AD and BC in the points E and F respectively. Prove that EF∣∣DC
View Solution
