PQ and RS are two parallel chords of a circle whose centre is O and radius is 10 cm. If PQ=16 cm and RS=12 cm, find the distance between PQ and RS, if they lie: on the same side of centre O
To find the distance between the two parallel chords PQ and RS in a circle with a radius of 10 cm, we can follow these steps:
Step 1: Understand the Geometry
We have a circle with center O and radius 10 cm. The chords PQ and RS are parallel and lie on the same side of the center O. The lengths of the chords are given as PQ=16 cm and RS=12 cm.
Step 2: Draw the Circle and Chords
Draw a circle with center O. Draw the chords PQ and RS parallel to each other. Mark the midpoints of the chords as M (for RS) and N (for PQ).
Step 3: Apply the Perpendicular Bisector Property
Since OM and ON are perpendicular to the chords, we can use the property that a perpendicular from the center of a circle to a chord bisects the chord. Thus:
- RM=MS=RS2=122=6 cm
- PQ=16 cm, so NQ=PQ/2=162=8 cm
Step 4: Use the Pythagorean Theorem
In triangle OMS:
OM2+MS2=OS2
Substituting the known values:
OM2+62=102
OM2+36=100
OM2=100−36=64
OM=√64=8 cm
In triangle ONQ:
ON2+NQ2=OQ2
Substituting the known values:
ON2+82=102
ON2+64=100
ON2=100−64=36
ON=√36=6 cm
Step 5: Calculate the Distance Between the Chords
The distance MN between the two chords PQ and RS is given by:
MN=OM−ON=8 cm−6 cm=2 cm
Final Answer
The distance between the chords PQ and RS is 2 cm.
---
We have a circle with center O and radius 10 cm. The chords PQ and RS are parallel and lie on the same side of the center O. The lengths of the chords are given as PQ=16 cm and RS=12 cm.
Draw a circle with center O. Draw the chords PQ and RS parallel to each other. Mark the midpoints of the chords as M (for RS) and N (for PQ).
Since OM and ON are perpendicular to the chords, we can use the property that a perpendicular from the center of a circle to a chord bisects the chord. Thus:
- RM=MS=RS2=122=6 cm
- PQ=16 cm, so NQ=PQ/2=162=8 cm
In triangle OMS:
OM2+MS2=OS2
Substituting the known values:
OM2+62=102
OM2+36=100
OM2=100−36=64
OM=√64=8 cm
ON2+NQ2=OQ2
Substituting the known values:
ON2+82=102
ON2+64=100
ON2=100−64=36
ON=√36=6 cm
The distance MN between the two chords PQ and RS is given by:
MN=OM−ON=8 cm−6 cm=2 cm
The distance between the chords PQ and RS is 2 cm.
Topper's Solved these Questions
Similar Questions
PQ and RS are two parallel chords of a circle whose centre is O and radius is 10cm. If PQ=16cm and RS=12cm, find the distance between PQ and RS, if they lie: on the same side of the centre O. on opposite side of the centre O.
View SolutionPQ and RS are two parallel chords of a circle and lines RP and SQ intersect each other at O as shown in Figure. Prove that OP=OQ
View SolutionAB and CD are two parallel chords of a circle with centre O such that AB=6 cm and CD=12 cm. The chords are on the same side of the centre and the distance between them is 3 cm. The radius of the circle is (a)6 cm (b) 5 √2 cm (c) 7 cm (d) 3 √5 cm
View SolutionPQ and RS are two parallel chords of a circle and lines RP and SQ intersect each other at O as shown in Figure. Prove that OP=OQ.
View SolutionIf the tangent at a point P to a circle with centre O cuts a line through O at Q such that PQ=24cm and OQ=25cm . Find the radius of the circle.
View SolutionFind the coordinates of points P, Q, R and S in Figure.
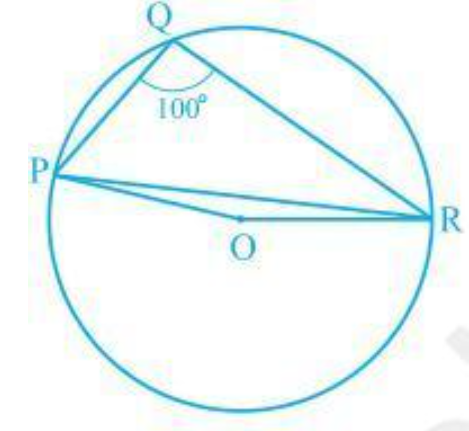
View Solution∠PQR=100∘, where P, Q and R are points on a circle with centre O. Find ∠OPR
View SolutionIn Figure, O is the centre of the circle of radius 5 cm.OP⊥AB, OQ⊥CD, ABCD, AB=6 cm and CD=8 cm. Determine PQ
View SolutionPQRS is a rectangle inscribed in a quadrant of a circle of radius 13cm. A is any point on PQ. If PS=5cm, then find ar ( RAS)
View SolutionIn triangle PQR, if PQ=RQ and L, M and N are the mid-points of the sides PQ, QR and RP respectively. Prove that LN=MN.
View Solution
