Question

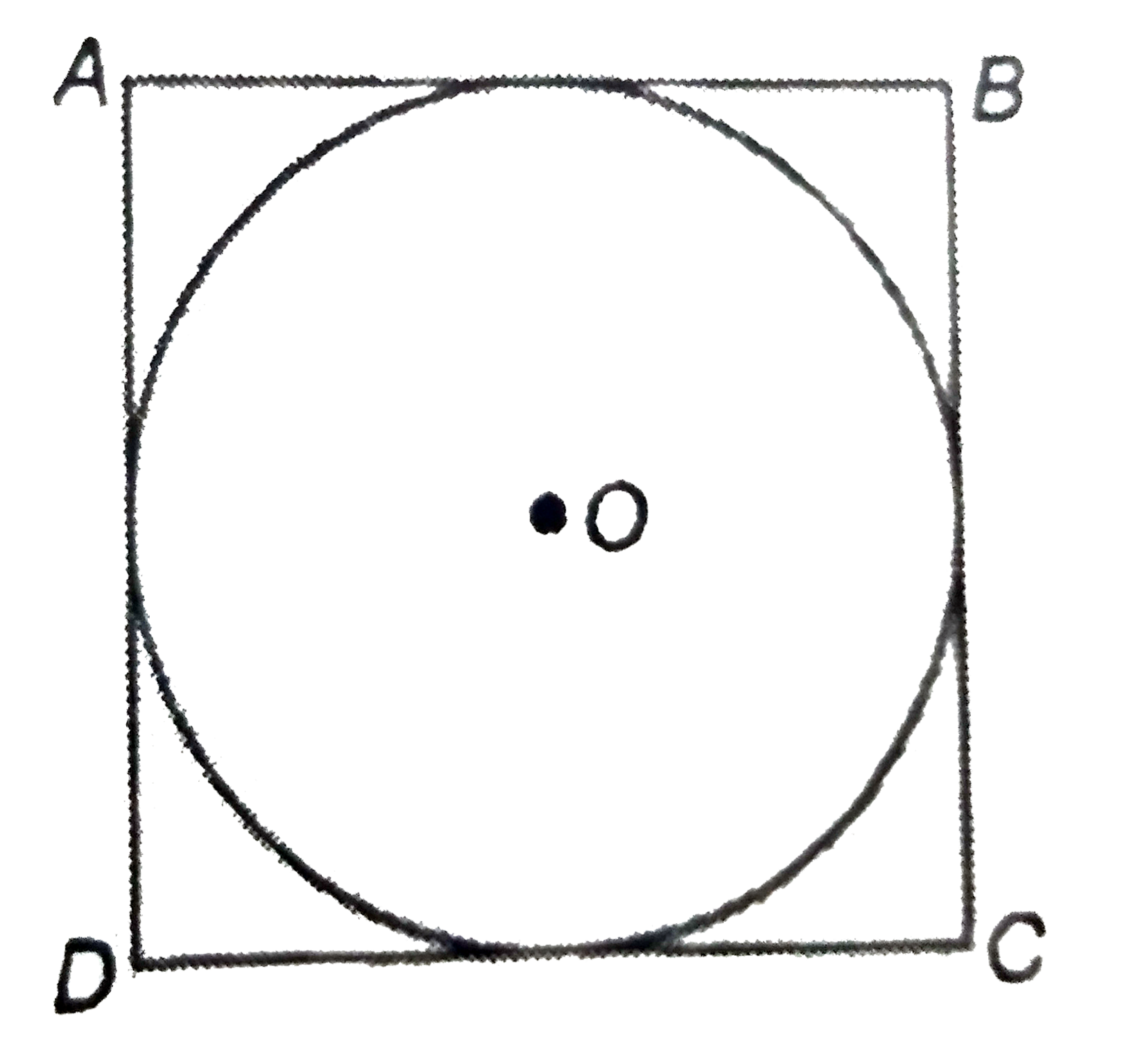
Figure, ABCD is a square of side 2a. Find the ratio between
the circumferences and
the areas of the incircle and the circum-circle of the square.

Explanation

To solve the problem, we need to find the ratio between the circumferences and the areas of the incircle and the circumcircle of a square ABCD with side length $$2a$$.

Step 1: Find the radius of the incircle.
The incircle of a square touches the midpoint of each side. Therefore, the diameter of the incircle is equal to the side length of the square.

- Given side length of the square \( = 2a\)
- Radius of the incircle \( r_{in} = \frac{2a}{2} = a\)

Step 2: Find the radius of the circumcircle.
The circumcircle of a square has its diameter equal to the diagonal of the square. We can find the diagonal using the Pythagorean theorem.

- The diagonal \(d\) of the square can be calculated as:
\( d = \sqrt{(2a)^2 + (2a)^2} = \sqrt{4a^2 + 4a^2} = \sqrt{8a^2} = 2\sqrt{2}a \)
- Therefore, the radius of the circumcircle \( r_{circ} = \frac{d}{2} = \frac{2\sqrt{2}a}{2} = \sqrt{2}a\)

Step 3: Calculate the circumferences of the incircle and circumcircle.
- Circumference of the incircle \(C_{in} = 2\pi r_{in} = 2\pi a\)
- Circumference of the circumcircle \(C_{circ} = 2\pi r_{circ} = 2\pi (\sqrt{2}a) = 2\pi\sqrt{2}a\)

Step 4: Find the ratio of the circumferences.
The ratio of the circumferences of the incircle to the circumcircle is:
\( \text{Ratio of circumferences} = \frac{C_{in}}{C_{circ}} = \frac{2\pi a}{2\pi\sqrt{2}a} = \frac{1}{\sqrt{2}} \)

Step 5: Calculate the areas of the incircle and circumcircle.
- Area of the incircle \(A_{in} = \pi r_{in}^2 = \pi a^2\)
- Area of the circumcircle \(A_{circ} = \pi r_{circ}^2 = \pi (\sqrt{2}a)^2 = \pi (2a^2) = 2\pi a^2\)

Step 6: Find the ratio of the areas.
The ratio of the areas of the incircle to the circumcircle is:
\( \text{Ratio of areas} = \frac{A_{in}}{A_{circ}} = \frac{\pi a^2}{2\pi a^2} = \frac{1}{2} \)

Final Result:
- The ratio of the circumferences of the incircle to the circumcircle is \(1 : \sqrt{2}\).
- The ratio of the areas of the incircle to the circumcircle is \(1 : 2\).