Question

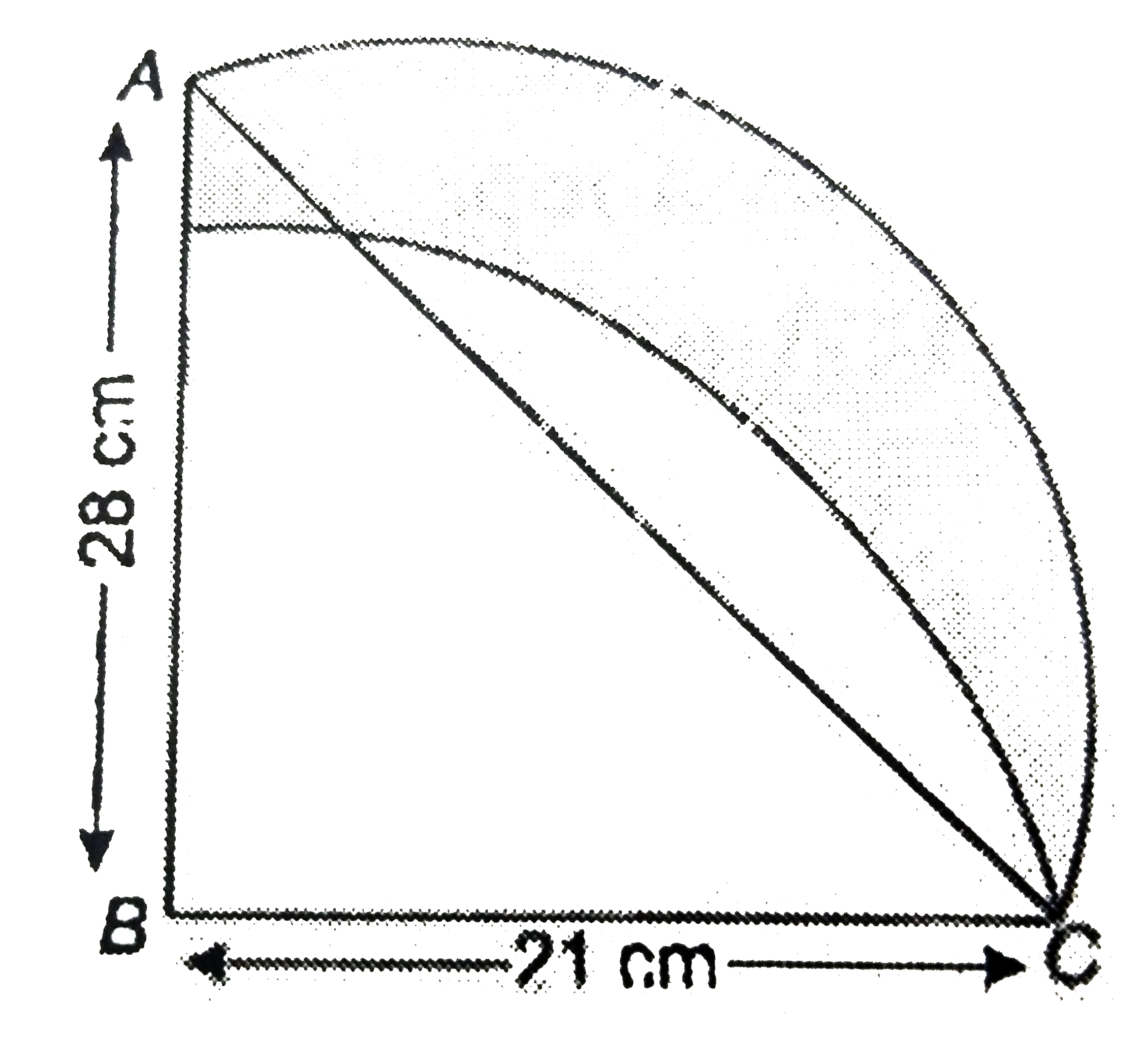
In Figure, ABC is a right-angled triangle, $\angle B={90}^0$,AB=28 cm
and BC=21 cm
With AC as diameter a semicircle is drawn and with BC as radius a
quarter circle is drawn. Find the area of the shaded region correct to two
decimal places.

Explanation

To find the area of the shaded region in the given problem, we will follow these steps:

Step 1: Calculate the area of triangle ABC
Triangle ABC is a right-angled triangle with:
- AB (height) = 28 cm
- BC (base) = 21 cm

The area of a triangle is given by the formula:
\( \text{Area of triangle} = \frac{1}{2} \times \text{base} \times \text{height} \)
Substituting the values:
\( \text{Area of triangle ABC} = \frac{1}{2} \times 21 \times 28 = \frac{1}{2} \times 588 = 294 \text{ cm}^2 \)

Step 2: Calculate the length of AC (diameter of the semicircle)
Using the Pythagorean theorem:
\( AC^2 = AB^2 + BC^2 \)
Substituting the values:
\( AC^2 = 28^2 + 21^2 = 784 + 441 = 1225 \)
Taking the square root:
\( AC = \sqrt{1225} = 35 \text{ cm} \)

Step 3: Calculate the radius of the semicircle
The radius \( r_1 \) of the semicircle is half of the diameter AC:
\( r_1 = \frac{AC}{2} = \frac{35}{2} = 17.5 \text{ cm} \)

Step 4: Calculate the area of the semicircle
The area of a semicircle is given by:
\( \text{Area of semicircle} = \frac{1}{2} \pi r_1^2 \)
Substituting the value of \( r_1 \):
\( \text{Area of semicircle} = \frac{1}{2} \pi \left(17.5\right)^2 = \frac{1}{2} \pi \times 306.25 = \frac{306.25\pi}{2} \approx 480.57 \text{ cm}^2 \quad (\text{using } \pi \approx 3.14) \)

Step 5: Calculate the radius of the quarter circle
The radius \( r_2 \) of the quarter circle is equal to BC:
\( r_2 = 21 \text{ cm} \)

Step 6: Calculate the area of the quarter circle
The area of a quarter circle is given by:
\( \text{Area of quarter circle} = \frac{1}{4} \pi r_2^2 \)
Substituting the value of \( r_2 \):
\( \text{Area of quarter circle} = \frac{1}{4} \pi (21)^2 = \frac{1}{4} \pi \times 441 = \frac{441\pi}{4} \approx 346.36 \text{ cm}^2 \quad (\text{using } \pi \approx 3.14) \)

Step 7: Calculate the area of the shaded region
The area of the shaded region is given by:
\( \text{Area of shaded region} = \text{Area of triangle ABC} + \text{Area of semicircle} - \text{Area of quarter circle} \)
Substituting the calculated areas:
\( \text{Area of shaded region} = 294 + 480.57 - 346.36 \approx 428.21 \text{ cm}^2 \)

Final Answer
The area of the shaded region is approximately:
\( \text{Area of shaded region} \approx 428.21 \text{ cm}^2 \)