Three vectors of magnitude meet in a point and their directions are along the diagonals of the adjacent faces of a cube. Determine their resultant.
To solve the problem of finding the resultant of three vectors with magnitudes a,2a, and 3a that meet at a point and are directed along the diagonals of the adjacent faces of a cube, we can follow these steps:
Step 1: Understand the Directions of the Vectors
The vectors are directed along the diagonals of the adjacent faces of a cube. Let's denote the vectors as:
- →A along the diagonal of face OABC
- →B along the diagonal of face ODEF
- →C along the diagonal of face OEFG
Step 2: Define the Unit Vectors
Assuming the cube has a side length of b, we can define the unit vectors along the diagonals:
- The diagonal →A can be represented as:
→A=a(1√2^i+1√2^j)
- The diagonal →B can be represented as:
→B=2a(1√2^j+1√2^k)
- The diagonal →C can be represented as:
→C=3a(1√2^k+1√2^i)
Step 3: Write the Vectors in Component Form
Now we can express the vectors in component form:
- →A=a√2^i+a√2^j
- →B=2a√2^j+2a√2^k
- →C=3a√2^k+3a√2^i
Step 4: Add the Vectors to Find the Resultant
The resultant vector →R is the sum of the three vectors:
→R=→A+→B+→C
Substituting the component forms:
→R=(a√2+3a√2)^i+(a√2+2a√2)^j+(2a√2+3a√2)^k
This simplifies to:
→R=4a√2^i+3a√2^j+5a√2^k
Step 5: Calculate the Magnitude of the Resultant Vector
The magnitude of the resultant vector →R is given by:
|→R|=
⎷(4a√2)2+(3a√2)2+(5a√2)2
Calculating each term:
|→R|=√16a22+9a22+25a22=√(16+9+25)a22=√50a22=√25a2=5a
Final Result
The magnitude of the resultant vector is:
5a
The vectors are directed along the diagonals of the adjacent faces of a cube. Let's denote the vectors as:
- →A along the diagonal of face OABC
- →B along the diagonal of face ODEF
- →C along the diagonal of face OEFG
Assuming the cube has a side length of b, we can define the unit vectors along the diagonals:
- The diagonal →A can be represented as:
→A=a(1√2^i+1√2^j)
- The diagonal →B can be represented as:
→B=2a(1√2^j+1√2^k)
- The diagonal →C can be represented as:
→C=3a(1√2^k+1√2^i)
Now we can express the vectors in component form:
- →A=a√2^i+a√2^j
- →B=2a√2^j+2a√2^k
- →C=3a√2^k+3a√2^i
The resultant vector →R is the sum of the three vectors:
→R=→A+→B+→C
Substituting the component forms:
→R=(a√2+3a√2)^i+(a√2+2a√2)^j+(2a√2+3a√2)^k
This simplifies to:
→R=4a√2^i+3a√2^j+5a√2^k
The magnitude of the resultant vector →R is given by:
|→R|=
⎷(4a√2)2+(3a√2)2+(5a√2)2
Calculating each term:
|→R|=√16a22+9a22+25a22=√(16+9+25)a22=√50a22=√25a2=5a
The magnitude of the resultant vector is:
5a
Topper's Solved these Questions
Similar Questions
Three coinitial vectors of magnitudes a, 2a and 3a meet at a point and their directions are along the diagonals if three adjacent faces if a cube. Determined their resultant R. Also prove that the sum of the three vectors determinate by the diagonals of three adjacent faces of a cube passing through the same corner, the vectors being directed from the corner, is twice the vector determined by the diagonal of the cube.
View SolutionThree vector each of magnitude A are acting at a point such that angle between any two consecuitve vectors in same plane is 60∘ The magniude of their resultant is
View Solution
Knowledge Check
There are n coplanar vectors each of magnitude m and each vector is inclined to the preceeding vector at an angle 2π/n. Then the magnitude of their resultant is
An mBm2Cm/nDzero
Write a vector of magnitude of 18 units in the direction of the vector ˆi−2ˆj−2ˆk.
View SolutionWrite a vector of magnitude 9 units in the direction of vector −2ˆi+ˆj+2ˆk
View SolutionTwo vectors of equal magnitude are acting through a point. The magnitude of resultant is equal to the magnitude of either vectoe. Find the angle between the vectors.
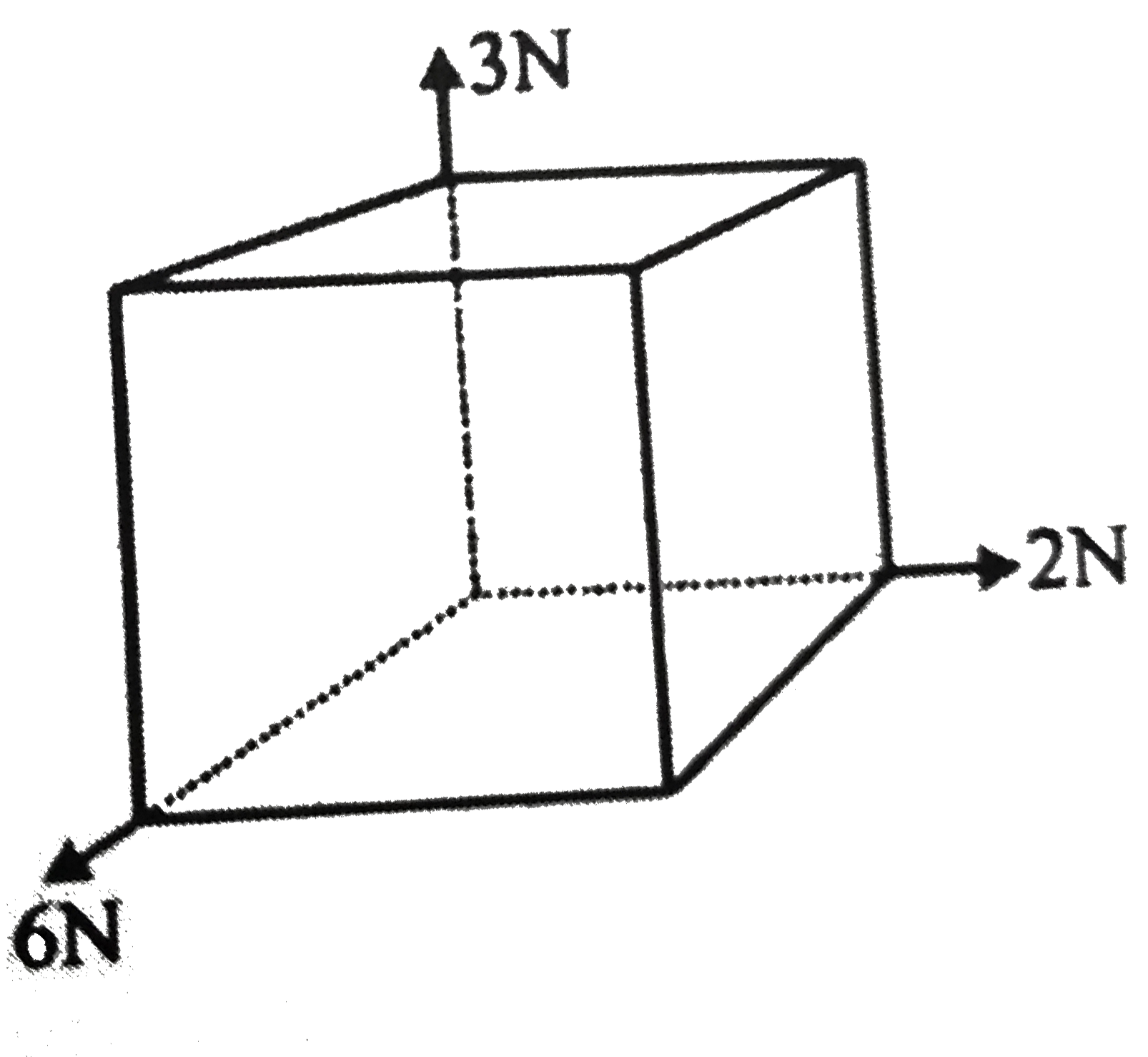
View SolutionThree forces of magnitudes 2N, 3N and 6N act at corners of a cube along three sides as shown in figure. Find the resultant of these forces in N.
View SolutionTwo vectors having equal magnitudes A make an angle θ with eahc other. Find the magnitude and direction of the resultant.
View SolutionTwo vectors having equal magnitudes A make an angle θ with each other. Find the magnitude and direction of the resultant.
View SolutionWrite a vector of magnitude of 18 units in the direction of the vector ˆi−2ˆj−2ˆk .
View Solution
