Question

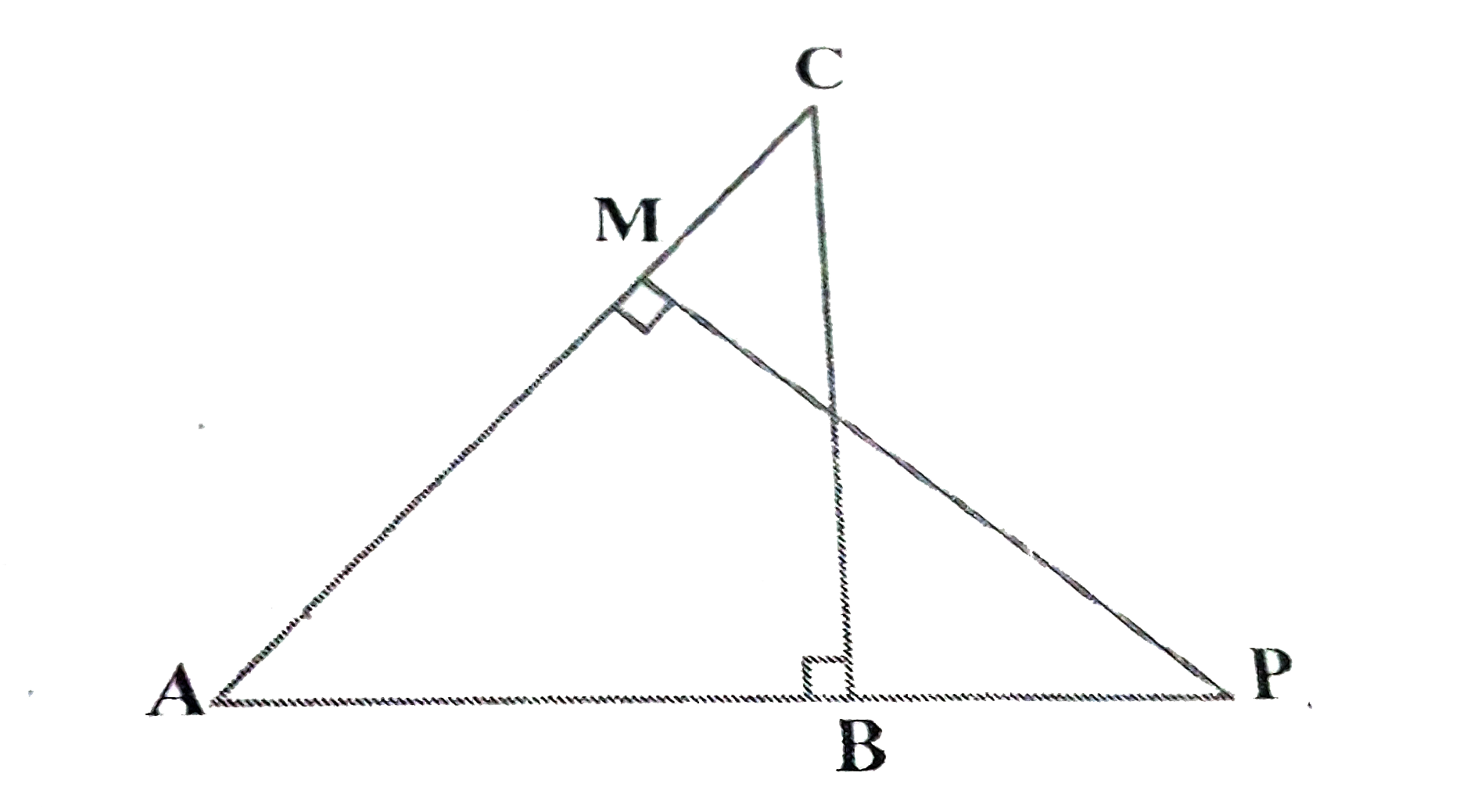
If $ABC$
and $AMP$
are two
right triangles, right angled at $B$
and $M$
respectively
such that $\angle MAP=\angle BAC$
. Prove
that
$\frac{CA}{PA}=\frac{BC}{MP}$

Explanation

To prove that $$\frac{CA}{PA}=\frac{BC}{MP}$$, we will use the properties of similar triangles.

1. Identify the Triangles: We have two right triangles, \( \triangle ABC \) and \( \triangle AMP \). Triangle \( ABC \) is right-angled at \( B \) and triangle \( AMP \) is right-angled at \( M \).

2. Given Angles: We are given that \(\angle MAP = \angle BAC\). This means that these two angles are equal.

3. Right Angles: Since triangle \( ABC \) is right-angled at \( B \), we have \(\angle ABC = 90^\circ\). Similarly, since triangle \( AMP \) is right-angled at \( M \), we have \(\angle AMP = 90^\circ\).

4. Common Angle: We also have the common angle \( \angle A \) in both triangles, which is the angle at vertex \( A \).

5. Angle-Angle (AA) Criterion: Since we have two angles in triangle \( ABC \) that are equal to two angles in triangle \( AMP \) (i.e., \(\angle A = \angle A\), \(\angle ABC = \angle AMP\), and \(\angle MAP = \angle BAC\)), we can conclude that the triangles are similar by the Angle-Angle (AA) criterion.

6. Proportional Sides: From the similarity of the triangles, we know that the corresponding sides are proportional. This gives us the relationship:
\( \frac{CA}{PA} = \frac{BC}{MP} \)

7. Conclusion: Therefore, we have proved that:
\( \frac{CA}{PA} = \frac{BC}{MP} \)