In figure,
are opposite rays:
(i) If
what is the value of ?
(ii) If
what is the value of ?
To solve the problem, we need to analyze the angles formed by the opposite rays OA and OB. Let's break it down step by step.
Step 1: Understand the relationship of angles
Since OA and OB are opposite rays, the angle AOB forms a straight line. Therefore, the measure of angle AOB is 180 degrees.
Step 2: Set up the equation
From the problem, we know:
- Angle AOC = 2y + 5 degrees
- Angle COB = 3x degrees
Since angle AOB is the sum of angles AOC and COB, we can write the equation:
Angle AOB=Angle AOC+Angle COB
This gives us:
180=(2y+5)+(3x)
Rearranging this, we get:
2y+3x+5=180
Subtracting 5 from both sides:
2y+3x=175
Let’s call this Equation 1.
Step 3: Solve for (i) when x=25∘
Substituting x=25∘ into Equation 1:
2y+3(25)=175
This simplifies to:
2y+75=175
Subtracting 75 from both sides:
2y=175−75
2y=100
Dividing both sides by 2:
y=50∘
Step 4: Solve for (ii) when y=35∘
Now substituting y=35∘ into Equation 1:
2(35)+3x=175
This simplifies to:
70+3x=175
Subtracting 70 from both sides:
3x=175−70
3x=105
Dividing both sides by 3:
x=35∘
Final Answers
(i) If x=25∘, then y=50∘.
(ii) If y=35∘, then x=35∘.
Since OA and OB are opposite rays, the angle AOB forms a straight line. Therefore, the measure of angle AOB is 180 degrees.
From the problem, we know:
- Angle AOC = 2y + 5 degrees
- Angle COB = 3x degrees
Angle AOB=Angle AOC+Angle COB
This gives us:
180=(2y+5)+(3x)
Rearranging this, we get:
2y+3x+5=180
Subtracting 5 from both sides:
2y+3x=175
Let’s call this Equation 1.
Substituting x=25∘ into Equation 1:
2y+3(25)=175
This simplifies to:
2y+75=175
Subtracting 75 from both sides:
2y=175−75
2y=100
Dividing both sides by 2:
y=50∘
Now substituting y=35∘ into Equation 1:
2(35)+3x=175
This simplifies to:
70+3x=175
Subtracting 70 from both sides:
3x=175−70
3x=105
Dividing both sides by 3:
x=35∘
(i) If x=25∘, then y=50∘.
Topper's Solved these Questions
Similar Questions
Knowledge Check
In the figure, what is the value of y if x:y=2:3?
 A16B32C48D54
A16B32C48D54If 0.25y+0.36=0.33y−1.48, what is the value of y10?
A2.30B1.40C0.75D0.64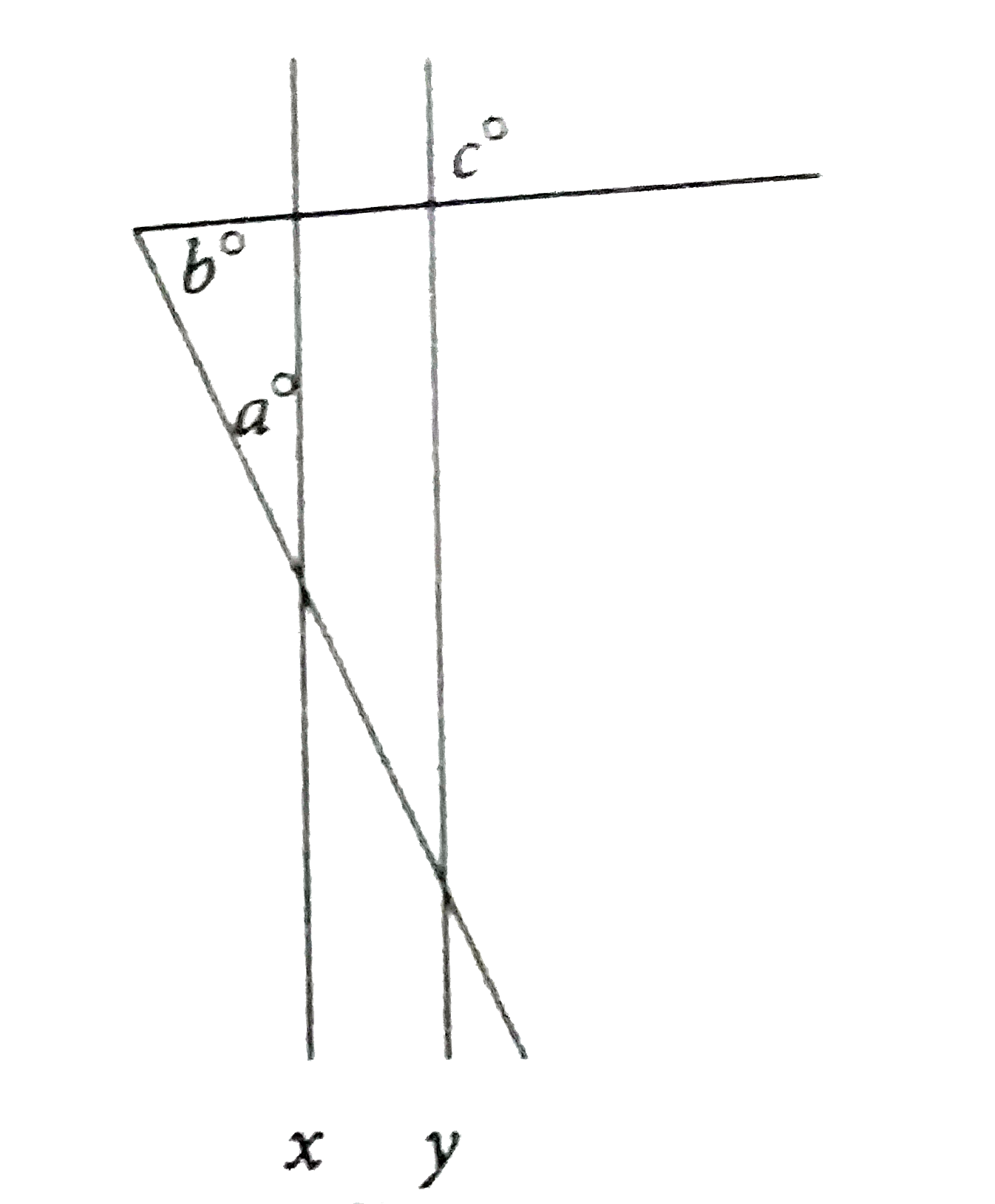
In the figure above, x∣∣y. What is the value of a?Ab+cB2b-cC180-b+cD180-b-c
In Fig. 7, OA and OB are opposite rays: (i) If x=75 find the value of y (ii) if y= 110 find the bvalue of x
View SolutionIn Fig, OA, OB are opposite rays and ∠AOC+ ∠BOD=900, Find ∠COD.
View SolutionIn Figure, O is the centre of the circle. If ∠BOD=160∘, find the values of x and y
View SolutionIn Figure, O is the centre of the circle. If ∠CEA=300, find the values of x, y and z
View SolutionIf x2+y2=29 and xy=2, find the value of
(i)x+y
(ii) x−y
View Solution
