In Fig.23, OA and OB are opposite rays: (i) If x=25 what is the value of y ? (ii) If y=35 what is the value of x ?
To solve the question step by step, we will break down both parts of the question regarding the angles formed by the opposite rays OA and OB.
Given Information:
- OA and OB are opposite rays.
- The angles formed are 2y+5 and 3x.
- The sum of angles on a straight line is 180∘.
Part (i): If x=25∘, what is the value of y?
1. Substitute the value of x:
x=25∘
Therefore, 3x=3×25=75∘.
2. Set up the equation:
The sum of the angles is:
2y+5+3x=180∘
Substituting 3x:
2y+5+75=180
3. Simplify the equation:
Combine like terms:
2y+80=180
4. Isolate 2y:
Subtract 80 from both sides:
2y=180−80
2y=100
5. Solve for y:
Divide both sides by 2:
y=1002=50∘
Part (ii): If y=35∘, what is the value of x?
1. Substitute the value of y:
y=35∘
Therefore, 2y=2×35=70∘.
2. Set up the equation:
The sum of the angles is:
2y+5+3x=180∘
Substituting 2y:
70+5+3x=180
3. Simplify the equation:
Combine like terms:
75+3x=180
4. Isolate 3x:
Subtract 75 from both sides:
3x=180−75
3x=105
5. Solve for x:
Divide both sides by 3:
x=1053=35∘
Final Answers:
(i) If x=25∘, then y=50∘.
(ii) If y=35∘, then x=35∘.
- OA and OB are opposite rays.
- The angles formed are 2y+5 and 3x.
- The sum of angles on a straight line is 180∘.
x=25∘
Therefore, 3x=3×25=75∘.
The sum of the angles is:
2y+5+3x=180∘
Substituting 3x:
2y+5+75=180
Combine like terms:
2y+80=180
Subtract 80 from both sides:
2y=180−80
2y=100
Divide both sides by 2:
y=1002=50∘
y=35∘
Therefore, 2y=2×35=70∘.
The sum of the angles is:
2y+5+3x=180∘
Substituting 2y:
70+5+3x=180
Combine like terms:
75+3x=180
Subtract 75 from both sides:
3x=180−75
3x=105
Divide both sides by 3:
x=1053=35∘
(i) If x=25∘, then y=50∘.
(ii) If y=35∘, then x=35∘.
Topper's Solved these Questions
Similar Questions
Knowledge Check
If x−y=3andx+y=5, what is the value of y?
A−4B−2C−1D1If x+2yy=5, what is the value of yx ?
A−3B−13C13D3If 4x−2y=10and7x+2y=23,what is the value of x?
A13B1C3D13
In Fig. 7, OA and OB are opposite rays: (i) If x=75 find the value of y (ii) if y= 110 find the bvalue of x
View SolutionDiagram (i) shows the curve y=logax. What is the value of a?
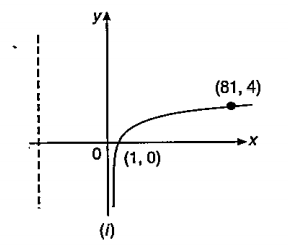 .
.
View SolutionIf x−3yx=7, what is the value of xy?
View SolutionIf x3=4 and x+y=32, what is the value of x-y?
View SolutionIf x+3x−3=y , what is the value of x in terms of y?
View Solution
