In the given figure , ∠Qgt∠R, PA is the bisector of ∠QPR and PM⊥ QR.
Prove that ∠APM = 1/2(∠Q-∠R).
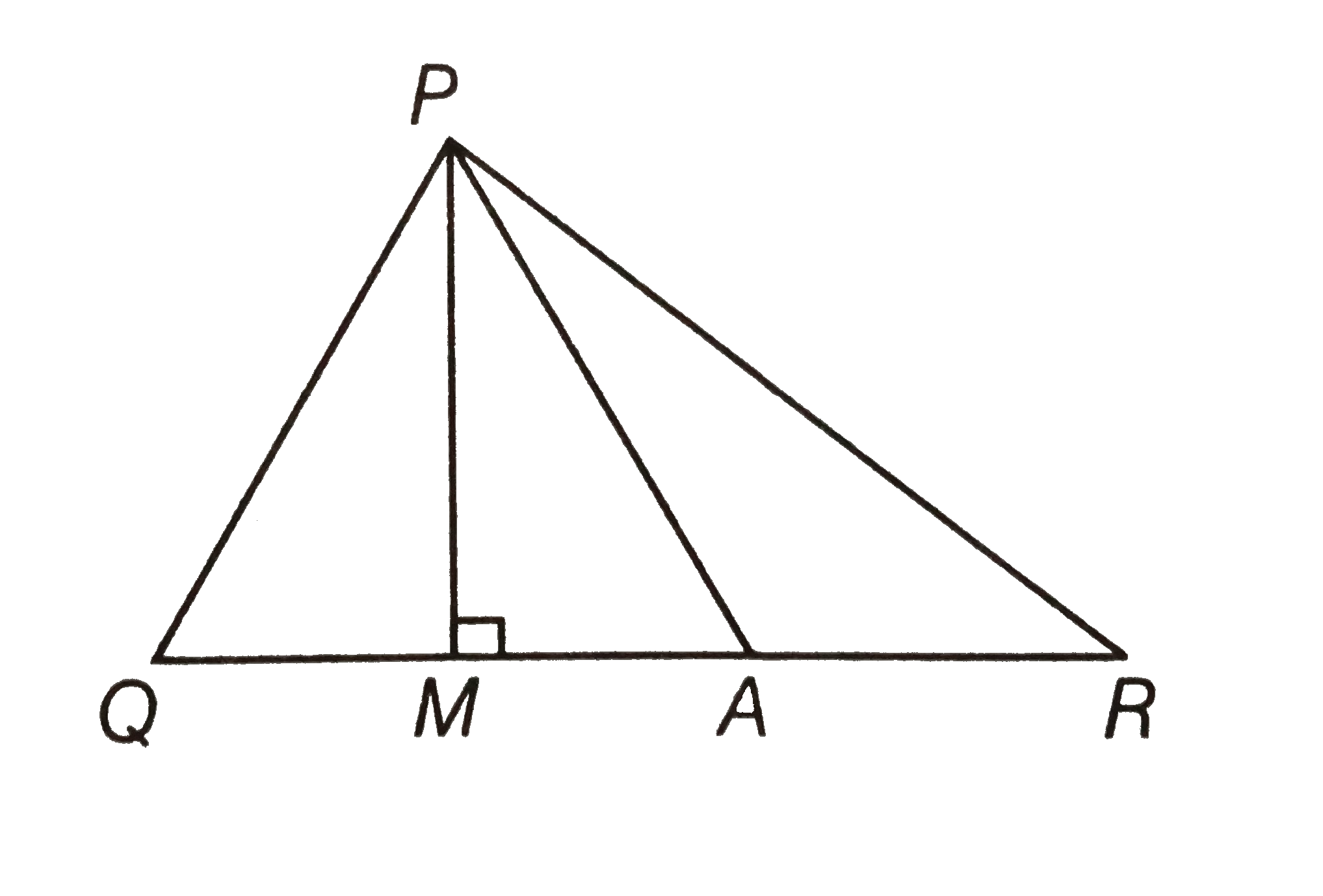
Step by step video & image solution for In the given figure , /_Qgt/_R, PA is the bisector of /_QPR and PMbot QR. Prove that /_APM = 1/2(/_Q-/_R). by Maths experts to help you in doubts & scoring excellent marks in Class 9 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
If PS is the bisector of ∠QPR and PT⊥QR . Show that ∠TPS=12(∠Q−∠R).
View SolutionIn figure PS is the bisector of ∠QPRof ΔPQR. Prove that QSSR=PQPR.

View SolutionProve that r1+r21=2R
View SolutionIn the given figure, QR is parallel to AB and DR is parallel to QB.
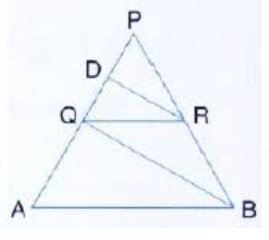
Prove that : PQ2=PD×PA.
View SolutionIn Fig. , the side QR of △ PQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR=12∠QPR.

View SolutionIn Fig. 6.44, the side QR of PQR is produced to a point S. If the bisectors of ∠PQR\ and∠PRS meet at point T, then prove that ∠QTR=12∠QPR .
View SolutionProve that r1+r2+r3−r=4R
View SolutionProve that r1+r2+r3−r=4R
View SolutionIn Figure, PQ>PR.QS and RS are the bisectors of ∠Q and ∠R respectively. Prove that SQ>SR.
View SolutionIn Figure, ∠PQR= ∠PRQ, then prove that ∠PQS= ∠PRT.
View Solution
