Question

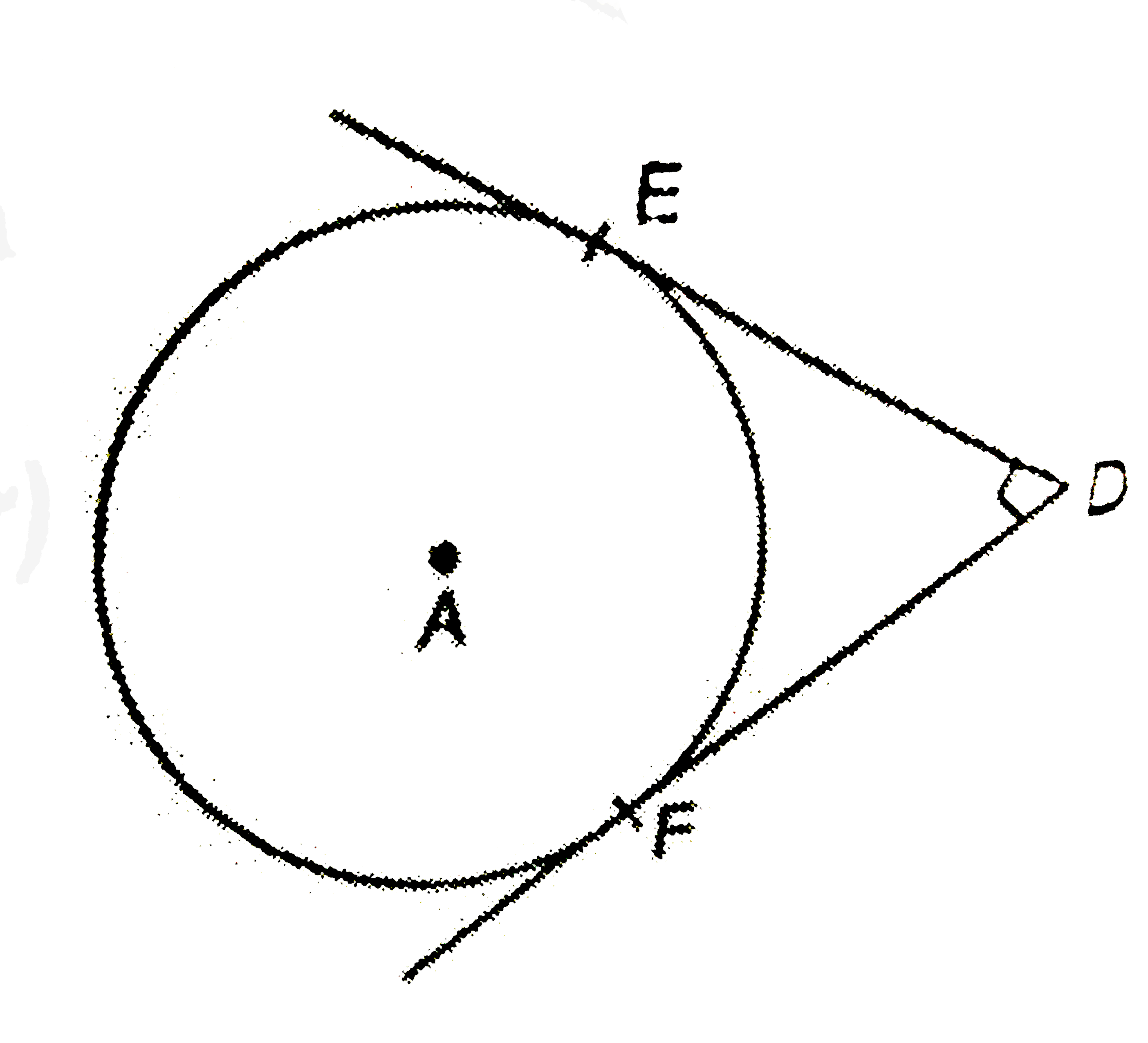
In Fig.
10.99, $DE$
and $DF$
are
tangents from an external point $D$
to a circle
with centre $A$
. If $DE=5cm$
and $DE\perp DF$
, then the
radius of the circle is (FIGURE)
(a)
3cm (b) 5cm (c) 4cm (d) 6cm

Explanation

To solve the problem step by step, we will follow the reasoning provided in the video transcript and apply the relevant geometric principles.

1. Identify the Given Information:
- We have a circle with center \( A \).
- \( DE \) and \( DF \) are tangents from point \( D \) to the circle.
- The length of \( DE = 5 \) cm.
- It is given that \( DE \perp DF \).

2. Draw the Radius to the Point of Contact:
- Let \( E \) be the point of contact of tangent \( DE \) with the circle.
- Let \( F \) be the point of contact of tangent \( DF \) with the circle.
- Draw the radius \( AE \) from the center \( A \) to point \( E \) and the radius \( AF \) from the center \( A \) to point \( F \).

3. Apply the Tangent-Radius Theorem:
- According to the theorem, the tangent at any point of a circle is perpendicular to the radius drawn to the point of contact.
- Therefore, \( AE \perp DE \) and \( AF \perp DF \).

4. Analyze the Angles:
- Since \( DE \perp DF \) (given), we have a right angle at point \( D \).
- This implies that angles \( AED \), \( ADF \), and \( EDF \) are all right angles (90 degrees).

5. Determine the Shape:
- Since all angles \( AED \), \( EDF \), and \( ADF \) are 90 degrees, quadrilateral \( AEDF \) is a square.
- In a square, all sides are equal.

6. Relate the Lengths:
- Since \( DE = 5 \) cm, and all sides of the square are equal, we have:
\( AE = DE = DF = AF = 5 \text{ cm} \)

7. Conclusion:
- The radius of the circle, which is \( AE \) or \( AF \), is therefore:
\( \text{Radius of the circle} = 5 \text{ cm} \)

Final Answer:
The radius of the circle is \( 5 \) cm.