A regular hexagon is inscribed the circle. If the area of hexagon is 24√3 cm2 , find the area of the circle. (Use π=3.14)
To find the area of the circle inscribed around a regular hexagon with a given area, we can follow these steps:
1. Understand the Relationship Between the Hexagon and the Circle:
A regular hexagon can be divided into 6 equilateral triangles. The radius of the circle inscribed around the hexagon is equal to the side length of the hexagon.
2. Use the Formula for the Area of a Regular Hexagon:
The area A of a regular hexagon can be calculated using the formula:
A=3√32a2
where a is the length of a side of the hexagon.
3. Set Up the Equation with the Given Area:
We know the area of the hexagon is 24√3cm2. Therefore, we can set up the equation:
3√32a2=24√3
4. Simplify the Equation:
To eliminate √3 from both sides, we divide by √3:
32a2=24
5. Solve for a2:
Multiply both sides by 23:
a2=24×23=16
6. Find the Side Length a:
Taking the square root of both sides gives:
a=√16=4cm
7. Determine the Radius of the Circle:
Since the radius r of the circle is equal to the side length a of the hexagon:
r=4cm
8. Calculate the Area of the Circle:
The area Ac of the circle is given by the formula:
Ac=πr2
Substituting π=3.14 and r=4:
Ac=3.14×(42)=3.14×16
9. Perform the Multiplication:
Ac=3.14×16=50.24cm2
Final Answer:
The area of the circle is 50.24cm2.
---
A regular hexagon can be divided into 6 equilateral triangles. The radius of the circle inscribed around the hexagon is equal to the side length of the hexagon.
The area A of a regular hexagon can be calculated using the formula:
A=3√32a2
where a is the length of a side of the hexagon.
We know the area of the hexagon is 24√3cm2. Therefore, we can set up the equation:
3√32a2=24√3
To eliminate √3 from both sides, we divide by √3:
32a2=24
Multiply both sides by 23:
a2=24×23=16
Taking the square root of both sides gives:
a=√16=4cm
Since the radius r of the circle is equal to the side length a of the hexagon:
r=4cm
The area Ac of the circle is given by the formula:
Ac=πr2
Substituting π=3.14 and r=4:
Ac=3.14×(42)=3.14×16
Ac=3.14×16=50.24cm2
The area of the circle is 50.24cm2.
Topper's Solved these Questions
Similar Questions
Knowledge Check
A triangle and a regular hexagon have the same perimeter. If the area of the hexagon is 72√3, what is the area of the triangle ?
A62.354B83.138C101.823D103.923
The area of a circle is 616 cm2. Find the radius of the circle.
View SolutionIn figure, find the area of the shaded region [Useπ=3.14]
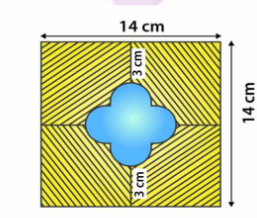
View SolutionIn figure, find the area of the shaded region [Useπ=3.14]
View SolutionIf the area of regular hexagon is 96√3, what is its perimeter?
View SolutionFind the area of the shaded region in Figure, if AC=24 cm , BC=10 cm and O is the centre of the circle. (Use π=3.14)
View SolutionA square is inscribed in a circle of radius 7 cm. Find the area of the square.
View SolutionABCDEF is a regular hexagon with centre O ). If the area of triangle OAB is 9 cm2 , find the area of: (i) the hexagon and (ii) the circle in which the hexagon is inscribed.
View Solution
