A circle is inscribed in an equilateral triangle ABC of side 12 cm, touching its sides (Figure). Find the radius of the inscribed circle and the area of the shaded part.
To solve the problem of finding the radius of the inscribed circle in an equilateral triangle and the area of the shaded part, we can follow these steps:
Step 1: Calculate the Area of the Equilateral Triangle
The formula for the area A of an equilateral triangle with side length a is given by:
A=√34a2
Given that the side length a=12 cm, we can substitute this value into the formula:
A=√34×122=√34×144=36√3 cm2
Step 2: Calculate the Radius of the Inscribed Circle
The radius R of the inscribed circle in an equilateral triangle can be calculated using the formula:
R=As
where A is the area of the triangle and s is the semi-perimeter of the triangle. The semi-perimeter s is given by:
s=3a2=3×122=18 cm
Now substituting the area we calculated in Step 1:
R=36√318=2√3 cm
Step 3: Calculate the Area of the Circle
The area Ac of the inscribed circle can be calculated using the formula:
Ac=πR2
Substituting the radius we found:
Ac=π(2√3)2=π×4×3=12π cm2
Step 4: Calculate the Area of the Shaded Region
The area of the shaded region is the area of the triangle minus the area of the circle:
Area of shaded region=A−Ac
Substituting the values we calculated:
Area of shaded region=36√3−12π
Step 5: Numerical Approximation
Using approximate values for √3≈1.732 and π≈3.14:
36√3≈36×1.732≈62.352 cm2
12π≈12×3.14≈37.68 cm2
Now, calculating the area of the shaded region:
Area of shaded region≈62.352−37.68≈24.672 cm2
Final Answers
- Radius of the inscribed circle: R=2√3 cm
- Area of the shaded region: ≈24.672 cm²
The formula for the area A of an equilateral triangle with side length a is given by:
The radius R of the inscribed circle in an equilateral triangle can be calculated using the formula:
The area Ac of the inscribed circle can be calculated using the formula:
The area of the shaded region is the area of the triangle minus the area of the circle:
Using approximate values for √3≈1.732 and π≈3.14:
12π≈12×3.14≈37.68 cm2
- Radius of the inscribed circle: R=2√3 cm
- Area of the shaded region: ≈24.672 cm²
Topper's Solved these Questions
Similar Questions
Construct a circle, inscribing an equilateral triangle with side 5.6 cm.
View SolutionA circle is inscribed in an equilateral triangle of side a. Find the area of any square inscribed in this circle.
View SolutionAn equilateral triangle of side 9 cm is inscribed in a circle. Find the radius of the circle.
View SolutionA circle is inscribed in an equilateral triangle of side a. The area of any square inscribed in this circle is ______.
View SolutionA circle is cirumscribed in an equilateral triangle of side 'l'. The area of any square inscribed in the circle is :
View SolutionIn Fig. an equilateral triangle ABC of side 6 cm has been inscribed in a circle. Find the area of the shaded region. (Take π=3.14 )
View SolutionIn figure, a square of diagonal 8 cm is inscribed in a circle. Find the area of the shaded region.
View SolutionABC is an equilateral triangle inscribed in a circle of radius 4 cm. Find the area of the shaded portion.

View SolutionIn an equilateral triangle of side 24cm, a circle is inscribed touching its sides. Find the area of the remaining portion of the triangle (Take√3=1.732)
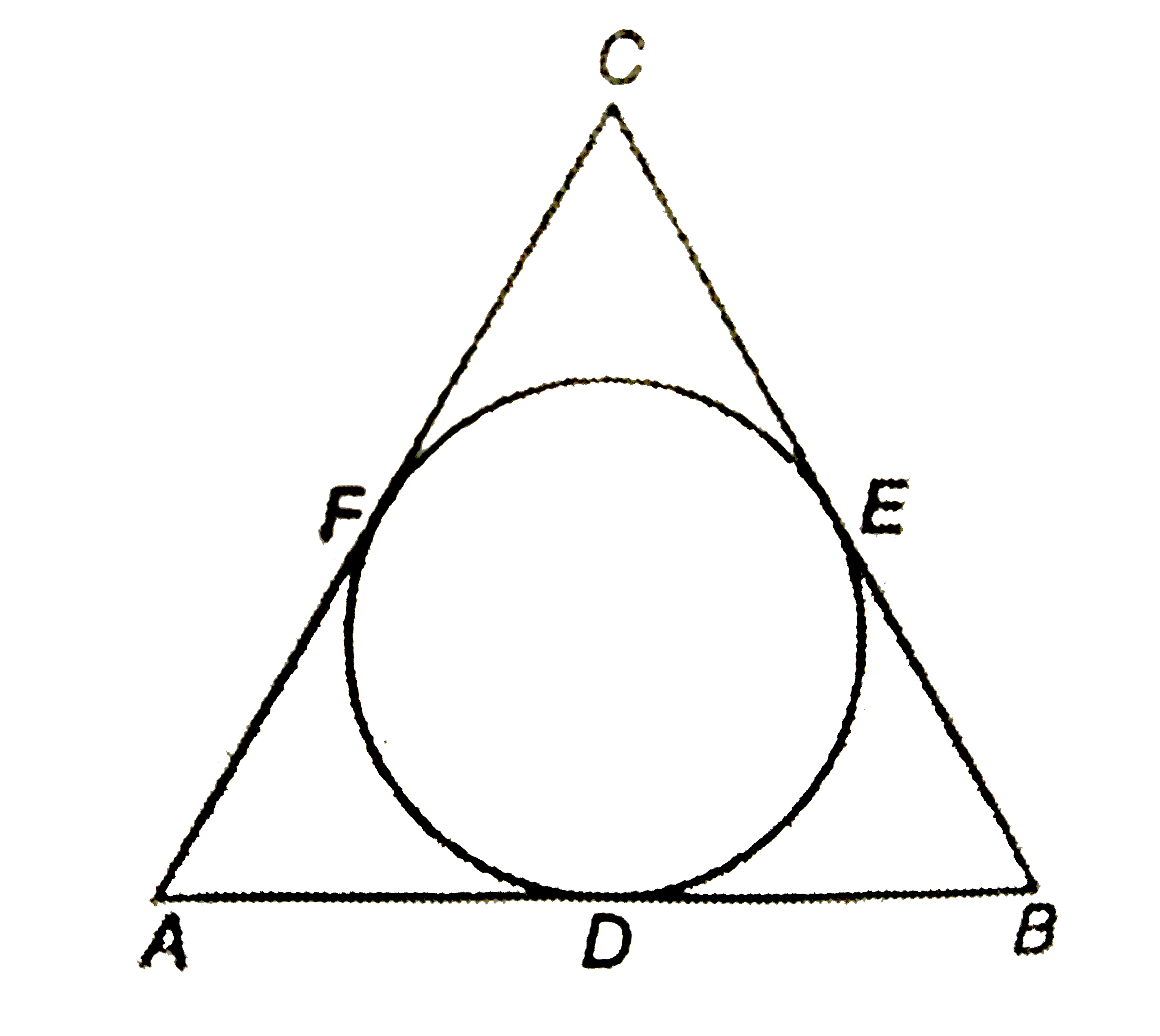
View SolutionA circle is inscribed in a △ABC having sides 8 cm, 10 cm and 12 cm as shown in figure. Find AD, BE and CF.
View Solution
