Question

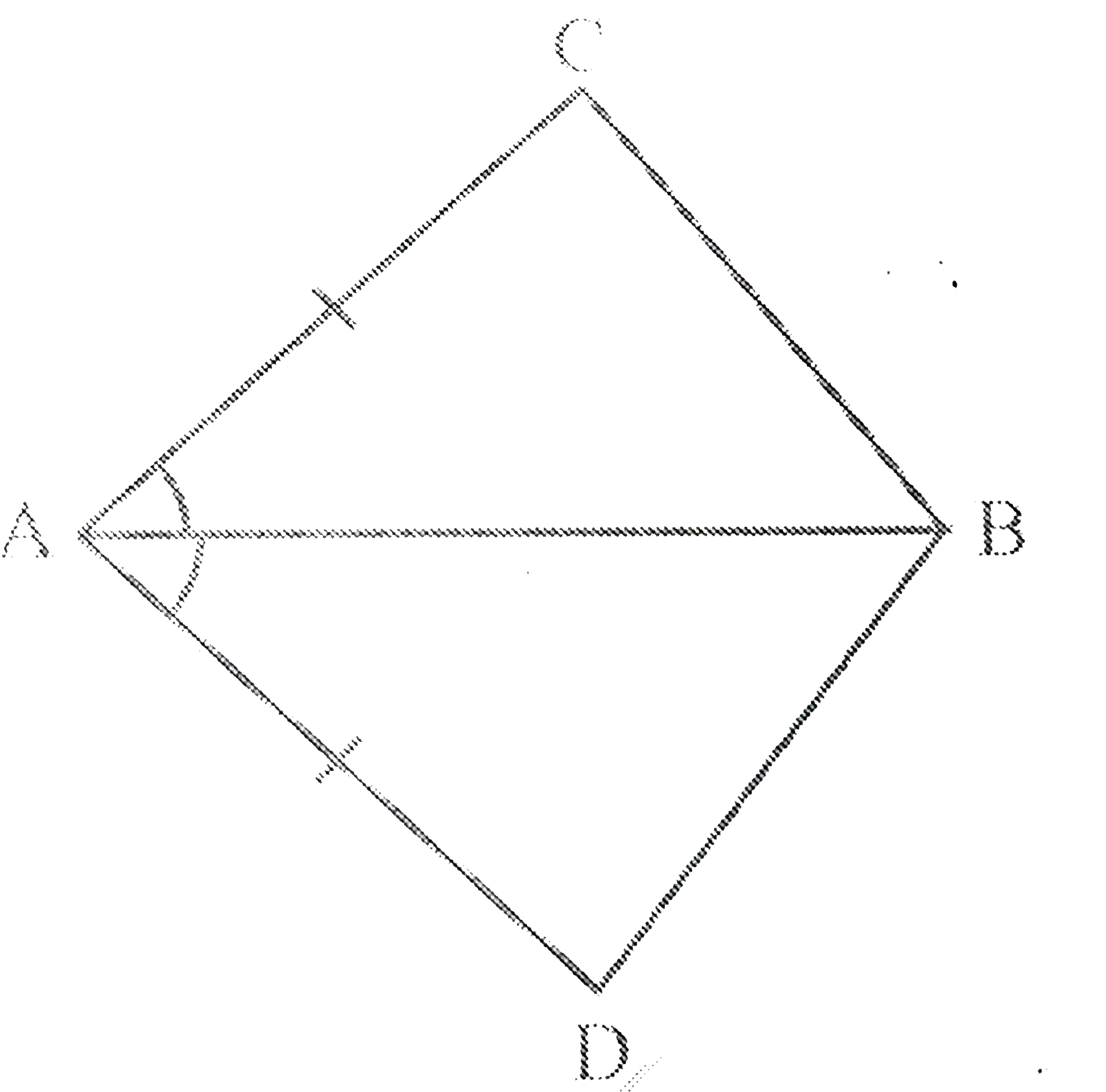
In quadrilateral $ACBD,AC=ADandAB$
bisects $\angle A$
. Show that $ABC\cong ABD\stackrel{.}{}$
What can you say about $BCandBD?$

Explanation

To solve the problem, we need to show that triangle ABC is congruent to triangle ABD using the information given about quadrilateral ACBD. Here’s a step-by-step solution:

Step 1: Identify Given Information
We are given:
- AC = AD (sides of the quadrilateral)
- AB bisects angle A (which means angle CAB = angle BAD)

Step 2: Draw the Diagram
Draw quadrilateral ACBD with points A, B, C, and D. Mark the sides AC and AD as equal. Also, mark the angle bisector AB such that it divides angle A into two equal angles.

Step 3: Identify Angles and Sides
From the information given:
- Angle CAB = Angle BAD (since AB bisects angle A)
- AC = AD (given)
- AB = AB (common side in both triangles)

Step 4: Apply the SAS Congruence Criterion
Now we have:
- One side (AC) is equal to another side (AD).
- The angle between these two sides (CAB) is equal to the angle (BAD).
- The common side (AB) is equal to itself.

By the Side-Angle-Side (SAS) congruence criterion, we can conclude that:
\( \triangle ABC \cong \triangle ABD \)

Step 5: Conclusion about BC and BD
Since the triangles ABC and ABD are congruent, by the Corresponding Parts of Congruent Triangles (CPCT) theorem, we can say:
\( BC = BD \)

Final Answer
Thus, we have shown that triangle ABC is congruent to triangle ABD, and we conclude that \( BC = BD \).

---