Out of 100 students two sections of 40 and 60 students are formed. If you and your friends are among the 100 students, what is the probability that i. You both enter the same section? ii. You both enter the different section?
To solve the problem, we need to calculate the probabilities for the two scenarios:
1. You and your friend both enter the same section.
2. You and your friend enter different sections.
Let's break down the solution step by step.
Step 1: Understand the total number of students and sections
We have a total of 100 students divided into two sections:
- Section A: 40 students
- Section B: 60 students
Step 2: Calculate the probability that both enter the same section
To find the probability that both you and your friend enter the same section, we need to consider the two scenarios:
- Both enter Section A (40 students)
- Both enter Section B (60 students)
Step 2.1: Calculate combinations for both sections
- The number of ways to choose 2 students from Section A (40 students):
C(40,2)=40×392=780
- The number of ways to choose 2 students from Section B (60 students):
C(60,2)=60×592=1770
Step 2.2: Calculate total combinations from all students
The total number of ways to choose 2 students from the total of 100 students:
C(100,2)=100×992=4950
Step 2.3: Calculate the probability
Now, we can find the probability that both you and your friend enter the same section:
P(Same Section)=C(40,2)+C(60,2)C(100,2)=780+17704950=25504950
Simplifying this fraction:
P(Same Section)=2550÷304950÷30=85165=1733
Step 3: Calculate the probability that both enter different sections
To find the probability that you and your friend enter different sections, we can use the complementary probability:
P(Different Sections)=1−P(Same Section)
Substituting the value we found:
P(Different Sections)=1−1733=33−1733=1633
Final Answers
1. The probability that you both enter the same section is 1733.
2. The probability that you both enter different sections is 1633.
2. You and your friend enter different sections.
We have a total of 100 students divided into two sections:
- Section A: 40 students
- Section B: 60 students
To find the probability that both you and your friend enter the same section, we need to consider the two scenarios:
- Both enter Section A (40 students)
- Both enter Section B (60 students)
- The number of ways to choose 2 students from Section A (40 students):
C(40,2)=40×392=780
C(60,2)=60×592=1770
The total number of ways to choose 2 students from the total of 100 students:
C(100,2)=100×992=4950
Now, we can find the probability that both you and your friend enter the same section:
P(Same Section)=C(40,2)+C(60,2)C(100,2)=780+17704950=25504950
Simplifying this fraction:
P(Same Section)=2550÷304950÷30=85165=1733
To find the probability that you and your friend enter different sections, we can use the complementary probability:
P(Different Sections)=1−P(Same Section)
Substituting the value we found:
P(Different Sections)=1−1733=33−1733=1633
1. The probability that you both enter the same section is 1733.
2. The probability that you both enter different sections is 1633.
Topper's Solved these Questions
Similar Questions
Out of 100 students, two sections of 40 and 60 are formed. If you and your friend are among the 100 students, what is the probability that(a) you both enter the same section?(b) you both enter the different sections?
View SolutionTwo friend were born in the same year, what is the probability that they have :
(i) same birthday (ii) different birthday.
View SolutionLet there are 4 sections of 25 students each in a coaching class. Now, out of 150 students 100 are to be selected randomly and enrolled in these sections. Then, the probability that the students A and B (both present in 150 students) are selected and placed in the same section, is
View SolutionA box contains 10 blocks, 3 of which are red. If you pick two blocks out of the box, what is the probability that they are both red? Assume that you do NOT replace the first block after you have picked it.
View Solution100 students appeared for two examinations .60 passed the first , 50 passed the second and 30 passed both . Find the probability that a student selected at random has failed in both examinations .
View SolutionSavita and Hamida are friends. What is the probability that both will have (i) the same birthday? (ii) different birthdays? (ignoring a leap year).
View SolutionIn a class of 60 students, 40 opted for NCC, 30 opted for NSS and 20 opted for both NCC and NSS. If one of these students is selected at random, then the probability that the student selected has opted neither for NCC nor for NSS is
View SolutionTwo dice are thrown at the same time. Find the probability of getting
(i) Same number on both dice.
(ii) different number of both dice.
View SolutionThere are 100 students in class VII in a school. 40 students are in Section A, 33 in Section B, and the remaining students are in Section C. Find what percentage of students are there in each section.
View SolutionThe following table gives the distribution of students of two sections according to the marks obtained by them
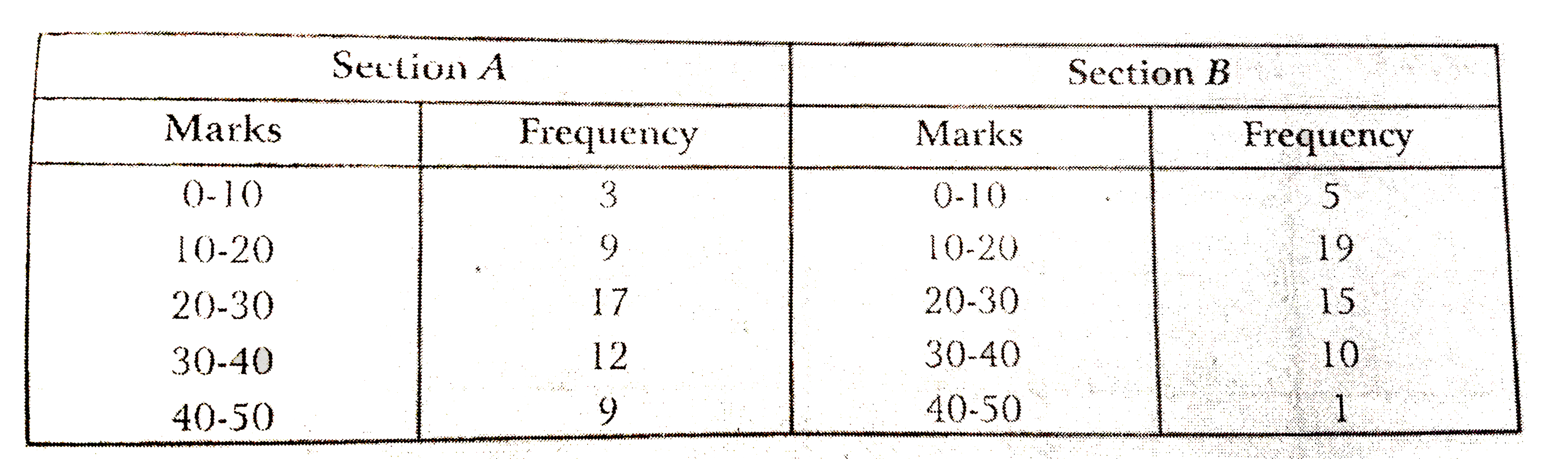
Represent the marks of the students of both the sections on the same graph by two frquency polygons. From the two polygons compare the performance of the two sections.
View Solution
