Let X be the discrete random variable. Then the variance of X is (a). E(X2) (b). E(X2)+(E(X))2 (c). E(X2)−(E(X))2 (d). √E(X2)−(E(X))2
To solve the question regarding the variance of a discrete random variable X, we need to recall the formula for variance. The variance of a discrete random variable X is defined as:
Var(X)=E(X2)−(E(X))2
Now, let's go through the options provided in the question step by step:
1. Understanding the Variance Formula:
- The variance measures how much the values of a random variable differ from the mean. It is calculated using the expected value of the square of the variable and the square of the expected value of the variable.
2. Identifying the Correct Formula:
- The formula for variance is given by:
Var(X)=E(X2)−(E(X))2
- This means that we take the expected value of the square of X and subtract the square of the expected value of X.
3. Analyzing the Options:
- (a) E(X2): This is not the variance; it is just the expected value of X2.
- (b) E(X2)+(E(X))2: This is incorrect because it adds the square of the expected value instead of subtracting it.
- (c) E(X2)−(E(X))2: This matches the variance formula we derived.
- (d) √E(X2)−(E(X))2: This represents the standard deviation, not the variance.
4. Conclusion:
- The correct answer is option (c): E(X2)−(E(X))2.
Thus, the final answer is:
The correct option is (c): E(X2)−(E(X))2
- The variance measures how much the values of a random variable differ from the mean. It is calculated using the expected value of the square of the variable and the square of the expected value of the variable.
- The formula for variance is given by:
Var(X)=E(X2)−(E(X))2
- This means that we take the expected value of the square of X and subtract the square of the expected value of X.
- (a) E(X2): This is not the variance; it is just the expected value of X2.
- (b) E(X2)+(E(X))2: This is incorrect because it adds the square of the expected value instead of subtracting it.
- (c) E(X2)−(E(X))2: This matches the variance formula we derived.
- (d) √E(X2)−(E(X))2: This represents the standard deviation, not the variance.
- The correct answer is option (c): E(X2)−(E(X))2.
Topper's Solved these Questions
Similar Questions
Knowledge Check
For a random variable X, E(X) = 3 and E(X2)=11 .Then variance of X is
A8B5C2D1
Let f(x) be a polynomial. Then, the second order derivative of f(ex) is f(ex)e2x+f'(ex)ex (b) f(ex)ex+f'(ex) (c) f(e^x)e^(2x)+f(ex)ex (d) f(ex)
View SolutionThe value of the integral ∫a−axex21+x2dx is (A) ea2 (B) 0 (C) e−a2 (D) a
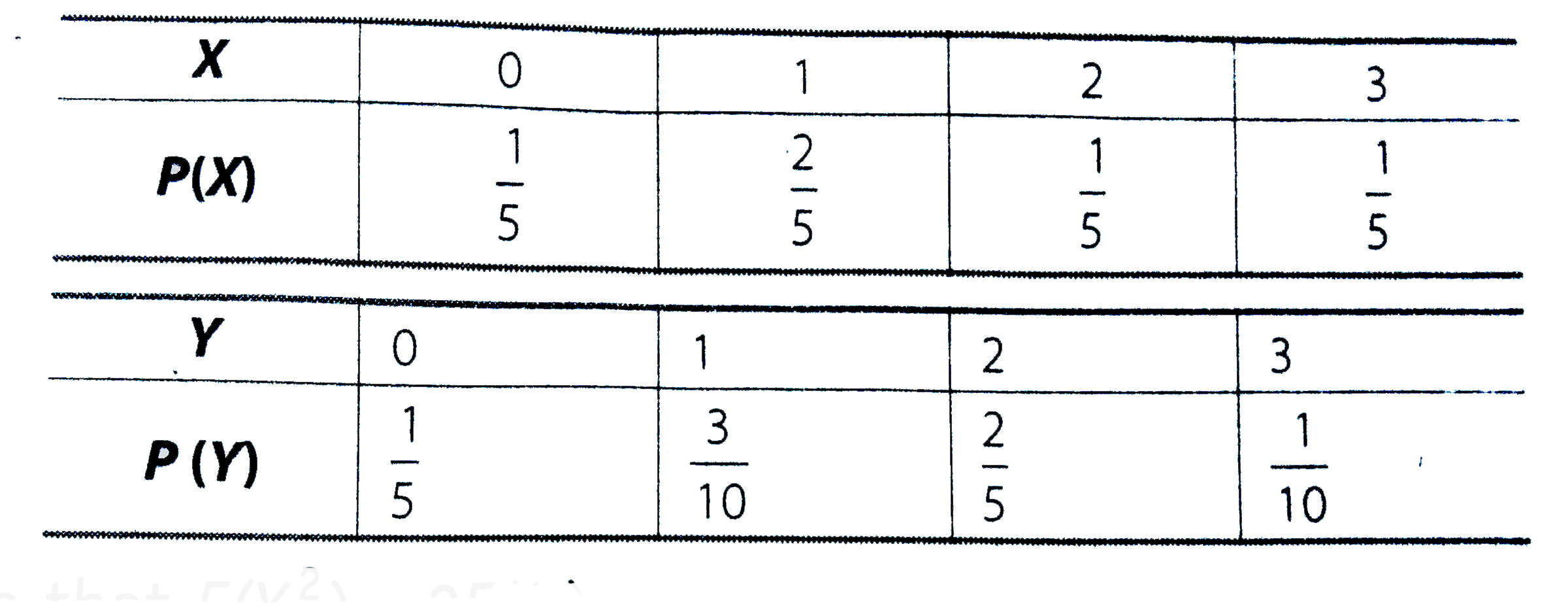
View SolutionThe probability distribution of a discrete random variable x is given as under
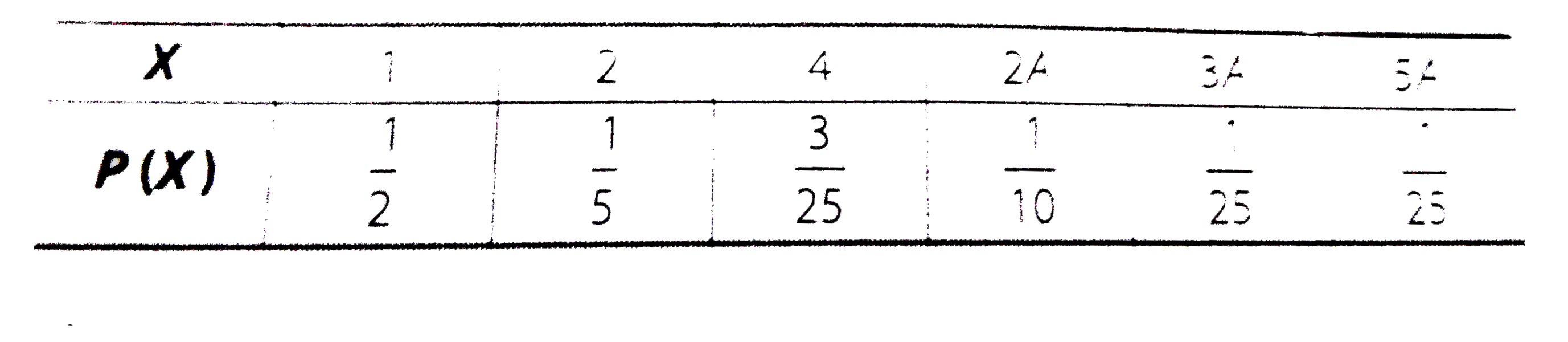
Calculate
(i) the value of A, if E(X)=2.94.
(ii) variance of X.
View Solution∫(1+(log)ex)21+(log)exx+1+((log)ex√x)2dx=
View SolutionLet f,g and h be real-valued functions defined on the interval [0,1] by f(x)=ex2+e−x2 , g(x)=xex2+e−x2 and h(x)=x2ex2+e−x2. if a,b and c denote respectively, the absolute maximum of f,g and h on [0,1] then
View SolutionIntegrate the functionse2x−e−2xe2x+e−2x
View SolutionIntegrate the functionse2x−e−2xe2x+e−2x
View Solution