A book exhibition was held for four days in a school. The number of tickets sold at the counter on the first, second, third and final days were respectively 1094, 1812, 2050 and 2751. Find the total number of tickets sold on all the four days.
To find the total number of tickets sold over the four days of the book exhibition, we will add the number of tickets sold each day. Here’s the step-by-step solution:
Step 1: Write down the number of tickets sold each day.
- Day 1: 1094 tickets
- Day 2: 1812 tickets
- Day 3: 2050 tickets
- Day 4: 2751 tickets
Step 2: Set up the addition of the tickets sold.
We need to add the numbers:
1094 + 1812 + 2050 + 2751
Step 3: Add the numbers step by step.
1. First, add the tickets sold on Day 1 and Day 2:
- 1094 + 1812 = 2906
2. Next, add the result to the tickets sold on Day 3:
- 2906 + 2050 = 4956
3. Finally, add the result to the tickets sold on Day 4:
- 4956 + 2751 = 7707
Step 4: Write the final total.
The total number of tickets sold over the four days is 7707.
---
- Day 1: 1094 tickets
- Day 2: 1812 tickets
- Day 3: 2050 tickets
- Day 4: 2751 tickets
We need to add the numbers:
1094 + 1812 + 2050 + 2751
1. First, add the tickets sold on Day 1 and Day 2:
- 1094 + 1812 = 2906
- 2906 + 2050 = 4956
- 4956 + 2751 = 7707
The total number of tickets sold over the four days is 7707.
Topper's Solved these Questions
Similar Questions
A book exhibition was held for four days in a school. The number of tickets sold at the counter on the first, second, third and final days were respectively 1094, 1812, 2050 and 2751. Find the total number of tickets sold on all the four days.
View SolutionOn a rainy day, 94% of the students were present in a school. If the number of students absent on that day was 174, find the total strength of the school.
View Solution
Knowledge Check
The mean number of tickets sold daily by a theater over a seven - day period was 52. The theater sold 46 tickets on the last day of that period. What was the mean number of tickets sold daily over the first six days ?
A53B54C55D56Tickets to the annual Follies Show at Littleton High School are $4 for adults and $2.50 for students. There is a cost of $750 to produce the show. If the x-axis represents the number of adult tickets sold and the y-axis represents the number of student tickets sold, which graph represents all the possible combinations of ticket sales that allow the junior class to at least cover the cost of the show ?
A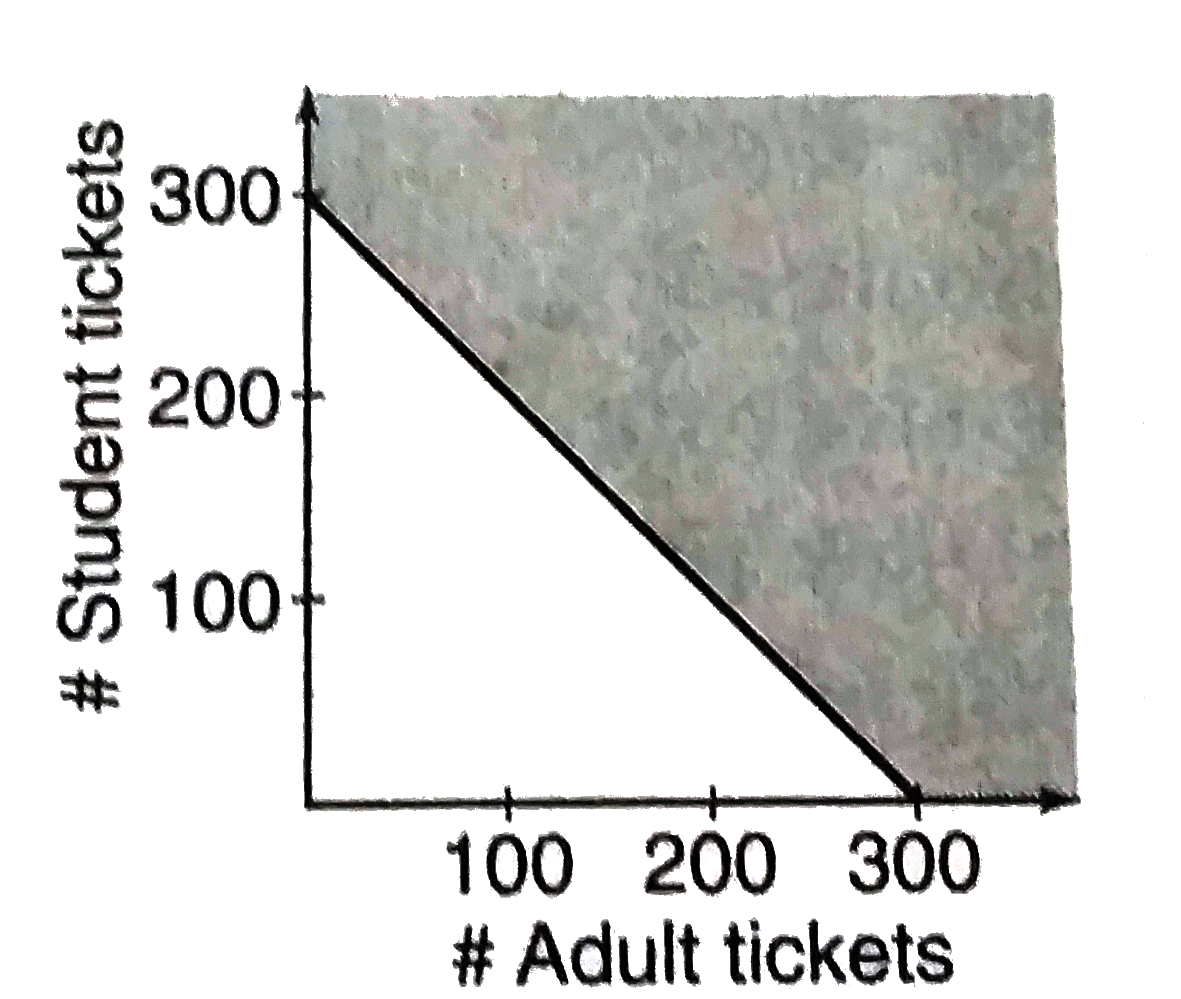 B
B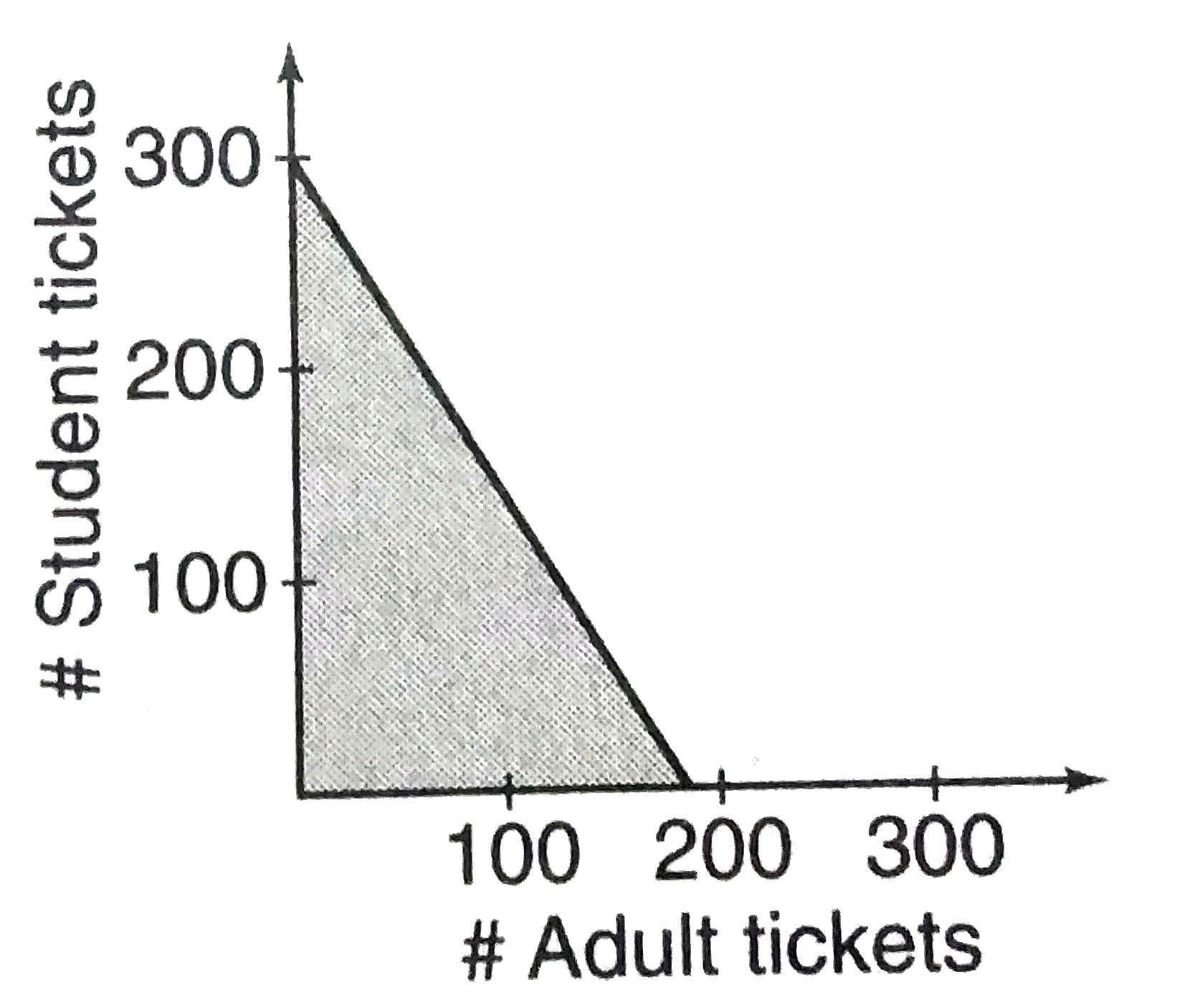 C
C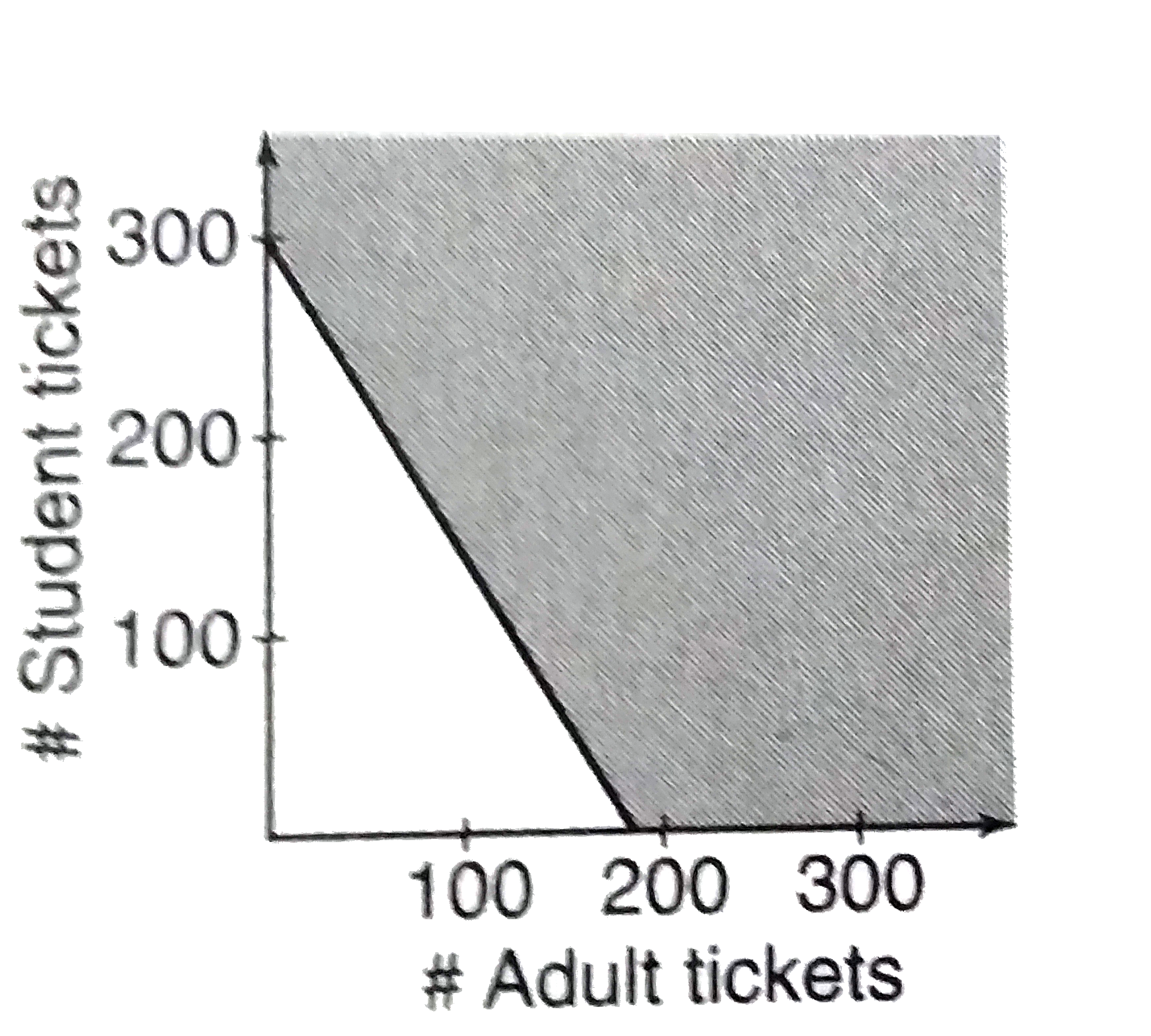 D
D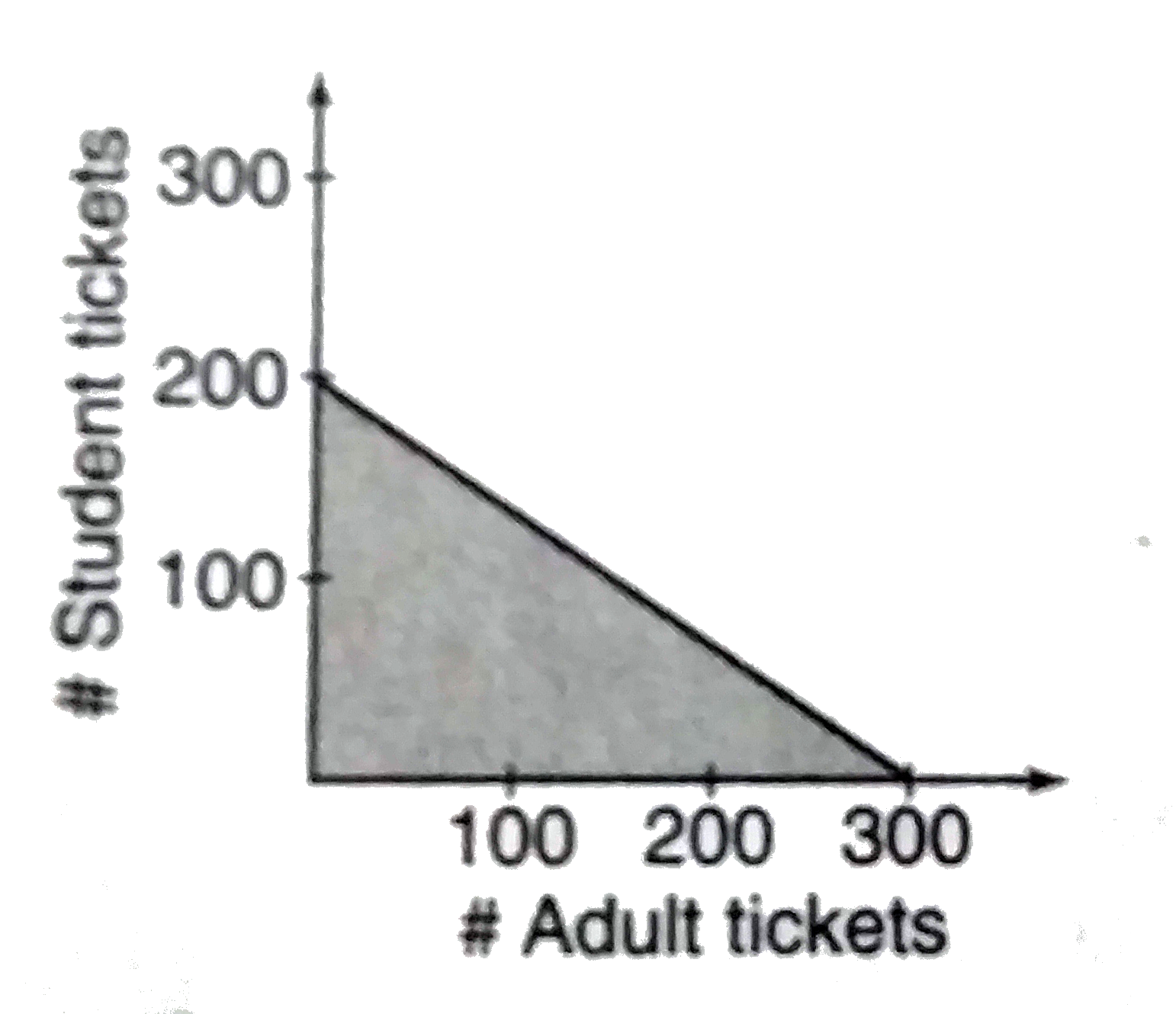
Two types of tickets were sold for a concert held at an amphitheater. Tickets to sit on a bench during the concert cost $75 each, and tickets to sit on the lawn during the concert cost $40 each. Organizers of the concert announced that 350 tickets had been sold and that $19,250 had been raised through ticket sales alone. Which of the following systems of equations could be used to find the number of tickets for bench seats, B, and the number of tickets for lawn seats, L, that were sold for the concert?
A(75B)(40L)=1,950
B+L=350B40B + 75L = 19,250
B+L=350C75B + 40L =350
B+L=19,250D75B+40L=19,250
B+L=350
150 workers were engaged to finish a piece of work in a certain number of days. Four workers dropped the second day, four more workers dropped the third day and so on. It takes 8 more days to finish the work now. Find the number of days in which the work was completed.
View SolutionThere are two confectioneries in a locality. The number of chocolate boxes in the first shop is 250 less than twice the number of chocolate boxes available in the second shop. By the end of the day, the first shop has sold 350 boxes and the second shop has sold 200 boxes. The number of boxes left unsold in both the shops is equal. Find the number of chocolates in both the shops at the beginning of the day.
View SolutionA dealer in refrigerators estimates from his past experience the probabilities of his selling refrigerators in a day. These are as follows: No of refrigerators sold in a day: 0 1 2 3 4 5 6 Probability 0.03 0.20 0.23 0.25 0.12 0.10 0.07 Find the mean number of refrigerators sold in a day.
View SolutionAbout 150 workers were engaged to finish a piece of work in a certain number of days. Four workers stopped working on the second day, four more workers stopped their work on the third day and so on. It took 8 more days to finish the work. Then the number of days in which the work was completed is 29 days b. 24 days c. 25 days d. none of these
View SolutionThe number of Mathematics books sold by a shopkeeper on six consecutive days is shown below Draw a bar graph to represent the above information choosing the scale of your choice.
View Solution
