Match the equivalent fractions and write another 2 for each: 250400 180200 660990 180360 220550
To solve the problem of matching equivalent fractions and writing two additional equivalent fractions for each given fraction, we will follow these steps:
Step 1: Identify the first fraction 250400
1. Simplify the fraction by finding the greatest common divisor (GCD) of 250 and 400.
- GCD of 250 and 400 is 50.
- Divide both the numerator and denominator by 50:
\(
\frac{250 \div 50}{400 \div 50} = \frac{5}{8}
\)
2. Now, we can write two more equivalent fractions:
- Multiply both the numerator and denominator by 2:
\(
\frac{5 \times 2}{8 \times 2} = \frac{10}{16}
\)
- Multiply both the numerator and denominator by 3:
\(
\frac{5 \times 3}{8 \times 3} = \frac{15}{24}
\)
Step 2: Identify the second fraction 180200
1. Simplify the fraction by finding the GCD of 180 and 200.
- GCD of 180 and 200 is 20.
- Divide both the numerator and denominator by 20:
\(
\frac{180 \div 20}{200 \div 20} = \frac{9}{10}
\)
2. Now, we can write two more equivalent fractions:
- Multiply both the numerator and denominator by 2:
\(
\frac{9 \times 2}{10 \times 2} = \frac{18}{20}
\)
- Multiply both the numerator and denominator by 3:
\(
\frac{9 \times 3}{10 \times 3} = \frac{27}{30}
\)
Step 3: Identify the third fraction 660990
1. Simplify the fraction by finding the GCD of 660 and 990.
- GCD of 660 and 990 is 330.
- Divide both the numerator and denominator by 330:
\(
\frac{660 \div 330}{990 \div 330} = \frac{2}{3}
\)
2. Now, we can write two more equivalent fractions:
- Multiply both the numerator and denominator by 2:
\(
\frac{2 \times 2}{3 \times 2} = \frac{4}{6}
\)
- Multiply both the numerator and denominator by 3:
\(
\frac{2 \times 3}{3 \times 3} = \frac{6}{9}
\)
Step 4: Identify the fourth fraction 180360
1. Simplify the fraction by finding the GCD of 180 and 360.
- GCD of 180 and 360 is 180.
- Divide both the numerator and denominator by 180:
\(
\frac{180 \div 180}{360 \div 180} = \frac{1}{2}
\)
2. Now, we can write two more equivalent fractions:
- Multiply both the numerator and denominator by 2:
\(
\frac{1 \times 2}{2 \times 2} = \frac{2}{4}
\)
- Multiply both the numerator and denominator by 3:
\(
\frac{1 \times 3}{2 \times 3} = \frac{3}{6}
\)
Step 5: Identify the fifth fraction 220550
1. Simplify the fraction by finding the GCD of 220 and 550.
- GCD of 220 and 550 is 110.
- Divide both the numerator and denominator by 110:
\(
\frac{220 \div 110}{550 \div 110} = \frac{2}{5}
\)
2. Now, we can write two more equivalent fractions:
- Multiply both the numerator and denominator by 2:
\(
\frac{2 \times 2}{5 \times 2} = \frac{4}{10}
\)
- Multiply both the numerator and denominator by 3:
\(
\frac{2 \times 3}{5 \times 3} = \frac{6}{15}
\)
Summary of Equivalent Fractions:
- 250400 matches with 58; additional: 1016, 1524
- 180200 matches with 910; additional: 1820, 2730
- 660990 matches with 23; additional: 46, 69
- 180360 matches with 12; additional: 24, 36
- 220550 matches with 25; additional: 410, 615
1. Simplify the fraction by finding the greatest common divisor (GCD) of 250 and 400.
- GCD of 250 and 400 is 50.
- Divide both the numerator and denominator by 50:
\(
\frac{250 \div 50}{400 \div 50} = \frac{5}{8}
\)
2. Now, we can write two more equivalent fractions:
- Multiply both the numerator and denominator by 2:
\(
\frac{5 \times 2}{8 \times 2} = \frac{10}{16}
\)
- Multiply both the numerator and denominator by 3:
\(
\frac{5 \times 3}{8 \times 3} = \frac{15}{24}
\)
1. Simplify the fraction by finding the GCD of 180 and 200.
- GCD of 180 and 200 is 20.
- Divide both the numerator and denominator by 20:
\(
\frac{180 \div 20}{200 \div 20} = \frac{9}{10}
\)
2. Now, we can write two more equivalent fractions:
- Multiply both the numerator and denominator by 2:
\(
\frac{9 \times 2}{10 \times 2} = \frac{18}{20}
\)
- Multiply both the numerator and denominator by 3:
\(
\frac{9 \times 3}{10 \times 3} = \frac{27}{30}
\)
1. Simplify the fraction by finding the GCD of 660 and 990.
- GCD of 660 and 990 is 330.
- Divide both the numerator and denominator by 330:
\(
\frac{660 \div 330}{990 \div 330} = \frac{2}{3}
\)
2. Now, we can write two more equivalent fractions:
- Multiply both the numerator and denominator by 2:
\(
\frac{2 \times 2}{3 \times 2} = \frac{4}{6}
\)
- Multiply both the numerator and denominator by 3:
\(
\frac{2 \times 3}{3 \times 3} = \frac{6}{9}
\)
1. Simplify the fraction by finding the GCD of 180 and 360.
- GCD of 180 and 360 is 180.
- Divide both the numerator and denominator by 180:
\(
\frac{180 \div 180}{360 \div 180} = \frac{1}{2}
\)
2. Now, we can write two more equivalent fractions:
- Multiply both the numerator and denominator by 2:
\(
\frac{1 \times 2}{2 \times 2} = \frac{2}{4}
\)
- Multiply both the numerator and denominator by 3:
\(
\frac{1 \times 3}{2 \times 3} = \frac{3}{6}
\)
1. Simplify the fraction by finding the GCD of 220 and 550.
- GCD of 220 and 550 is 110.
- Divide both the numerator and denominator by 110:
\(
\frac{220 \div 110}{550 \div 110} = \frac{2}{5}
\)
2. Now, we can write two more equivalent fractions:
- Multiply both the numerator and denominator by 2:
\(
\frac{2 \times 2}{5 \times 2} = \frac{4}{10}
\)
- Multiply both the numerator and denominator by 3:
\(
\frac{2 \times 3}{5 \times 3} = \frac{6}{15}
\)
- 250400 matches with 58; additional: 1016, 1524
- 180200 matches with 910; additional: 1820, 2730
- 660990 matches with 23; additional: 46, 69
- 180360 matches with 12; additional: 24, 36
- 220550 matches with 25; additional: 410, 615
Topper's Solved these Questions
Similar Questions
Knowledge Check
A national math examination has 4 statics problems. The distribution of the number of students who ansered the question correctly is shown in the chart. If 400 students took the exam and each question was worth 25 points, then what is the average score of the students taking the exam?
Question NumberNumber of students who solved the question1200230433504250A1 pointB25 pointsC26 pointsD69 points
If the cost of 15 mangoes is Rs 180, then what is the cost of 25 mangoes?
(a) 220
(b) 360
(c) 200
(d) 300
View SolutionWrite three equivalent fractions each for 23and(−5)6 such that one of the equivalent rational numbers for each has denominator 36.
View SolutionThe difference between the interest obtained for Rs 1000 at 12% per annum for 3 years and that for Rs 1500 at 8% per annum for 112 years is (a) Rs 360 (b) Rs 300 (c) Rs 180 (d) Rs 200
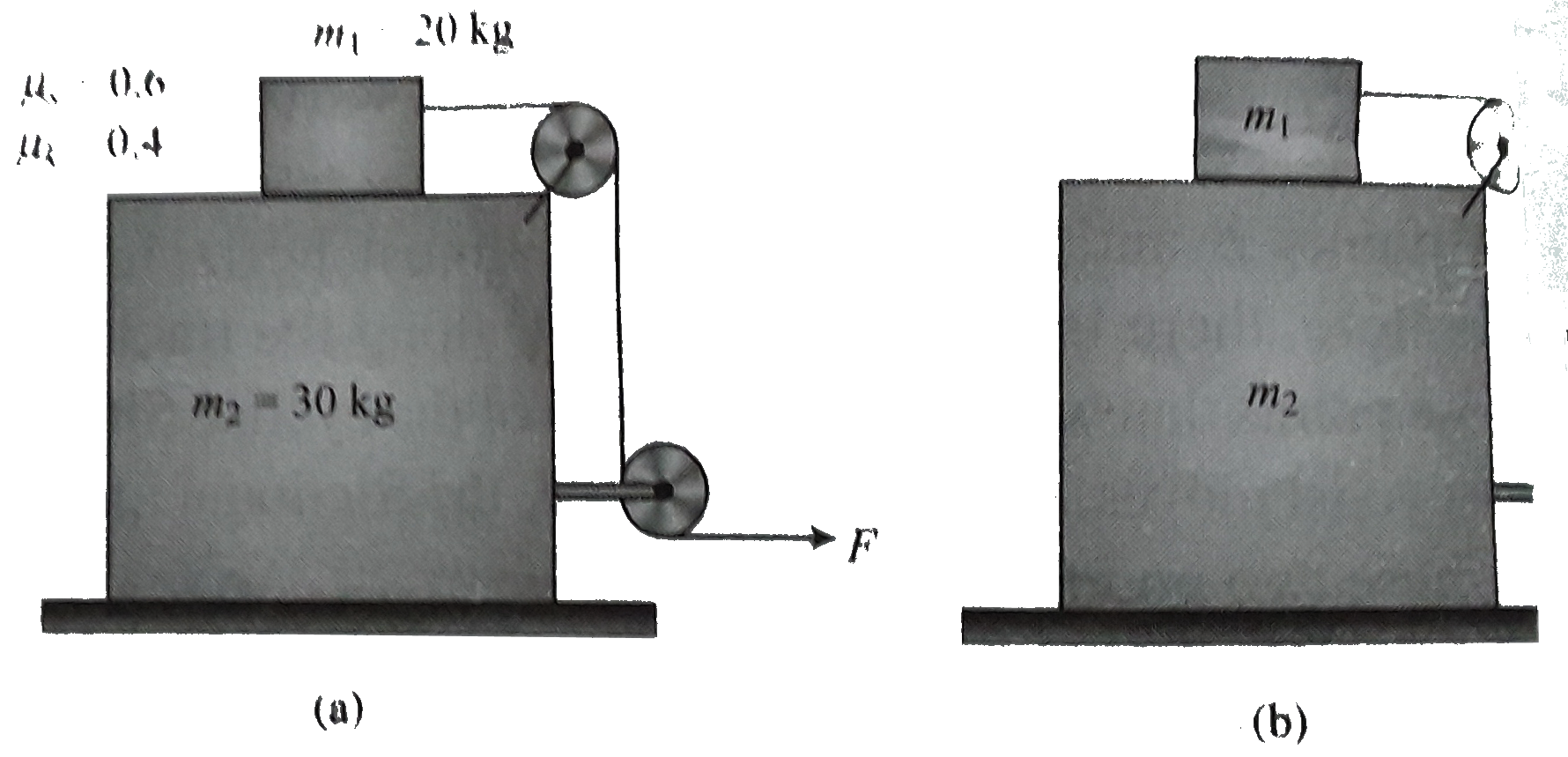
View SolutionA block of mass m1=20kg as placed on a wedge of mass m2=30kg rest on a smooth flooras shpwn in Fig (a) . Friction exists between block and wedge.
a. Find the acceleration of each block if (i) F=180N and (ii) F=400N
b. Solve the problem if force F=180N is direction upwards as shown in Fig (b)
View SolutionTwo parallel plates A and B are joined together to form a compound plate . The thicknesses of the plate are 4.0cm and 2.5cm respectively and the area of cross section is 100cm2 for each plate. The thermal conductivities are KA=200Wm−1C−1 for plate A and KB=400Wm−1C−1 for the plate B. The outer surface of the plate A is maintained at100∘C and the outer surface of the plate B is maintained at 0∘C. Find (a) the rate of heat flow through any cross section, (b) the temperature at the interface and (c) the equivalent thermal conductivity of the compound plate.

View SolutionKamla has a triangular field with sides 240 m, 200 m, 360 m, where she grew wheat. In another triangular field with sides 240 m, 320 m, 400 m adjacent to the previous field, she wanted to grow potatoes and onions. She divided the field in two parts by joining the mid point of the longest side to the opposite vertex and grew potatoes in one part and onions in one part. How much area (in hectares) has been used for wheat,potatoes and onions? ( 1 hectare = 10000 m2 )
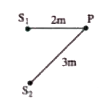
View SolutionTwo identical speakers emit sound waves of frequency 660Hz uniformly in all directions. The audio output of each speaker is 1mW and the speed of sound in air. A point P is a distance 2m from one speaker and 3m from the other. If they are driven coherently but out of phase by 180∘ , the intensity at the point 'P' is
View Solution
