In Figure, If the face EFGH is taken as the base, then name the lateral faces. Also, name the line segment representing the height of the cuboid.
To solve the question, we need to identify the lateral faces of the cuboid when the face EFHG is taken as the base, and also determine the line segments that represent the height of the cuboid.
1. Identify the Base Face:
- The base face given is EFHG.
2. Understand Lateral Faces:
- Lateral faces are the faces that are adjacent to the base face.
3. List the Lateral Faces:
- The lateral faces adjacent to the base EFHG are:
- Face AEHD
- Face AEFB
- Face BFGC
- Face DGHF
4. Identify the Height of the Cuboid:
- The height of the cuboid can be represented by the vertical line segments connecting the base to the top face.
- In this case, the line segments that represent the height of the cuboid are:
- Line segment GC
- Line segment BF
- Line segment AE
- Line segment DH
Final Answer:
- Lateral Faces: AEHD, AEFB, BFGC, DGHF
- Line Segments Representing Height: GC, BF, AE, DH
- The base face given is EFHG.
- Lateral faces are the faces that are adjacent to the base face.
- The lateral faces adjacent to the base EFHG are:
- Face AEHD
- Face AEFB
- Face BFGC
- Face DGHF
- The height of the cuboid can be represented by the vertical line segments connecting the base to the top face.
- In this case, the line segments that represent the height of the cuboid are:
- Line segment GC
- Line segment BF
- Line segment AE
- Line segment DH
- Lateral Faces: AEHD, AEFB, BFGC, DGHF
- Line Segments Representing Height: GC, BF, AE, DH
Topper's Solved these Questions
Similar Questions
In a parallelogram OABC, vectors →a,→b,→c are respectively the positions of vectors of vertices A,B,C with reference to O as origin. A point E is taken on the side BC which divide the line 2:1 internally. Also the line segment AE intersect the line bisecting the angle O internally in point P. If CP, when extended meets AB in point F. Then The position vector of point P, is
View SolutionIs it true that a cuboid is also known as a rectangular prism? What is the shape of the lateral faces of this prism?
View Solution
Knowledge Check
V=13π(d2)2h
A circle of rubber with a constant diameter d is placed on a table, its perimeter is anchored to the table and a string is attached to its centre. When the string is pulled upwards, a cone is formed with height h and volume V. The relationship between d, h, and V is represented above. Which of the following statements must be true ?
I. As the volume of the cone decreases, the height also decreases.
II. If the diameter of the base of the cone is 6 centimeters, the height can be determined by dividing the volume by 3π.
III. If the height of the conc triples, the volume must also triple.AI onlyBI and II onlyCII and III onlyDI, II, and III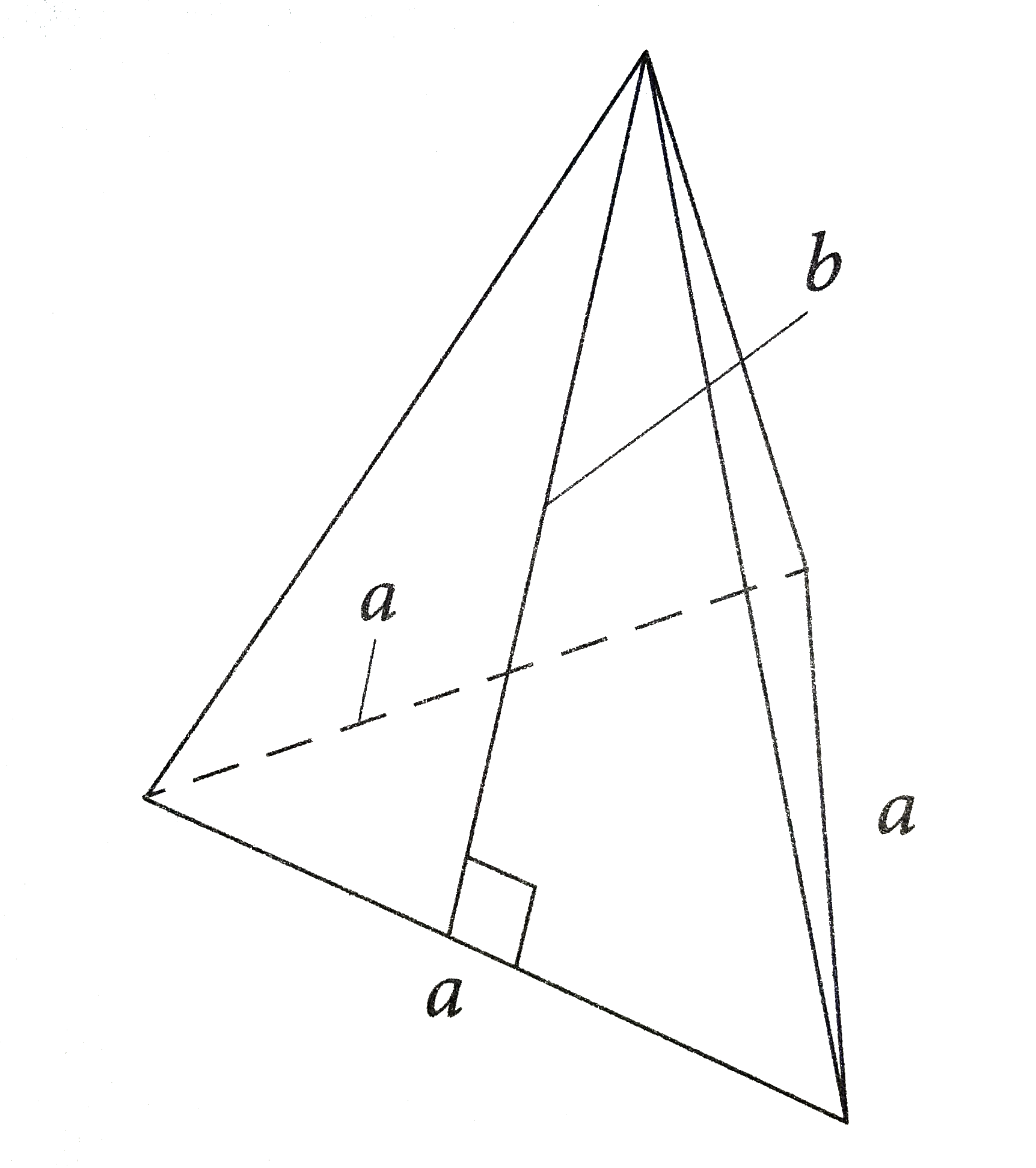
The formula above can be used to find the surface area of the right pyramid with equilateral triangular base shown, where a is the length of each side of the triangle base and b is the slant height of the lateral face. What must the expression 1.5ab represent?AThe area of the baseBThe area of a lateral faceCThe area of the base and one lateral faceDThe sum of the areas of the lateral faces
For the cuboid show in Figure. What is the base of this cuboid? What are the lateral faces of this cuboid? Name one pair of opposite faces. How many pairs of opposite faces are there? Name them. Name all the faces of this cuboid which have X as a vertex.
View SolutionA liquid of density ρ is filled in a beaker of cross section S to a height H and then a cylinder of mass m and corss section s is made to float in it as shown in figure. It the atmospheric pressure is p0, find the pressure a at the top face A of the cylinder b at the botom face C of the cylinder and c. at the base B of the beaker. Can ever these three pressures be equal?

View SolutionA wooden bookshelf has external dimensions as follows: Height=110cm,Depth=25cm,Breadth=85cm (See Figure). The thickness of the plank is 5cm everywhere. The external faces are to be polished and the inner face are to be painted. If the rate of polishing is 20paisepercm2 and if the rate of painting is 10paisepercm2. Find total cost of expenses.
View SolutionAn isosceles triangle of wood of base 2a and height h is placed with its base on the ground vertex directly above. The triangle faces the sun whose altitude is 30∘. Then, the tangent of the angle at the apex of the shadow is
View SolutionThe electric field →E1 at one face of a parallelopiped is uniform over the entire face and is directed out of the face. At the opposite face, the electric field →E2 is also uniform over the entire face and is directed into that face (as shown in figure). The two faces in question are inclined at 30∘ from the horizontal, →E1and→E2(both horizontal) have magnitudes of 2.50×104NC−1 and 7.00×104NC−1. respectively. Assuming that no other electric field lines cross the surfacesof the parallelopiped, the net charge contained with in is
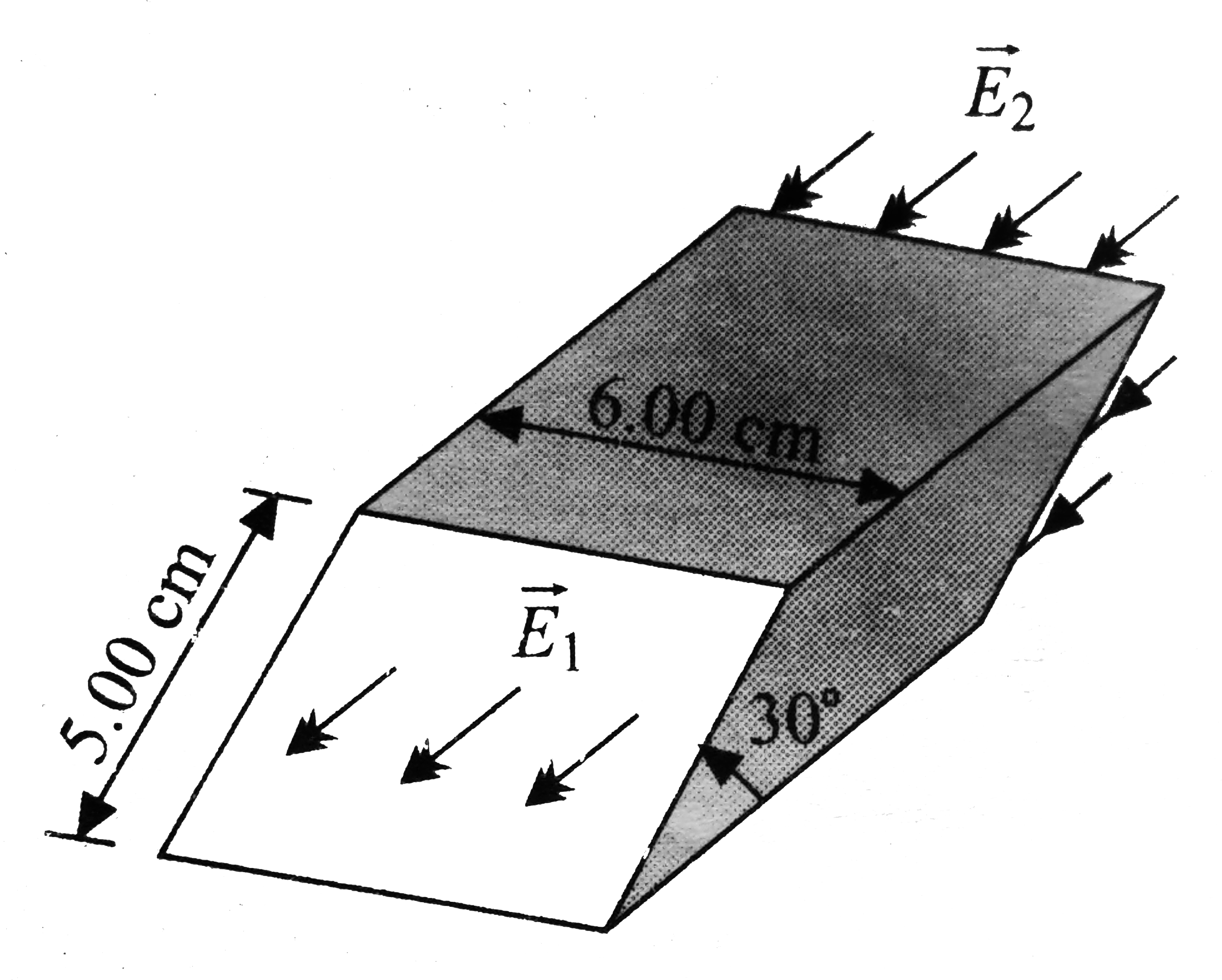
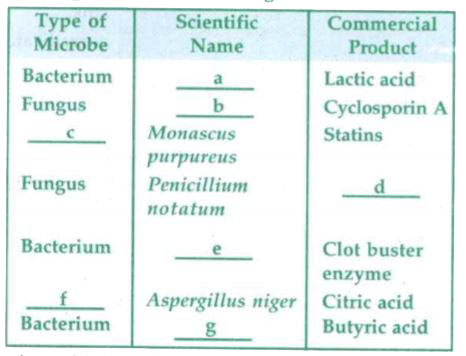
View SolutionName the blank spaces a, b, c, d, e, f and g given in the following table:
View Solution
