In Fig.105, If AOB and COD are straight lines. Then x+y= (a) 120 (b) 140 (c) 100 (d) 160
To solve the problem, we need to find the sum of angles x and y given that lines AOB and COD are straight lines.
1. Understanding the Straight Line Property:
Since AOB and COD are straight lines, the sum of the angles on a straight line is 180∘.
2. Setting Up the Equation for Line AOB:
For line AOB:
7x−20+3x=180
Here, 7x−20 and 3x are the angles formed on line AOB.
3. Combining Like Terms:
Combine the x terms:
10x−20=180
4. Isolating x:
Add 20 to both sides:
10x=180+20
10x=200
5. Solving for x:
Divide both sides by 10:
x=20010=20
6. Setting Up the Equation for Line COD:
For line COD:
y+3x=180
Substitute x=20:
y+3(20)=180
y+60=180
7. Isolating y:
Subtract 60 from both sides:
y=180−60
y=120
8. Finding x+y:
Now, we can find x+y:
x+y=20+120=140
Conclusion:
Thus, the value of x+y is 140.
Answer:
(b) 140
Since AOB and COD are straight lines, the sum of the angles on a straight line is 180∘.
For line AOB:
7x−20+3x=180
Here, 7x−20 and 3x are the angles formed on line AOB.
Combine the x terms:
10x−20=180
Add 20 to both sides:
10x=180+20
10x=200
Divide both sides by 10:
x=20010=20
For line COD:
y+3x=180
Substitute x=20:
y+3(20)=180
y+60=180
Subtract 60 from both sides:
y=180−60
y=120
Now, we can find x+y:
x+y=20+120=140
Thus, the value of x+y is 140.
(b) 140
Topper's Solved these Questions
Similar Questions
In Figure, If AOB and COD are straight lines,
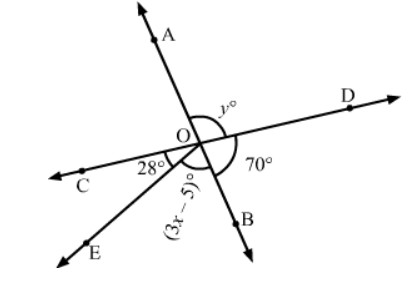
then
View SolutionIn Fig.95, If AB, CD and EF are straight lines, then x= (a) 5 (b) 10 (c) 20 (d) 30
View SolutionIn Fig.96, If AB, CD and EF are straight lines, then x+y+z= (a) 180 (b) 203 (c) 213 (v) 134
View SolutionIn Fig. 91, AOB is a straight line and 4x=5y. The value of x is (a) 100 (b) 105 (c) 110 (d) 115
View SolutionIf 15−16=4x, then x=
(a)−120 (b) −100 (c) 100 (d) 120
View SolutionIn Fig.94, if ∠AOC is a straight line, then the value of x is (a) 15 (b) 18 (c) 20 (d) 16
View SolutionIn Figure, if AB∣∣CD , then x= (a) 1000 (b) 1050 (c) 1100 (d) 1150
View SolutionAOB is a straight line and OC and OD are the two rays on it. If ∠AOC+∠BOD=85∘, then ∠COD=
View SolutionIn Fig.90, AOB is a straight line and the ray OC stands on it. The value of x is (a) 16 (b) 26 (c) 36 (d) 46
View SolutionIn Fig.92, AOB is a straight line such that ∠AOC=(3x+10)0, ∠COD=500 and ∠BOD=(x−8)0. The value of x is (a) 32 (b) 36 (c) 42 (d) 52
View Solution
