In Fig.7, a=b=c, name the angle which is congruent to∠AOC
To solve the problem, we need to find the angle that is congruent to angle AOC given that angles a, b, and c are equal.
1. Understand the Given Information:
We are given that a=b=c. This means that angles AOB, BOC, and COD are all equal.
2. Express the Angles:
Since a=b=c, we can denote each angle as x. Therefore:
∠AOB=x,∠BOC=x,∠COD=x
3. Relate the Angles:
We know that:
∠AOC=∠AOB+∠BOC
Substituting the values, we have:
∠AOC=x+x=2x
4. Find the Other Angle:
Similarly, we can express angle BOD:
∠BOD=∠BOC+∠COD=x+x=2x
5. Establish Congruence:
Since both angles AOC and BOD are equal:
∠AOC=∠BOD
This means that:
∠BOD is congruent to ∠AOC
6. Conclusion:
Therefore, the angle that is congruent to angle AOC is:
∠BOD
Final Answer:
The angle that is congruent to angle AOC is ∠BOD.
We are given that a=b=c. This means that angles AOB, BOC, and COD are all equal.
Since a=b=c, we can denote each angle as x. Therefore:
∠AOB=x,∠BOC=x,∠COD=x
We know that:
∠AOC=∠AOB+∠BOC
Substituting the values, we have:
∠AOC=x+x=2x
Similarly, we can express angle BOD:
∠BOD=∠BOC+∠COD=x+x=2x
Since both angles AOC and BOD are equal:
∠AOC=∠BOD
This means that:
∠BOD is congruent to ∠AOC
Therefore, the angle that is congruent to angle AOC is:
∠BOD
The angle that is congruent to angle AOC is ∠BOD.
Topper's Solved these Questions
Similar Questions
In Fig,8, ∠AOC≅∠PYR and ∠BOC≅∠QYR. Name the angle which is congruent to∠AOB.
View SolutionIn fig.25,two of the angles are indicated what is the measure of ∠ACD?
View SolutionIn Fig. 10.36, A,B and C are three points on a circle with centre O such that ∠BOC= 30o and ∠AOB= 60o. If D is a point on the circle other than the arc ABC, find ∠ADC.
View SolutionIn Fig. 15.98, ABC is a right angled triangle in which ∠A=90o , AB=21 cm and AC=28 cm . Semi-circles are described on AB, BC and AC as diameters. Find the area of the shaded region. (FIGURE)
View SolutionIn Figure, it is given that O is the centre of the circle and ∠AOC=150∘. Find ∠ABC
View SolutionIf any two angles and a non-included side of one triangle are equal to the corresponding angles and side of another triangle, then the two triangles are congruent. GIVEN : Two sABC and DEF such that ∠A=∠D,∠B=∠E,BC=EF TO PROVE : ABC≅DEF Figure
View SolutionIn Figure, O is the centre of the circle such that ∠AOC =1300, then ∠ABC= (a)1300 (b) 1150 (c) 650 (d) 1650
View SolutionIn Fig., which of the following statements are true: (i) Point B is the interior of ∠AOB (ii) Point B is the interior of ∠AOC (iii)Point A is the interior of ∠AOD (iv)point C is the exterior of ∠AOB (v)Point D is the exterior of ∠AOC.
View SolutionIn Fig. 6.13, lines AB and CD intersect at O. If ∠AOC +∠BOE=70∘and ∠BOD=40∘, find ∠BOE and reflex ∠COE.
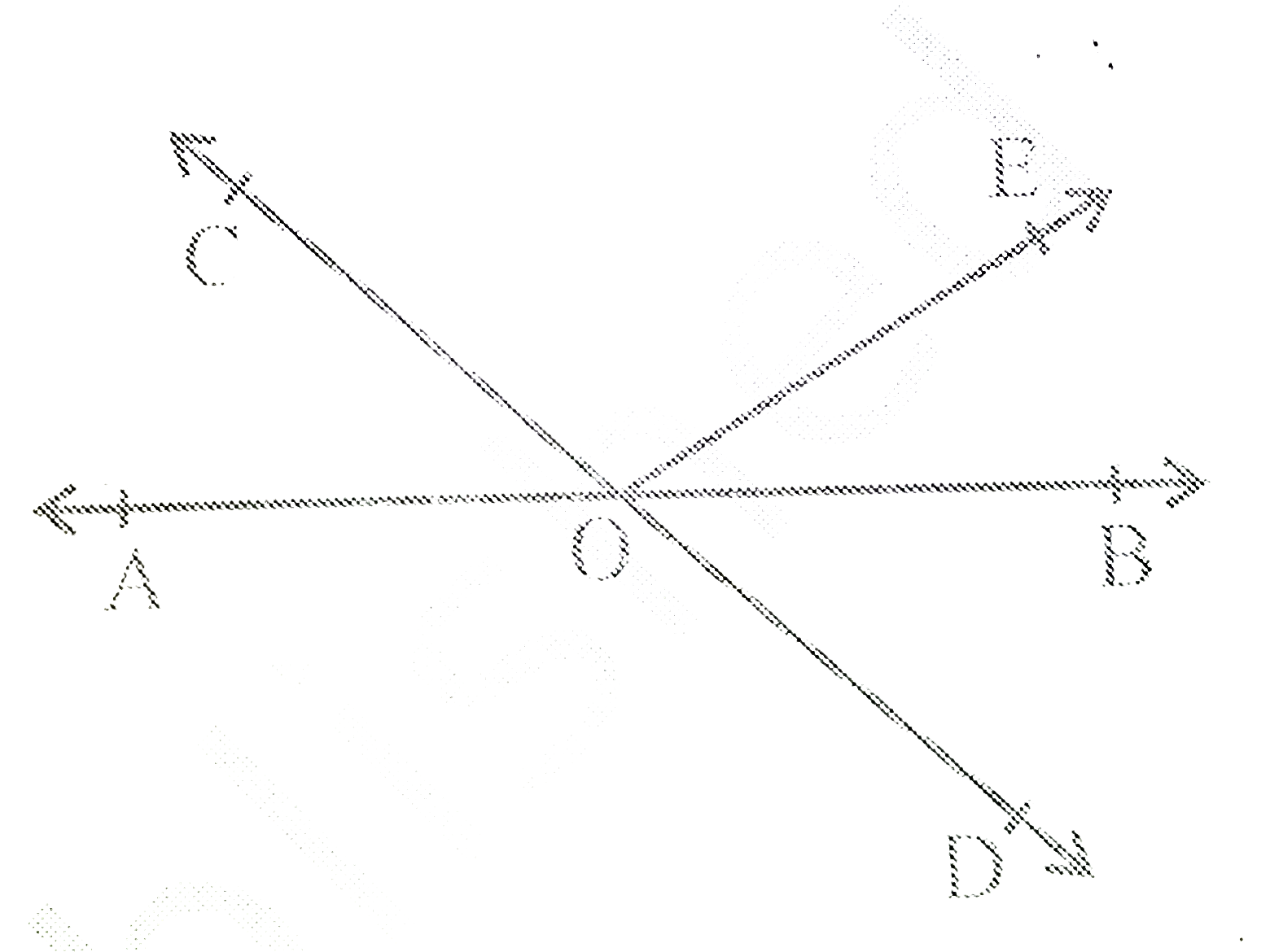
View SolutionIn Figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD=146∘, find ∠AOB
View Solution
