If the mean of is , find the value of
To solve the problem step by step, we need to find the value of x given that the mean of the numbers 6, 8, 5, x, and 4 is 7.
1. Understand the Mean Formula:
The mean (average) of a set of numbers is calculated using the formula:
Mean=Sum of ObservationsNumber of Observations
2. Identify the Observations:
The observations given are 6, 8, 5, x, and 4.
3. Count the Number of Observations:
There are a total of 5 observations (6, 8, 5, x, and 4).
4. Set Up the Equation Using the Mean:
According to the problem, the mean of these observations is 7. Therefore, we can set up the equation:
7=6+8+5+x+45
5. Multiply Both Sides by 5:
To eliminate the fraction, multiply both sides of the equation by 5:
7×5=6+8+5+x+4
This simplifies to:
35=6+8+5+x+4
6. Calculate the Sum of the Known Observations:
Now, add the known numbers:
6+8=14
14+5=19
19+4=23
So, we have:
35=23+x
7. Isolate x:
To find x, subtract 23 from both sides:
x=35−23
x=12
8. Final Answer:
The value of x is 12.
The mean (average) of a set of numbers is calculated using the formula:
Mean=Sum of ObservationsNumber of Observations
The observations given are 6, 8, 5, x, and 4.
There are a total of 5 observations (6, 8, 5, x, and 4).
According to the problem, the mean of these observations is 7. Therefore, we can set up the equation:
7=6+8+5+x+45
To eliminate the fraction, multiply both sides of the equation by 5:
7×5=6+8+5+x+4
This simplifies to:
35=6+8+5+x+4
Now, add the known numbers:
6+8=14
14+5=19
19+4=23
So, we have:
35=23+x
To find x, subtract 23 from both sides:
x=35−23
x=12
The value of x is 12.
Topper's Solved these Questions
Similar Questions
n' arithmetic means are there between 4 and 36. If the ratio of 3rd and (n-2)th mean is 2:3, find the value of n.
View SolutionThere are 'n' A.M.'s between 2 and 41. The ratio of 4th and (n - 1)th mean is 2 : 5, find the value of n.
View SolutionIf the mean deviation of the numbers 1,1+d, 1+2d, 1+3d,…1+100d from their mean is 255, then find the value of d
View SolutionFind the mean of
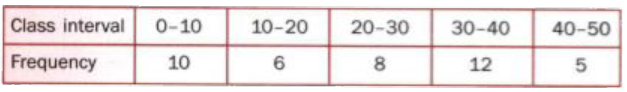
View SolutionThe total number of observations in the following distribution table is 120 and their mean is 50. Find the value of missing frequencies f1andf2 :

View SolutionThe total number of observations in the following distribution tale is 120 and their mean is 50. Find the values of missing frequencies f1 and f2
View SolutionThere are n A.M.s between 3 and 17. The ratio of the last mean to the first mean is 3:1. Find the value of n.
View SolutionThere are n A.M.s between 3 and 17. The ratio of the last mean to the first mean is 3:1. Find the value of n.
View SolutionIf n arithemetic means are inserted between 20 and 80 such that the ratio of first mean to the last mean is 1:3, then find the value of n.
View SolutionThere are m arithmetic means between 5 and -16 such that the ratio of the 7th mean to the (m-7)th mean is 1:4. Find the value of m.
View Solution
