Find the solution for following cryptarithms:
ABx3=CAB
To solve the cryptarithm AB×3=CAB, we will break down the problem step by step.
Step 1: Understand the structure of the numbers
The two-digit number AB can be expressed as 10A+B, where A is the tens digit and B is the units digit. The three-digit number CAB can be expressed as 100C+10A+B.
Step 2: Set up the equation
From the problem statement, we have:
(10A+B)×3=100C+10A+B
Step 3: Expand and simplify the equation
Expanding the left side gives:
30A+3B=100C+10A+B
Now, rearranging the equation:
30A+3B−10A−B=100C
This simplifies to:
20A+2B=100C
Dividing the entire equation by 2:
10A+B=50C
Step 4: Analyze the equation
From the equation 10A+B=50C, we can see that 10A+B must be a multiple of 50. Since A and B are digits (0-9), we can deduce possible values for C.
Step 5: Determine possible values for B
Since B is the unit digit of 3B, we need to find values of B such that 3B ends with the digit B. The only digits that satisfy this condition are:
- B=0 (since 3×0=0)
- B=5 (since 3×5=15, ends with 5)
Step 6: Case 1: B=0
If B=0:
10A+0=50C⟹10A=50C⟹A=5C
Since A must be a digit (0-9), the only possible value for C is 1 (since A must also be a digit):
A=5×1=5andC=1
Thus, we have:
- A=5
- B=0
- C=1
Step 7: Case 2: B=5
If B=5:
10A+5=50C
This leads to:
10A=50C−5⟹10A=5(10C−1)⟹2A=10C−1
For A to be a digit, 10C−1 must be even. However, since 10C−1 is odd for any integer C, this case does not yield valid digits for A.
Conclusion
The only valid solution from our analysis is:
- A=5
- B=0
- C=1
Thus, the solution to the cryptarithm AB×3=CAB is:
A=5,B=0,C=1
The two-digit number AB can be expressed as 10A+B, where A is the tens digit and B is the units digit. The three-digit number CAB can be expressed as 100C+10A+B.
From the problem statement, we have:
(10A+B)×3=100C+10A+B
Expanding the left side gives:
30A+3B=100C+10A+B
Now, rearranging the equation:
30A+3B−10A−B=100C
This simplifies to:
20A+2B=100C
Dividing the entire equation by 2:
10A+B=50C
From the equation 10A+B=50C, we can see that 10A+B must be a multiple of 50. Since A and B are digits (0-9), we can deduce possible values for C.
Since B is the unit digit of 3B, we need to find values of B such that 3B ends with the digit B. The only digits that satisfy this condition are:
- B=0 (since 3×0=0)
- B=5 (since 3×5=15, ends with 5)
If B=0:
10A+0=50C⟹10A=50C⟹A=5C
Since A must be a digit (0-9), the only possible value for C is 1 (since A must also be a digit):
A=5×1=5andC=1
Thus, we have:
- A=5
- B=0
- C=1
If B=5:
10A+5=50C
This leads to:
10A=50C−5⟹10A=5(10C−1)⟹2A=10C−1
For A to be a digit, 10C−1 must be even. However, since 10C−1 is odd for any integer C, this case does not yield valid digits for A.
The only valid solution from our analysis is:
- A=5
- B=0
- C=1
A=5,B=0,C=1
Topper's Solved these Questions
Similar Questions
Solve the following cryptarithms: ABx6=BBB
View SolutionSolve the following cryptarithms :
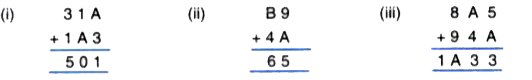
View SolutionSolve the following cryptarithms: 37+AB=9A
View SolutionSolve the cryptarithm: AB X AB= ACB
View SolutionSolve each of the following Cryptarithms: (i) A1+1B=B0
View SolutionSolve the cryptarithm: BAXB3=57A
View SolutionSolve the cryptarithm : AB+BA= DAD
View SolutionFind the sum of the following algebraic expressions :
3a−4b+5c,2a+3b−6c,−5a+8b+7c
View SolutionFind the values of a and b for which the following system of linear equations has infinite number of solutions: 2x−3y=7, (a+b)x−(a+b−3)y=4a+b
View SolutionFind the values of a and b for which the following system of equations has infinitely many solutions: 2x+3y=7, (a−b)x+(a+b)y=3a+b−2
View Solution
